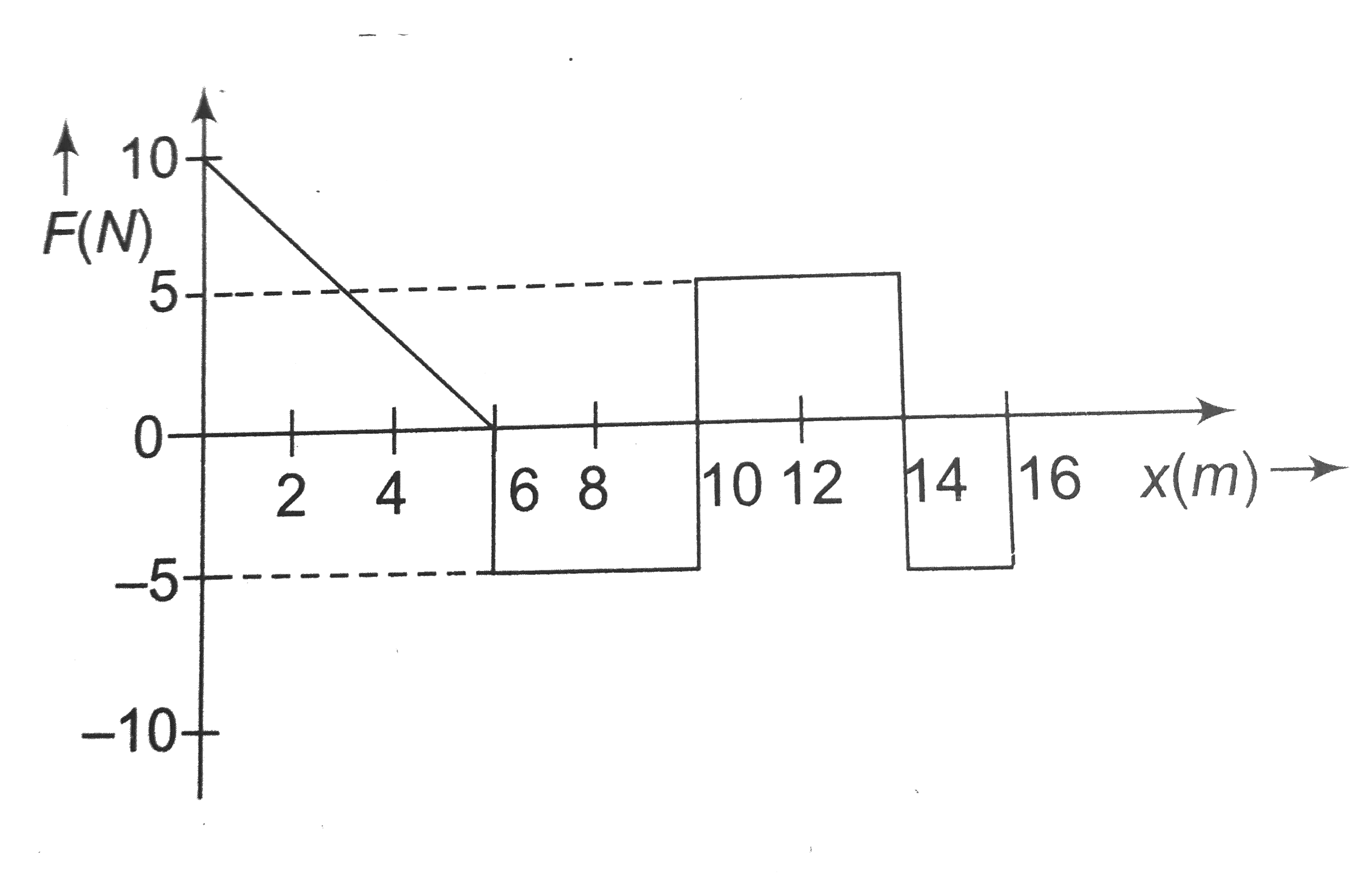
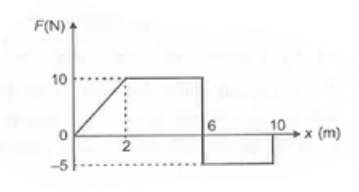
A particle is acted upon by a force F which varies with position x is shown in figure .If the particle at x=0 kinetic energy of 25J then the kinetic energy of the particle at x=16m is
45 J
30 J
70 J
20 J
The correct Answer is:A
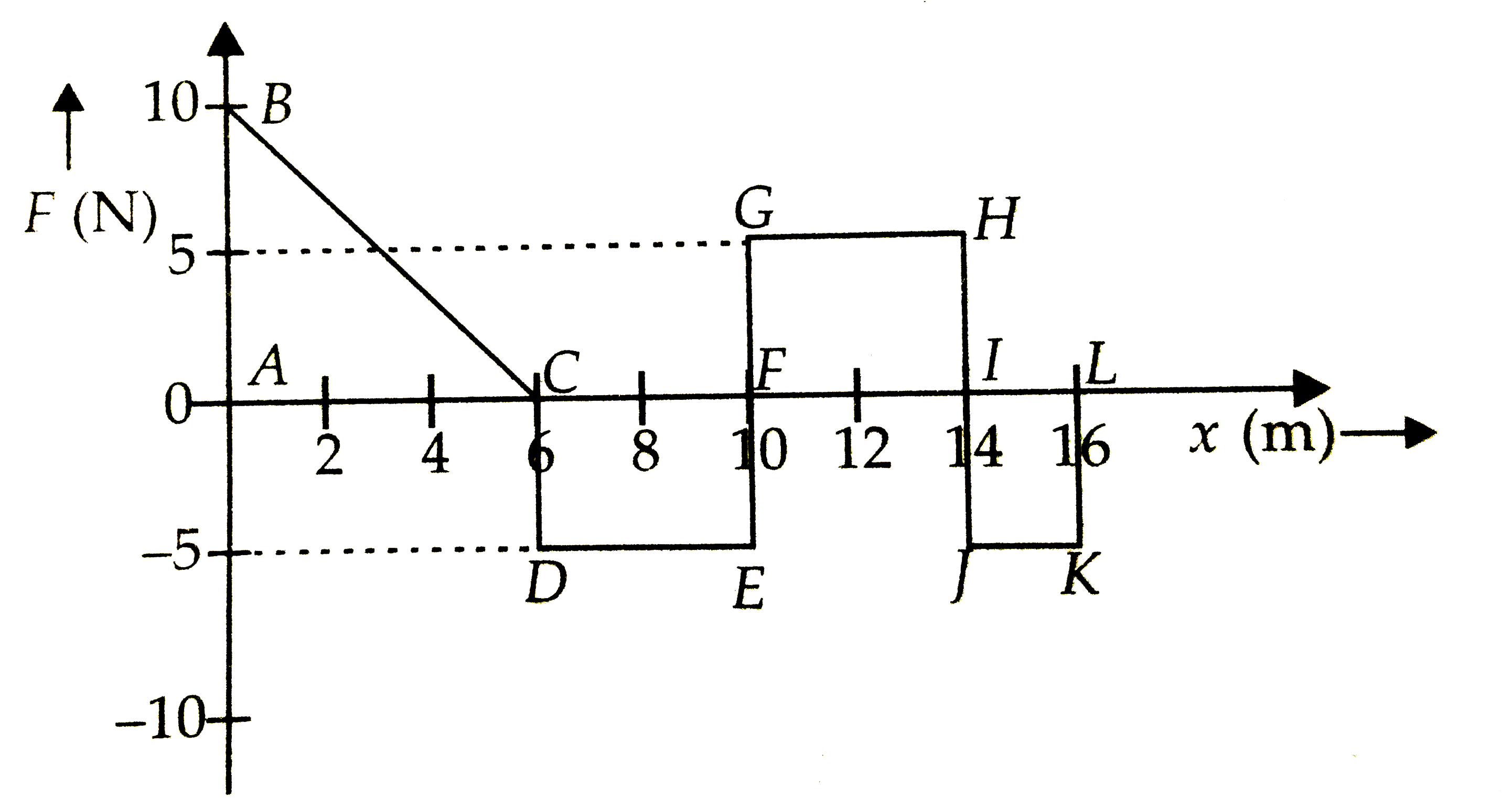
Work done W = Area under F-x graph with proper sign W = Area of triangle ABC+Area of rectangle CDEF+Area of rectangle FGHI+Area of rectangle IJKL
W=[12×6×10]+[4×(−5)]+[4×(5)]+[2×(−5)]
=30-20+20-10= 20 J...(i)
According to work energy theorem
Kf−Ki=W
or (Kf)x=16m−(Ki)x=0m=W
(Kf)x=16m=(Ki)x=0m+W=25 J + 20 J =45 J
Topper's Solved these Questions
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - NCERT
18 Videos
- WORK , ENERGY AND POWERBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WORK , ENERGY AND POWEREXERCISE - ASSERTION & REASON
15 Videos
- WAVESBOOK - NCERT FINGERTPS ENGLISHCHAPTER - WAVESEXERCISE - Assertion And Reason
15 Videos
Similar Questions
Cooling decreases the kinetic energy of particles.
View SolutionThe acceleration of a particle which moves along the positive x-axis varies with its position as shown in figure. If the velocity of the particle is 0.8ms−1 at x = 0, then velocity of the particle at x=1.4m is (∈ms−1).
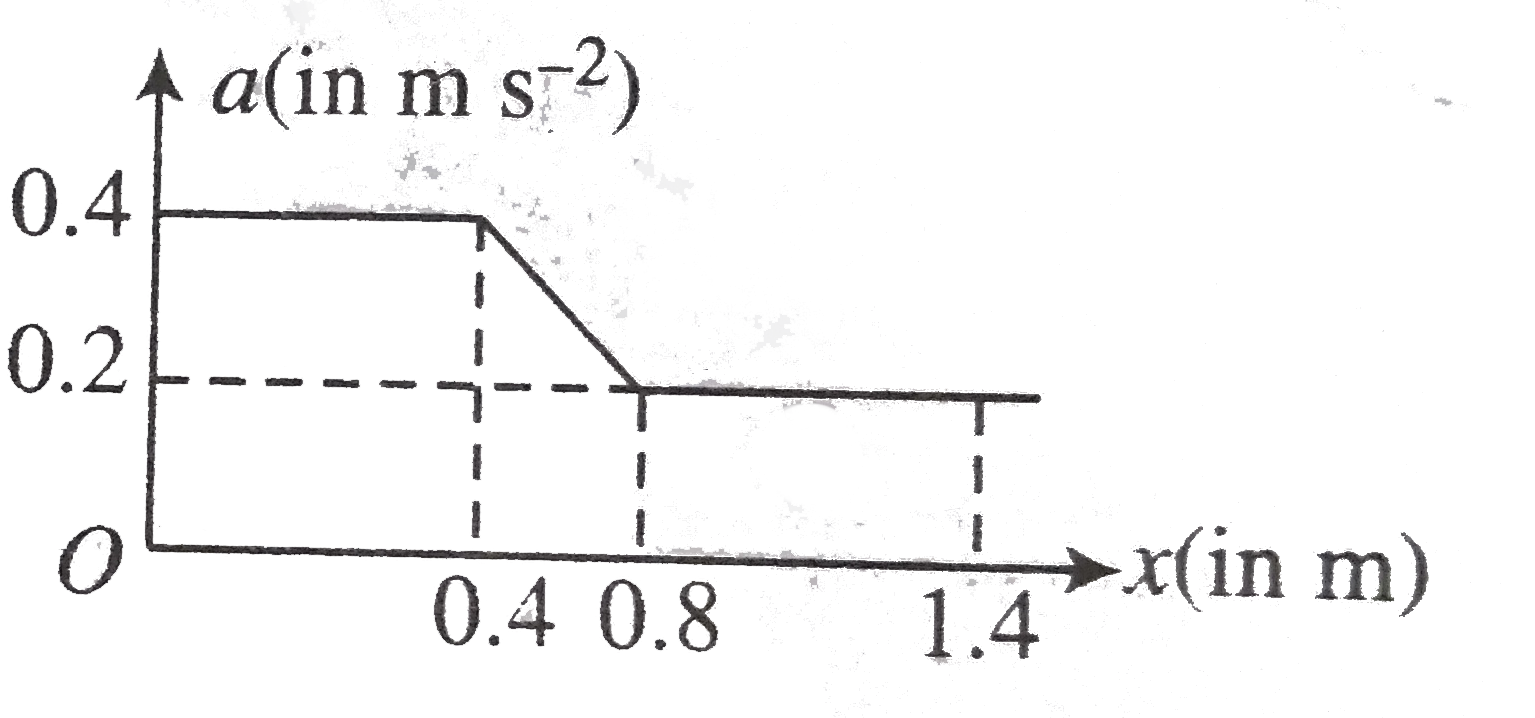
View SolutionWhen a particle executes SHM oscillates with a frequency v, then the kinetic energy of the particle
View SolutionSolute particles __________ the kinetic energy of the solvent.
View SolutionA particle is projected at an angle θ from the horizontal with kinetic energy (T). What is the kinetic energy of the particle at the highest point ?
View SolutionA force F acting on a particle varies with the position x as shown in figure. Find the work done by this force in displacing the particle from

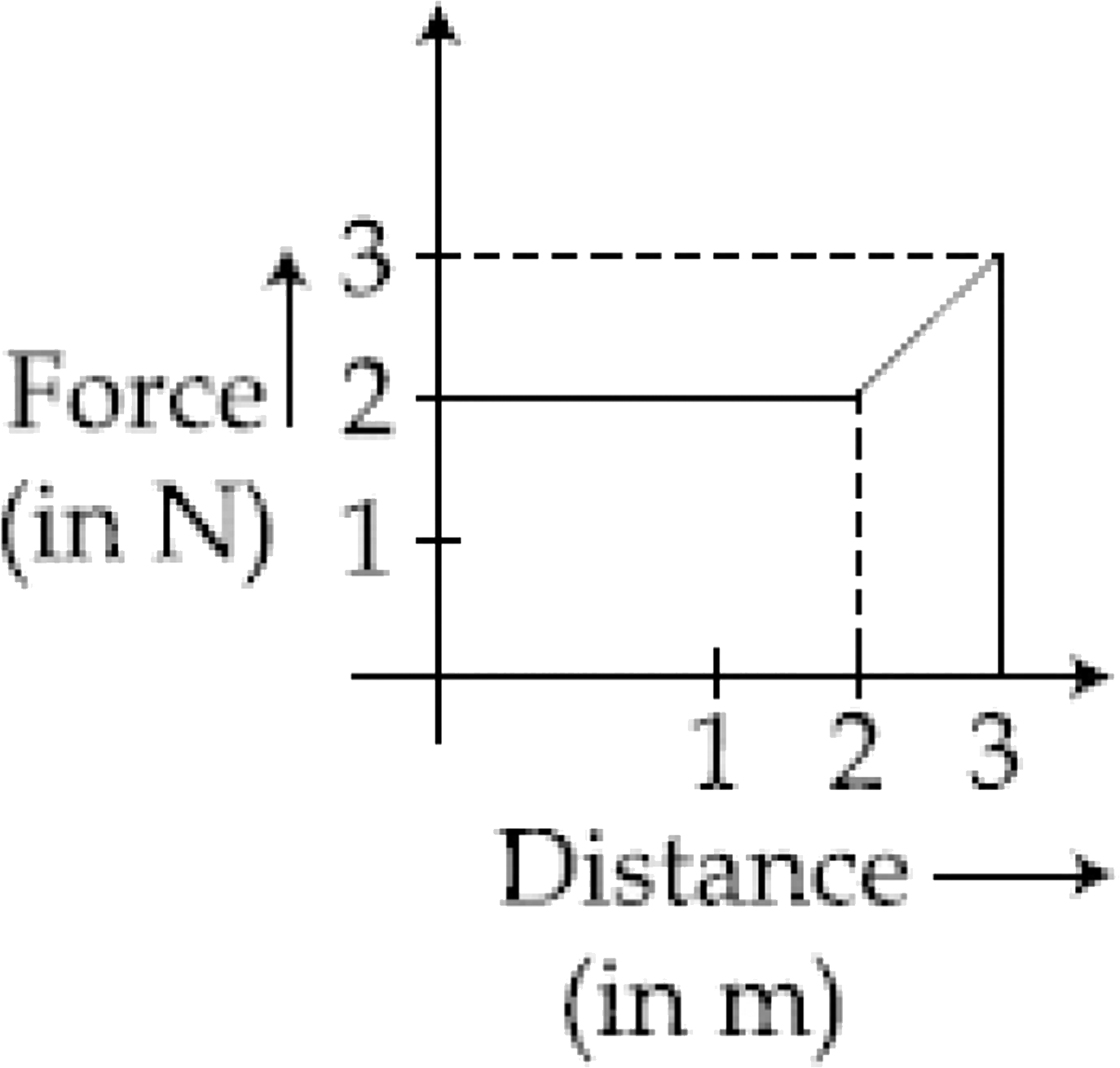
View SolutionA particle moves in one dimension from rest under the influence of a force that varies with the distance travelled by the particle as shown in the figure. The kinetic energy of the particle after it has travelled 3m is:
View SolutionThe variation of force (F) acting on a particle of mass 800 g with position (x) is as shown in figure. The time period of oscillation of the particles is
View SolutionA force (F) acting on a particle varies work done by a particle varies with the with the position x as shown in figure
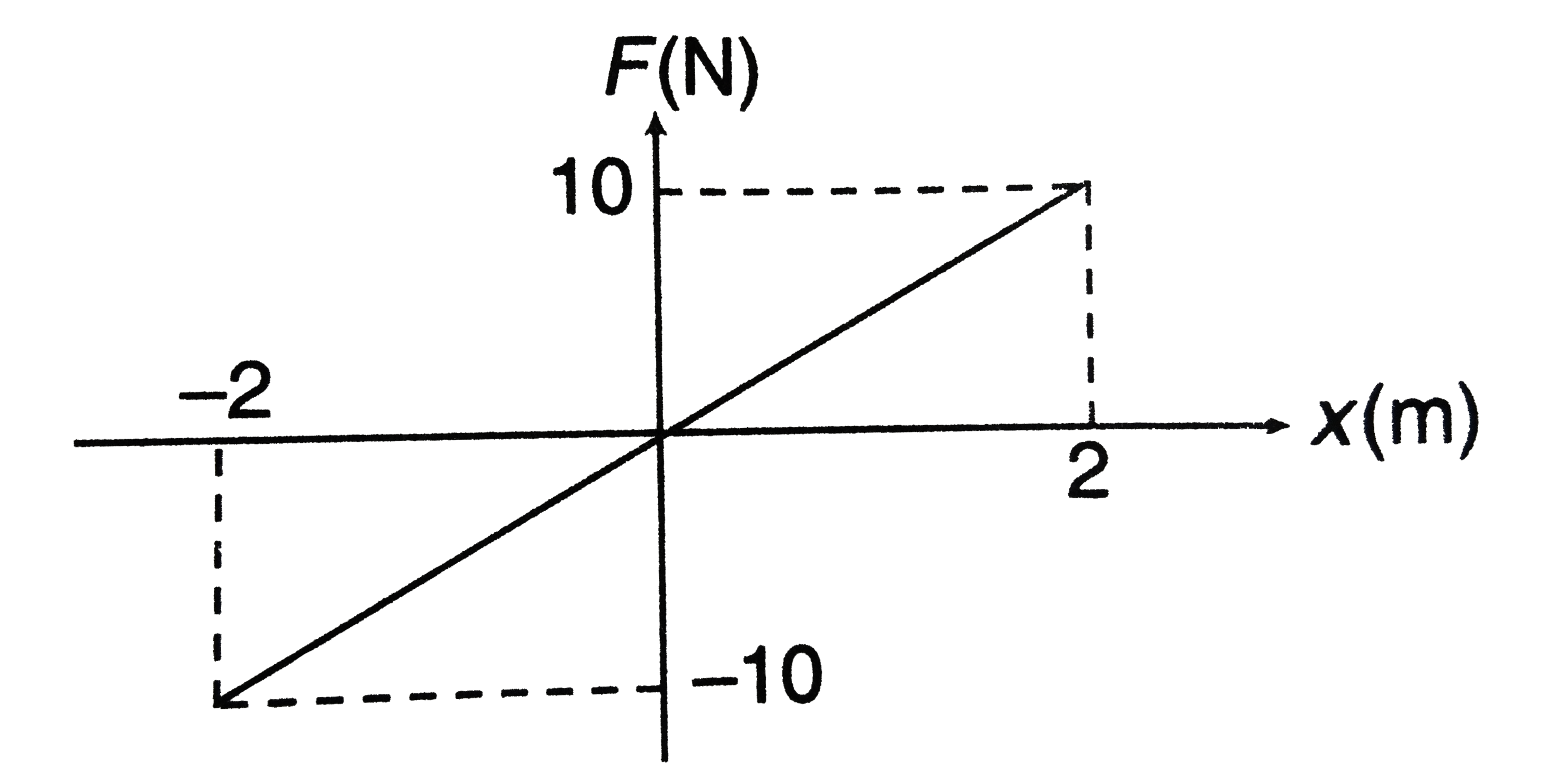 .
.
Find the work done by by force in displacing the particle from .
(a) x=−2m to x=0
(b) x=0 to x=2m..
View SolutionThe force versus position (F-x) graph of a particle of mass 2 kg is shown in the figure. If at x = 0 velocity of particle is 2 m/s then velocity of the particle at x = 8 m will be
View Solution