A particle is projected from a tower of height 25 m with velocity 20√2m/s at 45∘. (a) Find the time when particle strikes with ground. (b) The horizontal distance from the foot of tower where it strikes. (c) Also find the velocity at the time of collision.
The correct Answer is:A, B, C, D
To solve the problem step by step, we will break it down into three parts as stated in the question:
Given Data:
- Height of the tower (h) = 25 m
- Initial velocity (u) = 20√2 m/s
- Angle of projection (θ) = 45°
- Acceleration due to gravity (g) = 9.8 m/s² (acting downwards)
Step 1: Find the time when the particle strikes the ground.
1. Resolve the initial velocity into horizontal and vertical components:
- ux=u⋅cos(θ)=20√2⋅cos(45°)=20√2⋅1√2=20m/s
- uy=u⋅sin(θ)=20√2⋅sin(45°)=20√2⋅1√2=20m/s
2. Use the second equation of motion for vertical displacement:
The vertical displacement y is given by:
y=uyt−12gt2
Here, y=−25 m (downward displacement), uy=20 m/s, and g=9.8 m/s².
−25=20t−12⋅9.8t2
Rearranging gives:
4.9t2−20t−25=0
3. Solve the quadratic equation using the quadratic formula:
t=−b±√b2−4ac2a
Here, a=4.9, b=−20, and c=−25.
t=20±√(−20)2−4⋅4.9⋅(−25)2⋅4.9
t=20±√400+4909.8
t=20±√8909.8
t=20±29.839.8
Taking the positive root:
t=49.839.8≈5.08s
Step 2: Find the horizontal distance from the foot of the tower where it strikes.
1. Calculate the horizontal distance (d):
d=ux⋅t
d=20⋅5.08≈101.6m
Step 3: Find the velocity at the time of collision.
1. Calculate the vertical component of the velocity just before impact (v_y):
Using the equation:
v2y=u2y+2gh
v2y=202+2⋅9.8⋅25
v2y=400+490=890
vy=√890≈29.83m/s
2. The horizontal component of the velocity remains constant:
vx=20m/s
3. Calculate the resultant velocity (v):
v=√v2x+v2y
v=√202+29.832=√400+890=√1290≈35.91m/s
4. Find the direction of the velocity:
tan(θ)=vyvx=29.8320
θ=tan−1(29.8320)≈33.84∘
Final Answers:
(a) Time when the particle strikes the ground: 5.08 s
(b) Horizontal distance from the foot of the tower: 101.6 m
(c) Velocity at the time of collision: 35.91 m/s at an angle of 33.84° to the horizontal.
- Height of the tower (h) = 25 m
- Initial velocity (u) = 20√2 m/s
- Angle of projection (θ) = 45°
- Acceleration due to gravity (g) = 9.8 m/s² (acting downwards)
- ux=u⋅cos(θ)=20√2⋅cos(45°)=20√2⋅1√2=20m/s
- uy=u⋅sin(θ)=20√2⋅sin(45°)=20√2⋅1√2=20m/s
The vertical displacement y is given by:
y=uyt−12gt2
Here, y=−25 m (downward displacement), uy=20 m/s, and g=9.8 m/s².
−25=20t−12⋅9.8t2
Rearranging gives:
4.9t2−20t−25=0
t=−b±√b2−4ac2a
Here, a=4.9, b=−20, and c=−25.
t=20±√(−20)2−4⋅4.9⋅(−25)2⋅4.9
t=20±√400+4909.8
t=20±√8909.8
t=20±29.839.8
Taking the positive root:
t=49.839.8≈5.08s
d=ux⋅t
d=20⋅5.08≈101.6m
Using the equation:
v2y=u2y+2gh
v2y=202+2⋅9.8⋅25
v2y=400+490=890
vy=√890≈29.83m/s
vx=20m/s
v=√v2x+v2y
v=√202+29.832=√400+890=√1290≈35.91m/s
tan(θ)=vyvx=29.8320
θ=tan−1(29.8320)≈33.84∘
(a) Time when the particle strikes the ground: 5.08 s
(b) Horizontal distance from the foot of the tower: 101.6 m
(c) Velocity at the time of collision: 35.91 m/s at an angle of 33.84° to the horizontal.
Topper's Solved these Questions
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Exercise 7.2
10 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Exercise 7.3
6 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Miscellaneous Examples
4 Videos
- MOTION IN A PLANEBOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery
32 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer
8 Videos
Similar Questions
A particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
View SolutionA particle is projected from ground with velocity 20(√2)m/s at 45∘. At what time particle is at height 15 m from ground? (g=10m/s2)
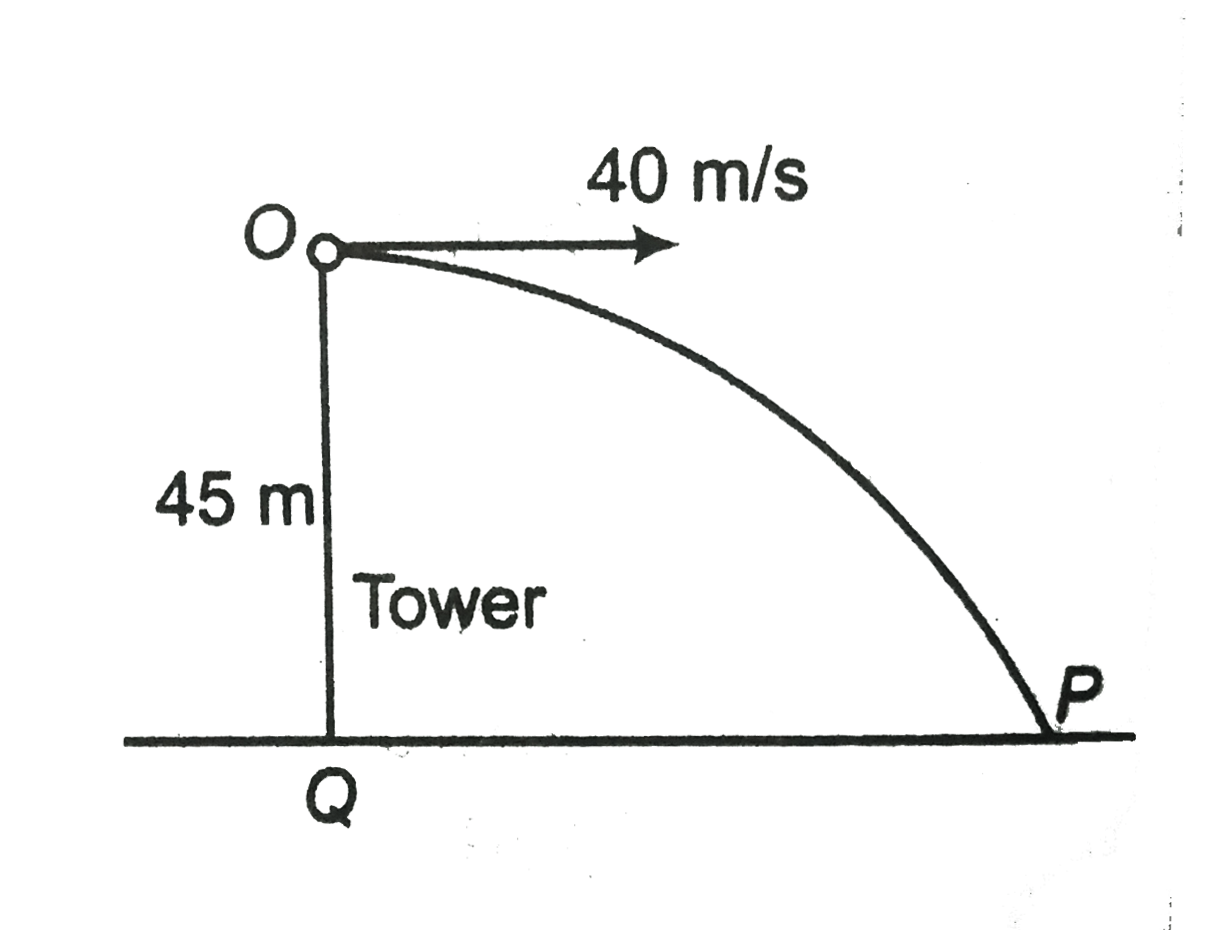
View SolutionIn the figure shown, find
(a) the time when the particle strikes the ground at P.
(b) the horizontal distance QP
(c) velocity of the particle at P.
View SolutionA particle is projected from ground with velocity 40(√2)m/s at 45∘. Find
(a) velocity and
(b) displacement of the particle after 2 s. (g=10m/s2)
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionA ball is thrown upwards from the top of a tower 40m high with a velocity of 10m/s. Find the time when it strikes the ground. Take g=10m/s2.
View SolutionThe particle of mass 1 kg is projected with velocity 20√2m/s at 45∘ with ground . When , the particle is at highest point (g=10m/s2),
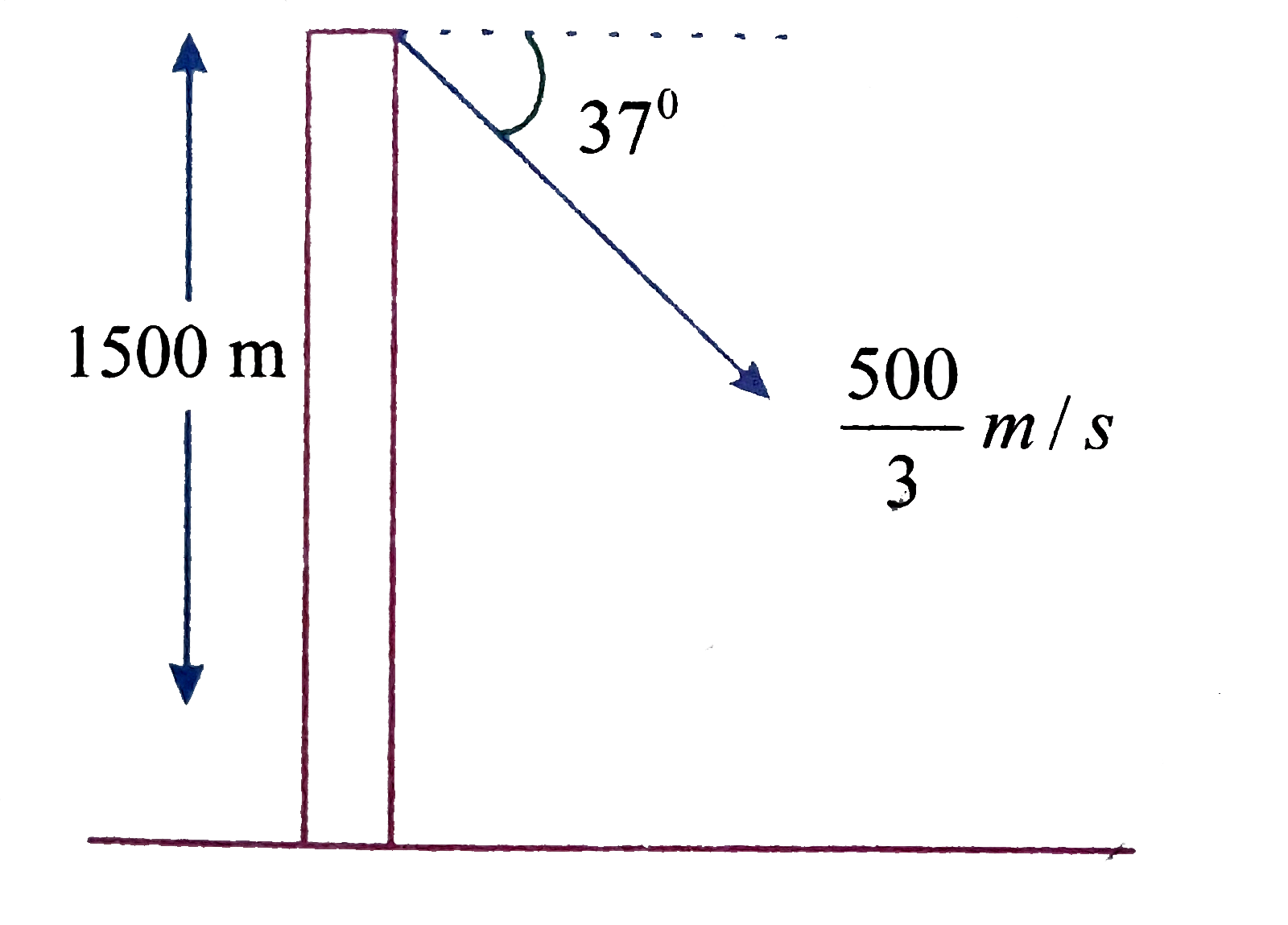
View SolutionA particle is projected from a tower as shown in figure, then find the distance from the foot of the tower where it will strike the ground. (g=10m/s2)
View SolutionA particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View SolutionA particle of mass 50 g is projected horizontally from the top of a tower of height 50 m with a velocity 20m/s. If g=10m/s2, then find the :-
View Solution
