A particle is projected from ground with velocity 20(√2)m/s at 45∘. At what time particle is at height 15 m from ground? (g=10m/s2)
The correct Answer is:A, C, D
To solve the problem of finding the time at which a particle projected from the ground reaches a height of 15 meters, we can follow these steps:
Step 1: Identify the given values
- Initial velocity u=20√2m/s
- Angle of projection θ=45∘
- Height sy=15m
- Acceleration due to gravity g=10m/s2
Step 2: Calculate the vertical component of the initial velocity
The vertical component of the initial velocity uy can be calculated using the formula:
uy=usin(θ)
Substituting the values:
uy=20√2⋅sin(45∘)=20√2⋅1√2=20m/s
Step 3: Use the kinematic equation for vertical motion
The equation for vertical motion is given by:
sy=uyt+12ayt2
Here, ay=−g=−10m/s2 (since gravity acts downwards). Substituting the known values:
15=20t−12⋅10t2
This simplifies to:
15=20t−5t2
Step 4: Rearrange the equation
Rearranging the equation gives:
5t2−20t+15=0
Dividing the entire equation by 5:
t2−4t+3=0
Step 5: Factor the quadratic equation
We can factor the quadratic equation:
(t−1)(t−3)=0
Step 6: Solve for t
Setting each factor to zero gives:
t−1=0⇒t=1s
t−3=0⇒t=3s
Conclusion
The particle reaches a height of 15 meters at two different times: t=1s and t=3s.
---
- Initial velocity u=20√2m/s
- Angle of projection θ=45∘
- Height sy=15m
- Acceleration due to gravity g=10m/s2
The vertical component of the initial velocity uy can be calculated using the formula:
uy=usin(θ)
Substituting the values:
uy=20√2⋅sin(45∘)=20√2⋅1√2=20m/s
The equation for vertical motion is given by:
sy=uyt+12ayt2
Here, ay=−g=−10m/s2 (since gravity acts downwards). Substituting the known values:
15=20t−12⋅10t2
This simplifies to:
15=20t−5t2
Rearranging the equation gives:
5t2−20t+15=0
Dividing the entire equation by 5:
t2−4t+3=0
We can factor the quadratic equation:
(t−1)(t−3)=0
Setting each factor to zero gives:
t−1=0⇒t=1s
t−3=0⇒t=3s
The particle reaches a height of 15 meters at two different times: t=1s and t=3s.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Single Correct10videos
PROJECTILE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 More Than One Correct8videos
PROJECTILE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 1 Single Correct16videos
PROJECTILE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - MOTION IN A PLANEEXERCISE - (C )Medical entrances gallery32videos
MOTION IN A PLANE
- BOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - Integer8videos
PROPERTIES OF MATTER
Similar Questions
A particle is projected from ground with velocity 40√2m/s at 45∘. At time t=2s
View SolutionA particle is projected from ground with velocity 3ˆi+4ˆj m/s. Find range of the projectile :-
View SolutionA particle is projected from ground with velocity 40(√2)m/s at 45∘. Find
(a) velocity and
(b) displacement of the particle after 2 s. (g=10m/s2)View SolutionThe particle of mass 1 kg is projected with velocity 20√2m/s at 45∘ with ground . When , the particle is at highest point (g=10m/s2),
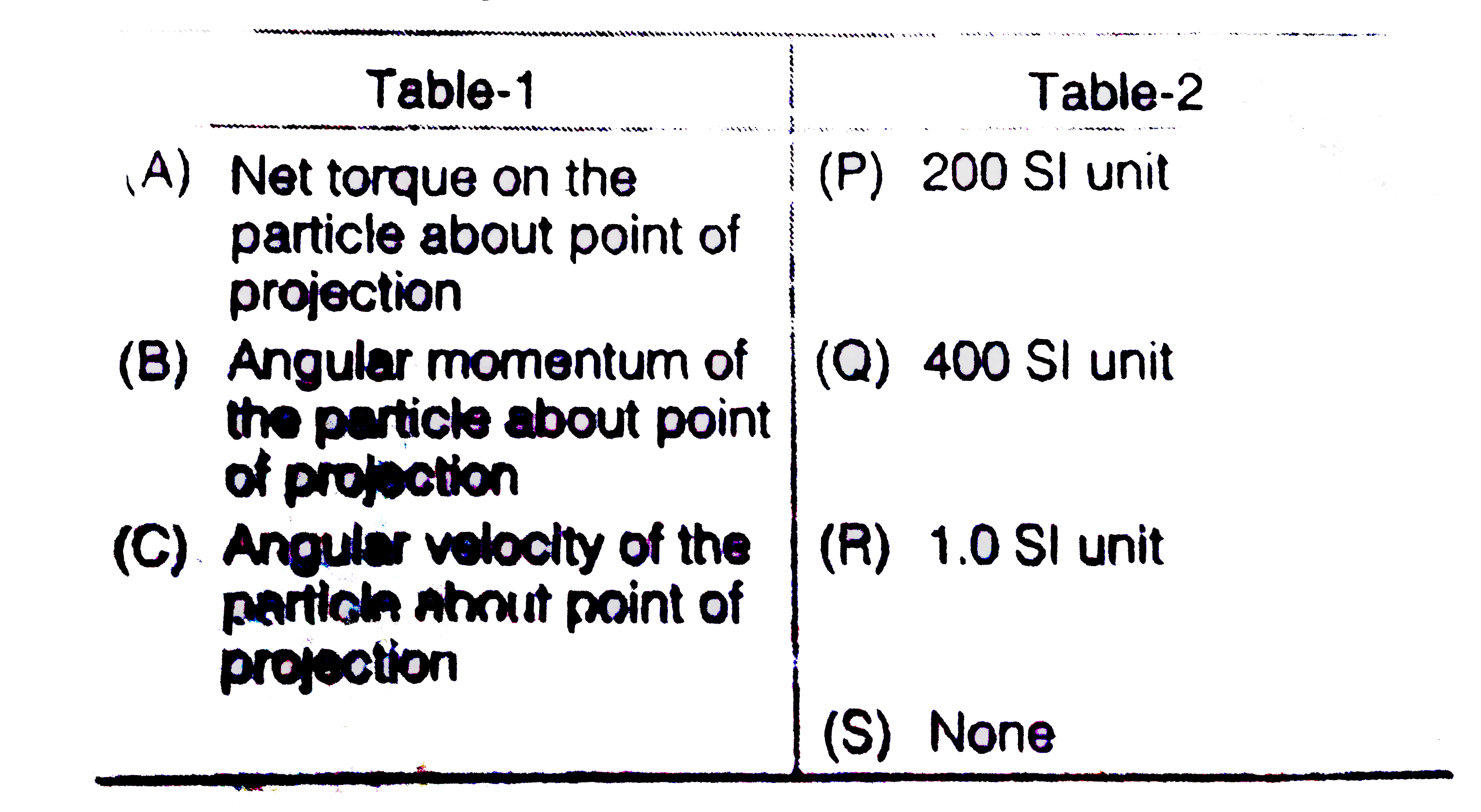 View Solution
View SolutionA particle is projected from a tower of height 25 m with velocity 20√2m/s at 45∘. (a) Find the time when particle strikes with ground. (b) The horizontal distance from the foot of tower where it strikes. (c) Also find the velocity at the time of collision.
View SolutionA projectile of mass 3m is projected from ground with velocity 20√2m/s at 45∘. At highest point it explodes into two pieces. One of mass 2m and the other of mass m. Both the pieces fly off horizontally in opposite directions. Mass 2m falls at a distance of 100m from point of projection. Find the distance of second mass from point of projection where it strikes the ground.
(g=10m/s2)View SolutionA particle is projected from ground with initial velocity u=20(√2)m/s at θ=45∘. Find
(a) R,H and T,
(b) velocity of particle after 1 s
(c) velocity of particle at the time of collision with the ground (x-axis).
 View Solution
View SolutionA particle is projected upwards with velocity 20m/s. Simultaneously another particle is projected with velocity 20(√2)m/s at 45∘. (g=10m/s2)
(a) What is acceleration of first particle relative to the second?
(b) What is initial velocity of first particle relative to the other?
(c) What is distance between two particles after 2 s?View SolutionA particle is projected from ground with velocity 50m/s at 37∘ from horizontal. Find velocity and displacement after 2 s. sin37∘=35.
View SolutionA particle is projected vertically upwards from ground with velocity 10 m/s. Find the time taken by it to reach at the highest point ?
View Solution

