A particle is projected upwards with initial velocity u. assume acceleration due to gravity is 10m/s2. It is found that particle covers 5m in last second before reaching the maximum height. What can be the possible value of u?
20m/s
35m/s
5m/s
40m/s
The correct Answer is:a,b,d
To solve the problem, we need to determine the initial velocity u of a particle projected upwards, given that it covers a distance of 5 m in the last second before reaching its maximum height. The acceleration due to gravity is g=10m/s2.
1. Understanding the Motion:
- The particle is projected upwards with an initial velocity u.
- It moves upwards until it reaches its maximum height, where its velocity becomes zero.
- The distance covered in the last second before reaching the maximum height is given as 5 m.
2. Using the Formula for Distance in the nth Second:
- The distance covered in the nth second can be calculated using the formula:
Sn=u+a2⋅(2n−1)
- Here, Sn is the distance covered in the nth second, u is the initial velocity, a is the acceleration (which is −g or −10m/s2 in this case), and n is the total time taken to reach the maximum height.
3. Setting Up the Equation:
- Since the distance covered in the last second (which is the nth second) is 5 m, we can write:
5=u+−102⋅(2n−1)
- Simplifying this gives:
5=u−5(2n−1)
- Rearranging gives:
5=u−10n+5
- Thus:
u=10n
4. Finding the Time to Reach Maximum Height:
- The time n taken to reach the maximum height can also be found using the equation:
v=u+at
- At maximum height, the final velocity v=0:
0=u−10n
- This implies:
u=10n
5. Substituting for n:
- From the previous step, we have u=10n. We can substitute this into the distance equation:
5=10n−5(2n−1)
- Simplifying gives:
5=10n−10n+5
- This equation is satisfied for any n≥1.
6. Finding Possible Values of u:
- Since n must be greater than or equal to 1, we can substitute values for n:
- If n=1, then u=10×1=10m/s.
- If n=2, then u=10×2=20m/s.
- If n=3, then u=10×3=30m/s.
- If n=4, then u=10×4=40m/s.
Conclusion:
The possible values of u are 10m/s,20m/s,30m/s, and 40m/s.
- The particle is projected upwards with an initial velocity u.
- It moves upwards until it reaches its maximum height, where its velocity becomes zero.
- The distance covered in the last second before reaching the maximum height is given as 5 m.
- The distance covered in the nth second can be calculated using the formula:
Sn=u+a2⋅(2n−1)
- Here, Sn is the distance covered in the nth second, u is the initial velocity, a is the acceleration (which is −g or −10m/s2 in this case), and n is the total time taken to reach the maximum height.
- Since the distance covered in the last second (which is the nth second) is 5 m, we can write:
5=u+−102⋅(2n−1)
- Simplifying this gives:
5=u−5(2n−1)
- Rearranging gives:
5=u−10n+5
- Thus:
u=10n
- The time n taken to reach the maximum height can also be found using the equation:
v=u+at
- At maximum height, the final velocity v=0:
0=u−10n
- This implies:
u=10n
- From the previous step, we have u=10n. We can substitute this into the distance equation:
5=10n−5(2n−1)
- Simplifying gives:
5=10n−10n+5
- This equation is satisfied for any n≥1.
- Since n must be greater than or equal to 1, we can substitute values for n:
- If n=1, then u=10×1=10m/s.
- If n=2, then u=10×2=20m/s.
- If n=3, then u=10×3=30m/s.
- If n=4, then u=10×4=40m/s.
The possible values of u are 10m/s,20m/s,30m/s, and 40m/s.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE ( D.(MULTIPLE CHOICE QUESTIONS))
9 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE (ASSERTION REASON)
9 Videos
- MOTION IN A STRAIGHT LINE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - COMPETITION FILE ( B.(MULTIPLE CHOICE QUESTIONS))
74 Videos
- MOTION IN A PLANE BOOK - MODERN PUBLICATIONCHAPTER - MOTION IN A PLANE EXERCISE - Chapter Practice Test
15 Videos
- OSCILLATIONS BOOK - MODERN PUBLICATIONCHAPTER - OSCILLATIONS EXERCISE - Practice Test (For Board Examination)
12 Videos
Similar Questions
A particle is projected vertically upwards from ground with an initial speed of 57m/s .If acceleration due to gravity is taken to be equal to 10m/s2 ,then the displacement of the particle in the sixth second of its motion will be?
View SolutionA body is projected vertically upwards with velocity of 98m/s If acceleration due to gravity ceases to act after 5 seconds, distance travelled by the body in the next 5 seconds is
View Solution
Knowledge Check
A particle is projected vertically upwards with a velocity √gR, where R denotes the radius of the earth and g the acceleration due to gravity on the surface of the earth. Then the maximum height ascended by the particle is
AR2BRC2RD5R4The initial velocity of the particle is 10m/sec and its retardation is 2m/sec2. The distance moved by the particle in 5th second of its motion is
A1mB19mC50mD75mA particle of mass m is projected with an initial velocity U at an angle θ to the horizontal. The torque of gravity on projectile at maximum height about the point of projection is
AmU2Sin2θ2BmU2Sin2θCmU2Sinθ2D12mU2(Sin2θ)2
A particle is projected vertically upwards with an initial velocity of 40m/s. Find the displacement and distance covered by the particle in 6s. Take g=10m/s2.
View SolutionA rocket is fired upwards such that its engine takes 10 s to explods fully. Its velocity- time graph is as shown in the figure. Acceleration due to gravity is 10m/s2.
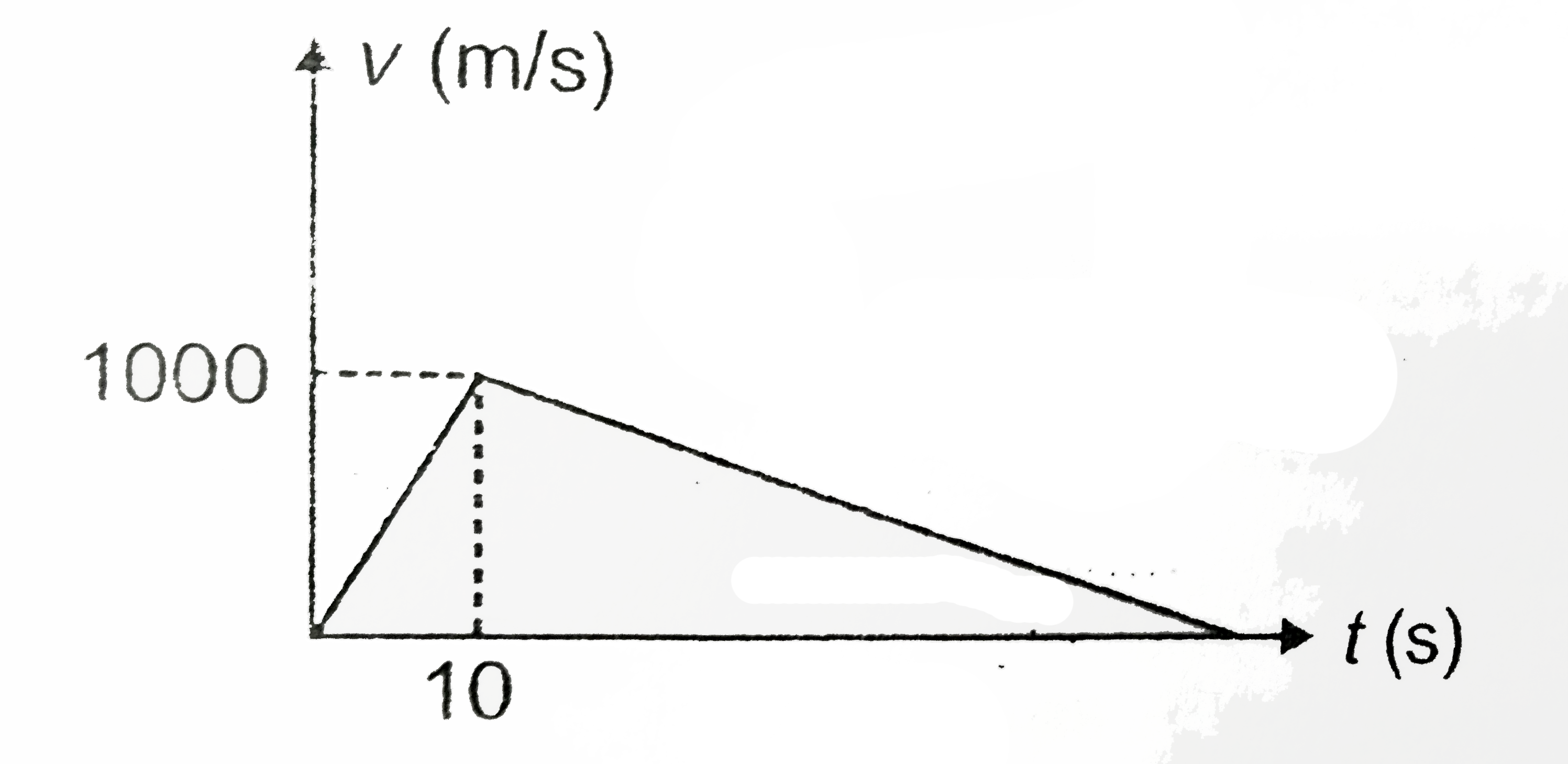
The maximum height attained by the rocket is
View SolutionThe height reached in time t by a particle thrown upward with a speed u is given by
h=ut−12gt2
where g is acceleration due to gravity. Find the time taken in reaching the maximum height.
View SolutionThree particles are projected upwards with initial speeds 10m/s,20m/s,30m/s. The displacements covered by them in their last second of motion are x1,x2,x3 then:
View SolutionA particle has an initial velocity of 9m/s due east and a constant acceleration of 2m/s2 due west. The displacement coverd by the particle in the fifth second of its motion is :
View Solution
