A particle is taken from point A to point B under the influence of a force field. Now it is taken back from B to A and it is observed that the work done in taking the particle from A to B is not equal to the work done in taking it from B to A. If Wnc and Wc are the work done by non-conservative and conservative forces present in the system, respectively, ΔU is the change in potential energy and Δk is the change in kinetic energy, then
(a) Wnc−ΔU=Δk
(b) Wc=−ΔU
(c) Wnc+Wc=Δk
(d) Wnc−ΔU=−Δk
The correct Answer is:A, B, C
To solve the problem, we need to analyze the situation based on the principles of work, energy, and the characteristics of conservative and non-conservative forces.
1. Understanding the Work-Energy Theorem:
The work-energy theorem states that the total work done on an object is equal to the change in its kinetic energy. Mathematically, this can be expressed as:
Wtotal=ΔK
where Wtotal is the total work done by all forces acting on the particle, and ΔK is the change in kinetic energy.
Hint: Recall that the total work done includes contributions from both conservative and non-conservative forces.
2. Separating Work Done by Forces:
The total work done can be separated into work done by conservative forces (Wc) and work done by non-conservative forces (Wnc):
Wtotal=Wc+Wnc
Therefore, we can rewrite the work-energy theorem as:
Wc+Wnc=ΔK
Hint: Identify the types of forces acting on the particle and how they contribute to the work done.
3. Work Done by Conservative Forces:
For conservative forces, the work done is related to the change in potential energy (U). The work done by conservative forces can be expressed as:
Wc=−ΔU
where ΔU is the change in potential energy.
Hint: Remember that the work done by conservative forces is path-independent and depends only on the initial and final positions.
4. Combining the Equations:
Substituting the expression for Wc into the work-energy theorem gives us:
−ΔU+Wnc=ΔK
Rearranging this, we find:
Wnc=ΔK+ΔU
Hint: This equation shows how non-conservative work relates to changes in kinetic and potential energy.
5. Conclusion:
Based on the analysis, we can conclude that:
- The work done by conservative forces is equal to the negative change in potential energy.
- The total work done includes contributions from both conservative and non-conservative forces, leading to changes in kinetic energy.
Therefore, the correct statements based on the relationships derived are:
- Wc=−ΔU
- Wnc+Wc=ΔK
- Wnc=ΔK+ΔU
The correct options are A, B, and C.
Final Answer:
The correct options are A, B, and C.
The work-energy theorem states that the total work done on an object is equal to the change in its kinetic energy. Mathematically, this can be expressed as:
Wtotal=ΔK
where Wtotal is the total work done by all forces acting on the particle, and ΔK is the change in kinetic energy.
The total work done can be separated into work done by conservative forces (Wc) and work done by non-conservative forces (Wnc):
Wtotal=Wc+Wnc
Therefore, we can rewrite the work-energy theorem as:
Wc+Wnc=ΔK
For conservative forces, the work done is related to the change in potential energy (U). The work done by conservative forces can be expressed as:
Wc=−ΔU
where ΔU is the change in potential energy.
Substituting the expression for Wc into the work-energy theorem gives us:
−ΔU+Wnc=ΔK
Rearranging this, we find:
Wnc=ΔK+ΔU
Based on the analysis, we can conclude that:
- The work done by conservative forces is equal to the negative change in potential energy.
- The total work done includes contributions from both conservative and non-conservative forces, leading to changes in kinetic energy.
- Wc=−ΔU
- Wnc+Wc=ΔK
- Wnc=ΔK+ΔU
The correct options are A, B, and C.
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension
55 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Integer
14 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Single Correct
100 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
Knowledge Check
Work done by the conservative force on a system is equal to :
Athe change in kinetic energy of systemBthe change in potential energy of systemCthe change in total machanical energy of systemDNone of the aboveThe negative of the work done 'by the conservative internal forces on a system equals the change in:
Atotal energyBkinetic energyCpotential energyDnone of theseThe work done in taking a unit positive charge from P to A is WA and P to B is WB. Then
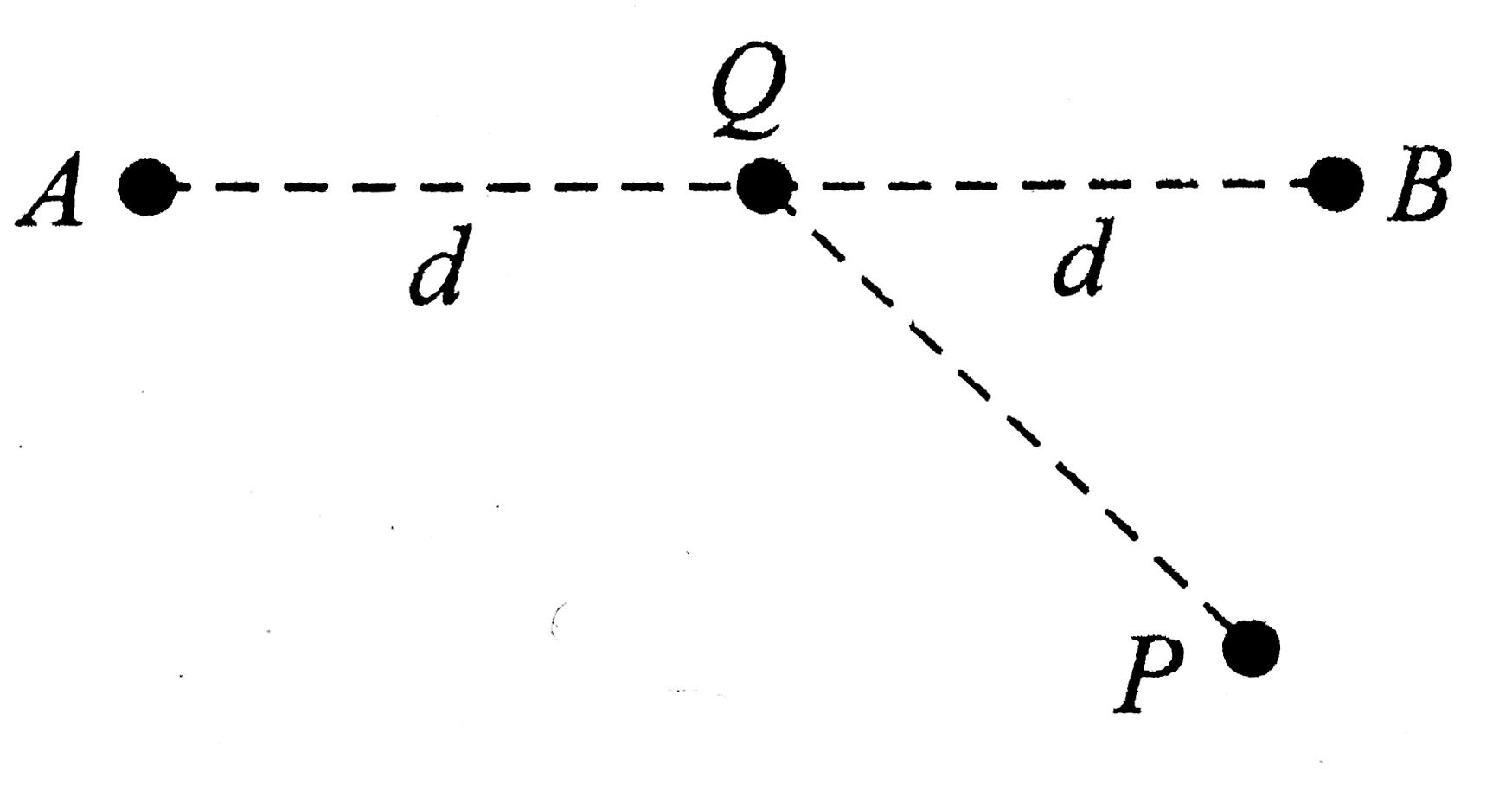 .AWA>WBBWA<WBCWA=WBDWA+WB=0
.AWA>WBBWA<WBCWA=WBDWA+WB=0
A body moves from a point A to B under the action of a force shown in figure. What is the amount of work done ?
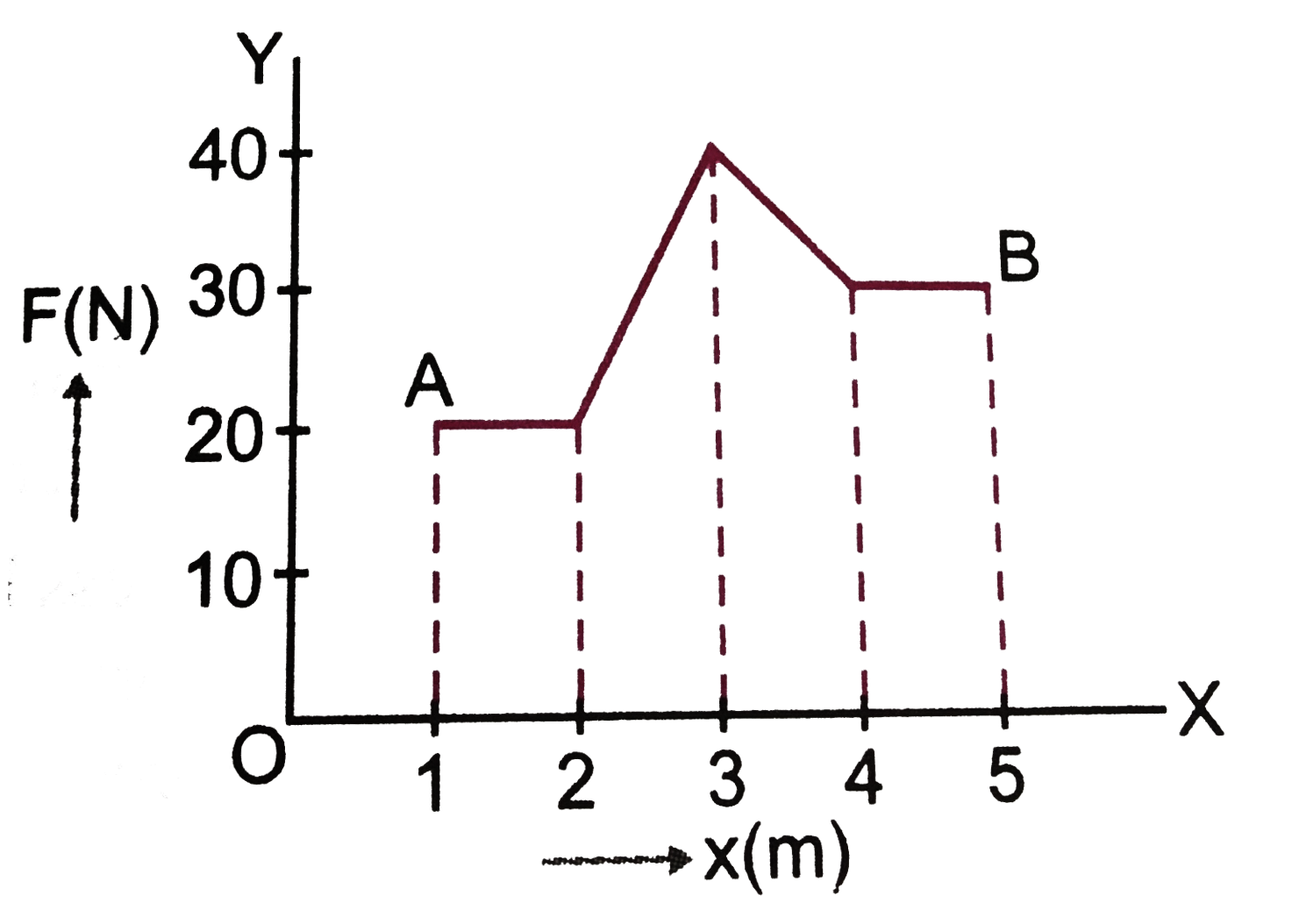
View SolutionA body moves from point A to point B under the action of a force varying in magnitude as shown in the force displacement graph. Find total work done by the force :
View SolutionA charge is taken from a point A to a point B. The work done per unit charge in the process is called
View SolutionA charge is taken a point A to a point B. The work done per unit charge in the process is called
View SolutionA particle is moving in a conservative force field from point A to point B.UA and UB are the potential energies of the particle at point A and B and WC is the work done by conservative forces in the process of taking the particle from A and B, which of the following is true?
View Solution