A particle moves in a closed orbit around the origin, due to a force which is directed towards the origin. The de-broglie wavelength of the particles varries cyclists between two valuse λ1,λ2withλ1>λ2. Which of the following statements are true?
The particle could be moving in a circular orbit with origin as centre
The particle could be moving in an elliptic orbit with origin as its focus
When the de-broglie wavelength is λ1, the particle is nearer the origin than when its value is λ2
When the de-broglie wavelength is λ2, the particle is nearer the origin than when its value is λ1
The correct Answer is:b,d
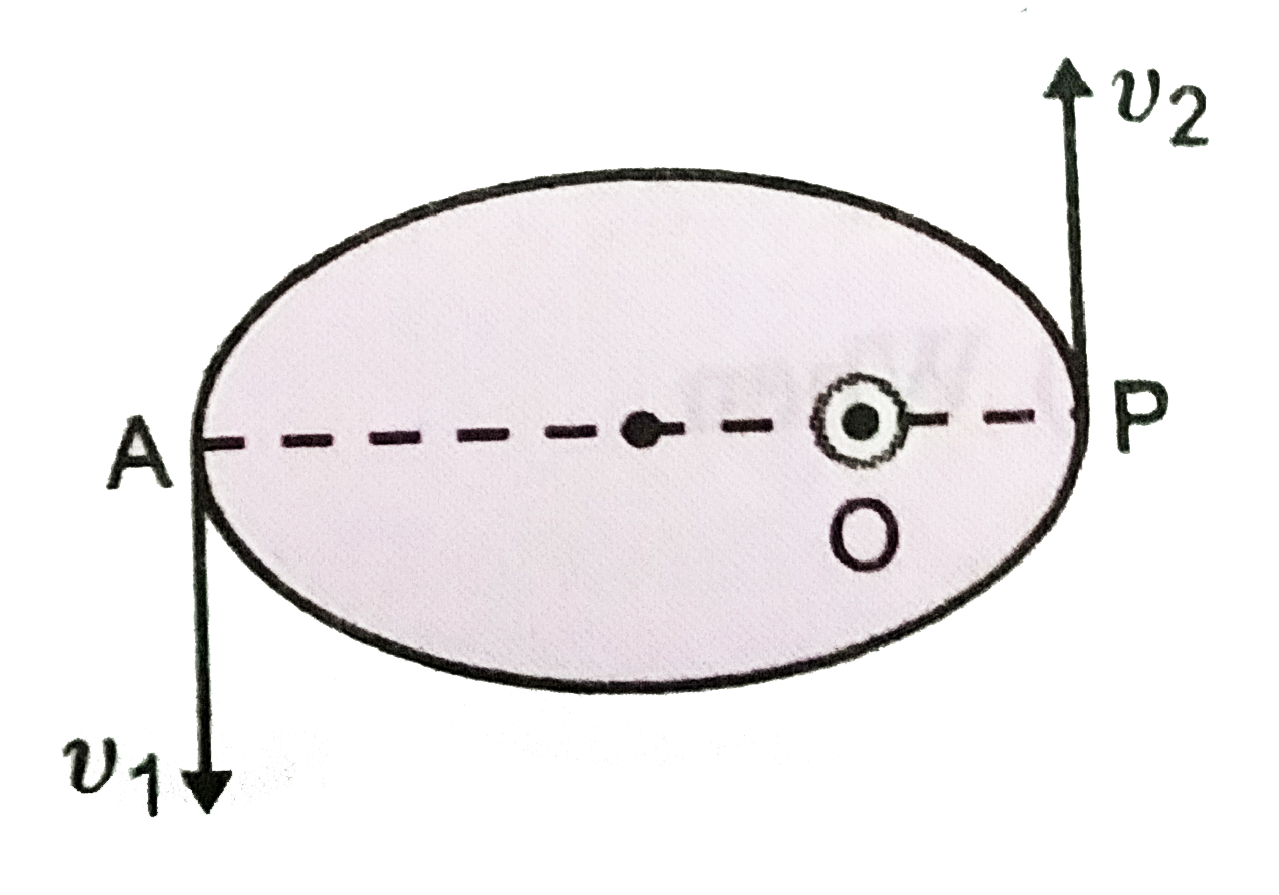
The de-Broglie wavelength of the particle can be varying cyclically between two values λ1andλ2, if particle is moving in an elliptical orbit with origin as its one focus. Let v1,v2 be the speed of particle at A and B respectively and origin is at focus O. If λ1,λ2 are the de-Broglie wavelength associated with particle while moving at A and B respectively, then
λ1=hmv1andλ2=hmv2
∴λ1λ2=v2v1,Sinceλ1>λ2∴v2>v1
From figure we note that origin O is close to P than A. thus options (b) and (d) are true.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Value based question
1 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)
2 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions
1 Videos
Similar Questions
Knowledge Check
The de-Broglie wavelength of an electron, an α -particle and a proton are λe,λα,λp. Which is wrong from the following:
Aλe>λpBλe<λpCλp>λαDλe>λp>λαThe de-Broglie wavelength of an electron, an α-particle and a proton are λe,λa,λp. Which is wrong from the following?
Aλe>λpBλe<λpCλp>λαDλe>λp>λαIf →aand→b are two unit vectors perpenicualar to each other and →c=λ1→a+λ2→b+λ3(→a×→b), then which of the following is (are) true ?
Aλ1=→a.→cBλ2=∣∣→b×→c∣∣Cλ3=∣(→a×→b|×→c|Dλ1→a+λ2→b+λ3(→a×→b)
A particle of mass 3 m at rest decay Into two particles of masses m and 2m having non-zero velocities. What is the ratio of the de-Broglie wavelengths of the particle (λ1/λ2).
View SolutionTwo sound waves of wavelengths λ1 and λ2(λ2>λ1) produces nbeats/s , the speed of sound is
View SolutionA charged particle is associated from rest through a certain potential difference. The de-Broglie wavelength is λ1 when it is accelerated through V1 and is λ2 when accelerated through V2. The ratio λ1/λ2 is
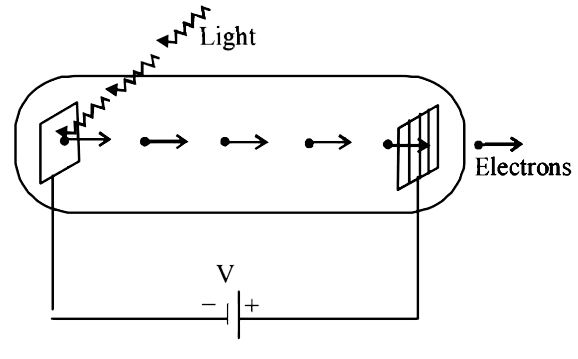
View SolutionLight of wavelength λphfalls on a plate a vacum teke as shown in the figure .The work function of the conducting meterial kept at a distance d from the cathon A petential different V is maximum between the electrodes if the minimum de Brogle waveleeength of the electrons passing through the anode is λe which of the following statement (s) is (are) true?
View SolutionA particle of mass M at rest decay's into two particle of masses m1 and m2 having non zero velocity. The ratio of the de Broglie wavelengths of the masses λ1/λ2 is
View Solution
