A particle moves in a straight line. Figure-1.21 shows the distance traversed by the particle as a function of time t.Using the graph, find (a)the average velocity of the point during the time of motion, (b) the maximum velocity, (c) the time t=t0 at which the instantaneous velocity is equal to the mean velocity averaged over the first t0 seconds.
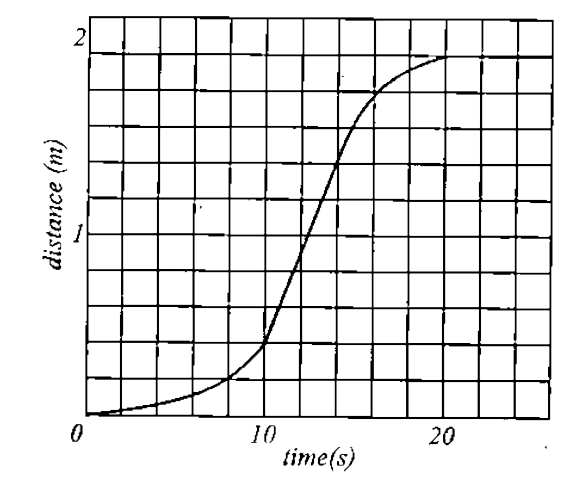
The correct Answer is:[(a) 10 cm/s, (b) 25 cm/s, (c) 16 s]
Step by step video, text & image solution for A particle moves in a straight line. Figure-1.21 shows the distance traversed by the particle as a function of time t.Using the graph, find (a)the average velocity of the point during the time of motion, (b) the maximum velocity, (c) the time t = t_(0) at which the instantaneous velocity is equal to the mean velocity averaged over the first t_(0) seconds. by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Discussion Question
18 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Conceptual MCQs Single Option Correct
35 Videos
- KINEMATICSBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - KINEMATICSEXERCISE - Unsolved Numerical Pro.
83 Videos
- GRAVITATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - GRAVITATIONEXERCISE - Unsolved Numerical
94 Videos
- LINEAR MOMENTUM & ITS CONSERVATIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - LINEAR MOMENTUM & ITS CONSERVATIONEXERCISE - exercise 1.3
3 Videos
Similar Questions
A point moves rectilinearly in on direction. Figure shows
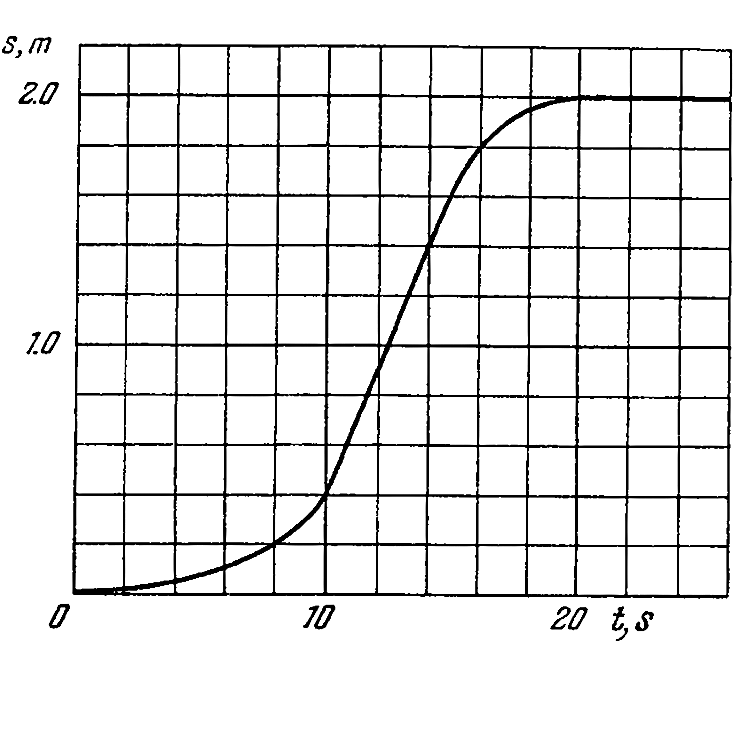
the distance s traversed by the point as a function of the time t. Using the plot find:
(a) the average velocity of the point during the time of motion,
(b) the maximum velocity,
(c) the time moment t0 at which the instantaneous velocity is equal to the mean velocity averaged over the first t0 seconds.
View SolutionA particle moves with a velocity v(t)=(12)kt2 along a straight line . Find the average speed of the particle in a time t.
View Solution
Knowledge Check
Position time graph is shown which is a semicircle form t=2 to t=8sec. Find time t at which the instantaneous velocity, is equal to average velocity over first t second.
 A4.8secB3.2secC2.4secD5sec
A4.8secB3.2secC2.4secD5secA point traversed 3/4 th of the circle of radius R in time t. The magnitude of the average velocity of the particle in this time interval is
AπRtB3πR2tCR√2tDR√2tThe diagram shows the displacement-time graph for a particle moving in a straight line. Find the average velocity for the interval from t=0 to t=5s.
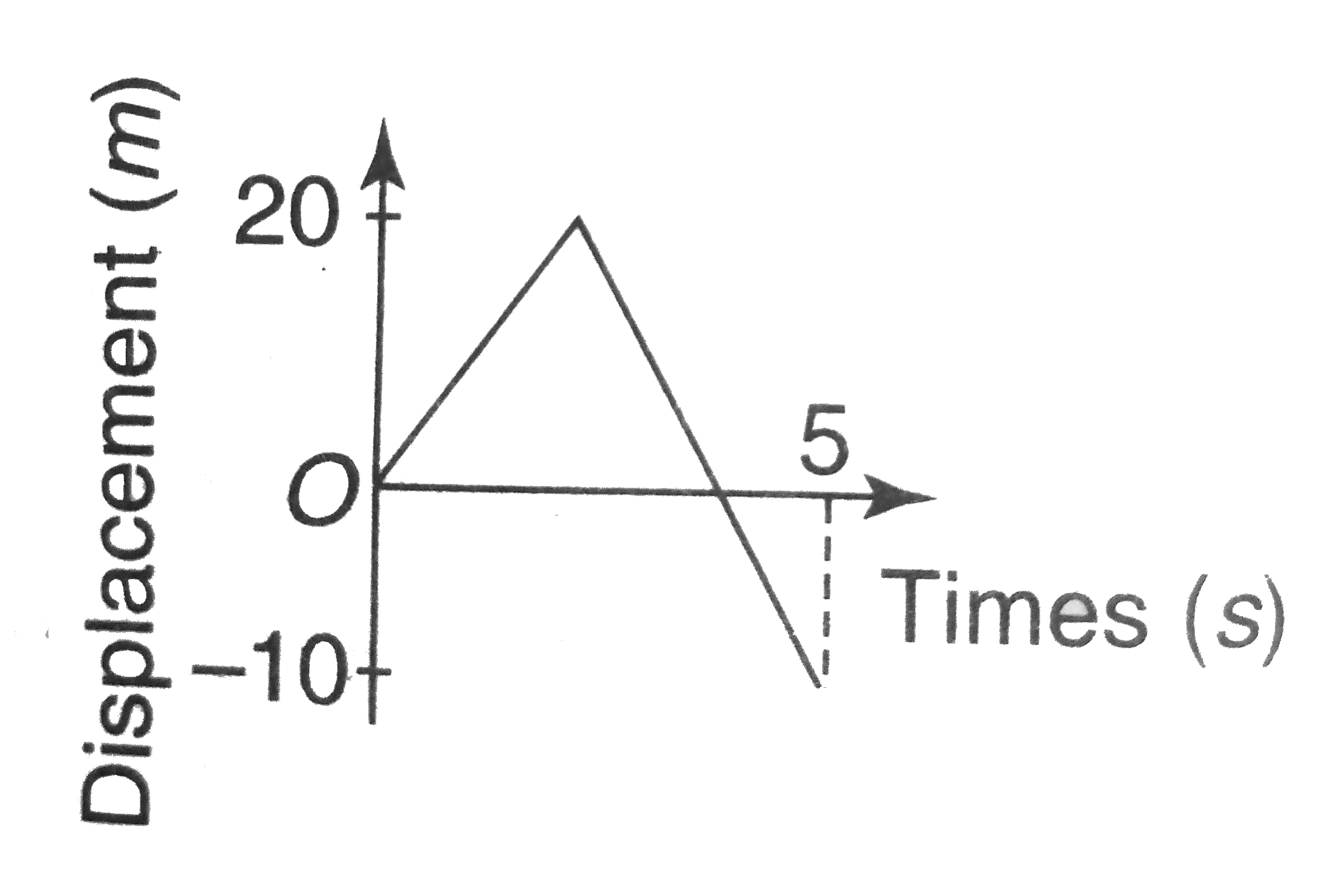 A−2ms−1B2ms−1C−4ms−1D4ms−1
A−2ms−1B2ms−1C−4ms−1D4ms−1
From the velocity time plot shown in figure find the distance travelled by the particle dureing the first 40 seconds. Also find the average velocity during this period.

View SolutionA particle is projected with a speed v and an angle θ to the horizontal. After a time t, the magnitude of the instantaneous velocity is equal to the magnitude of the average velocity from 0 to t. Find t.
View SolutionFigure shows the graph of the x-coordinaste of a particle going along the X-axis as a function of time.Find a. te average velocity during 0 to 10s, b. instantaneous velocity at 2,5,8 and 12s.

View SolutionThe diagram shows the displacement-time graph for a particle moving in a straight line. Find the average velocity for the interval from t=0 to t=5s.

View SolutionThe fig. shows the displacement time graph of a particle moving on a straight line path. What is the magnitude of average velocity of the particle over 10 seconds?
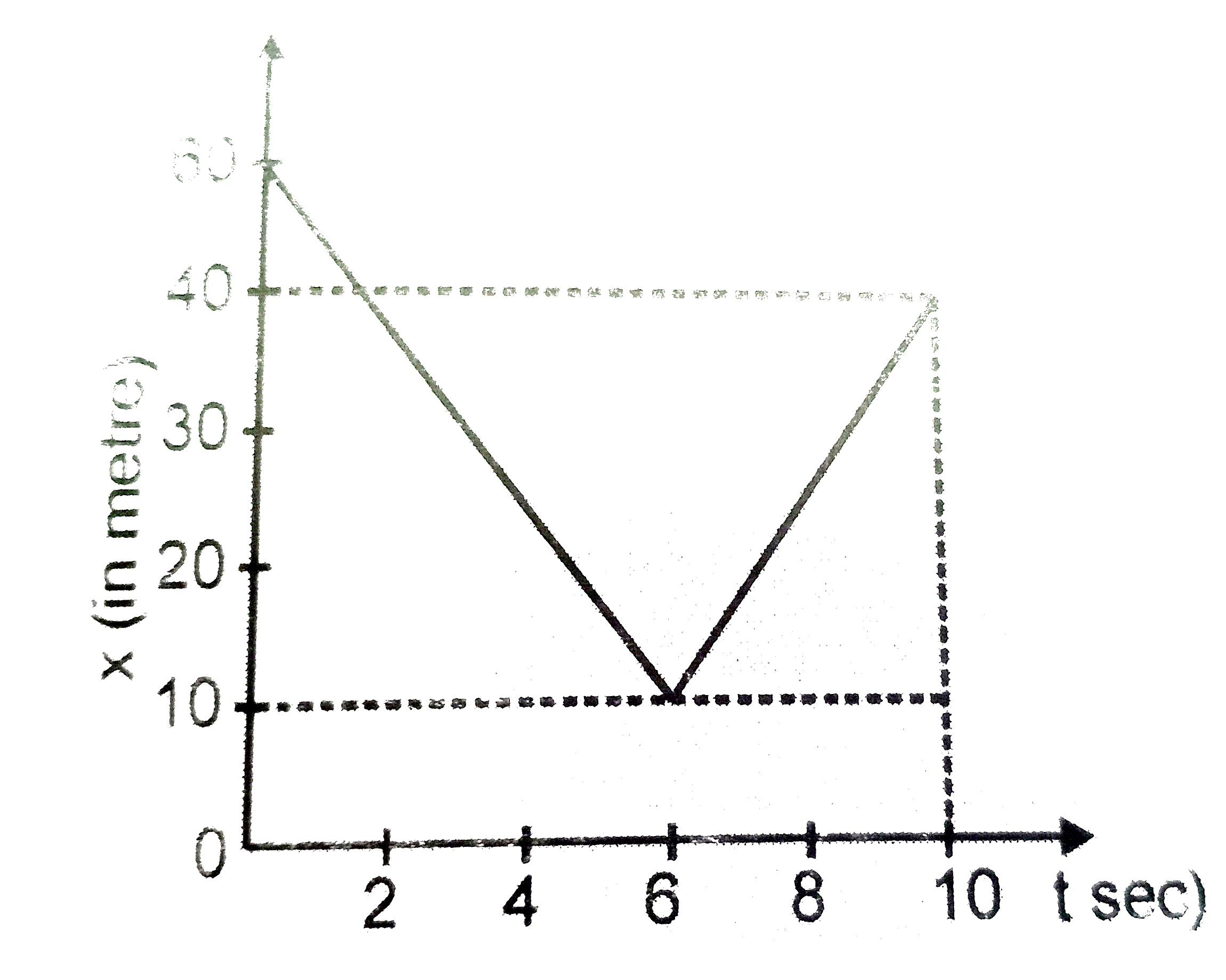
View Solution
