A particle, moving horizontally, collides perpendicularly at one end of a rod having equal mass and placed on a smooth horizontal surface.

Particle comes to rest if collision is perfectly elastic and centre of m rod starts to move with the same velocity.
Particle continues to move along the same direction, whatever is the value of e.
Particle may get rebound back.
Velocity of mid-point of the rod will be less than v/2 if the particle gets stuck.
The correct Answer is:B, D
Whenever two paticles having equal mass collide head on elastically, their velocities get interchanged. Therefore, if the particle collides at midpoint of the rod then velocities would get interchanged. In that case option a would be correct. But now the particle strikes at an end of the rod, hence particle head on collision does not take place. Therefore, option a is incorrect.
If the particle gets rebound back to its original path, then its final mometum will become negative. Since mass of the particle and rod is equal, therefore law of conservation of momenum will be satisfied only when velocity of centre of mass of the rod is greater than orginal velocity of te particle. Hence, kinetic energy of the system (just after collision) will become greater than that (just before the collision), which is impossible. Hence, the particle cannot rebound back or it will colntinue to move along the same direction. Hence, option b is correct but option c is incorrect. if the paticle gets stick to the rod, centre of mass o the system will lie at a distance l/4 from theend at which the particle sticks. According to the law of conservation of momentum cenre of mass will start ot move wit velocity v/2. But the particle will simultaneously start to rotate about tecentre of mass m an anticlockwise direction. Angular velocity ω of that rotational motion can be calculated by applying law of mass m an anticlockwise direction. Angular velocity ω of that rotational motion can be calculated by applying law of conservation of angular momentum. Hence the resultant velocity of the midpoint of the rod will be equal to [(v0)/2)−(lω/4)] which is obviously less than v0/2. Therefore option d correct.
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Multiple Correct
26 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Linked Comprehension
71 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Subjective
19 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Integer
11 Videos
- SOUND WAVES AND DOPPLER EFFECTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - SOUND WAVES AND DOPPLER EFFECTEXERCISE - Integer
16 Videos
Similar Questions
A man of mass m is standing at one end of a trolley of mass Mand length L placed at rest on a smooth horizontal surface. The man starts moving towards the other end and covers distance L, then distance moved by man w.r.t ground is
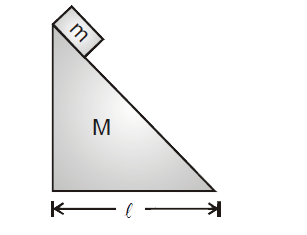
View SolutionA particle of mass m is placed at rest on the top of a smooth wedge of mass M, which in turn is placed at rest on a smooth horizontal surface as shown in figure. Then the distance moved by the wedge as the particle reaches the foot of the wedge is :
View SolutionA uniform rod is kept vertically on a horizontally smooth surface at a point O. IF it is rotated slightly and released, it falls down on the horizontal surface. The lower end will remain
View SolutionA block of mass m is placed at rest on a smooth wedge of mass M placed at rest on a smooth horizontal surface. As the system is released

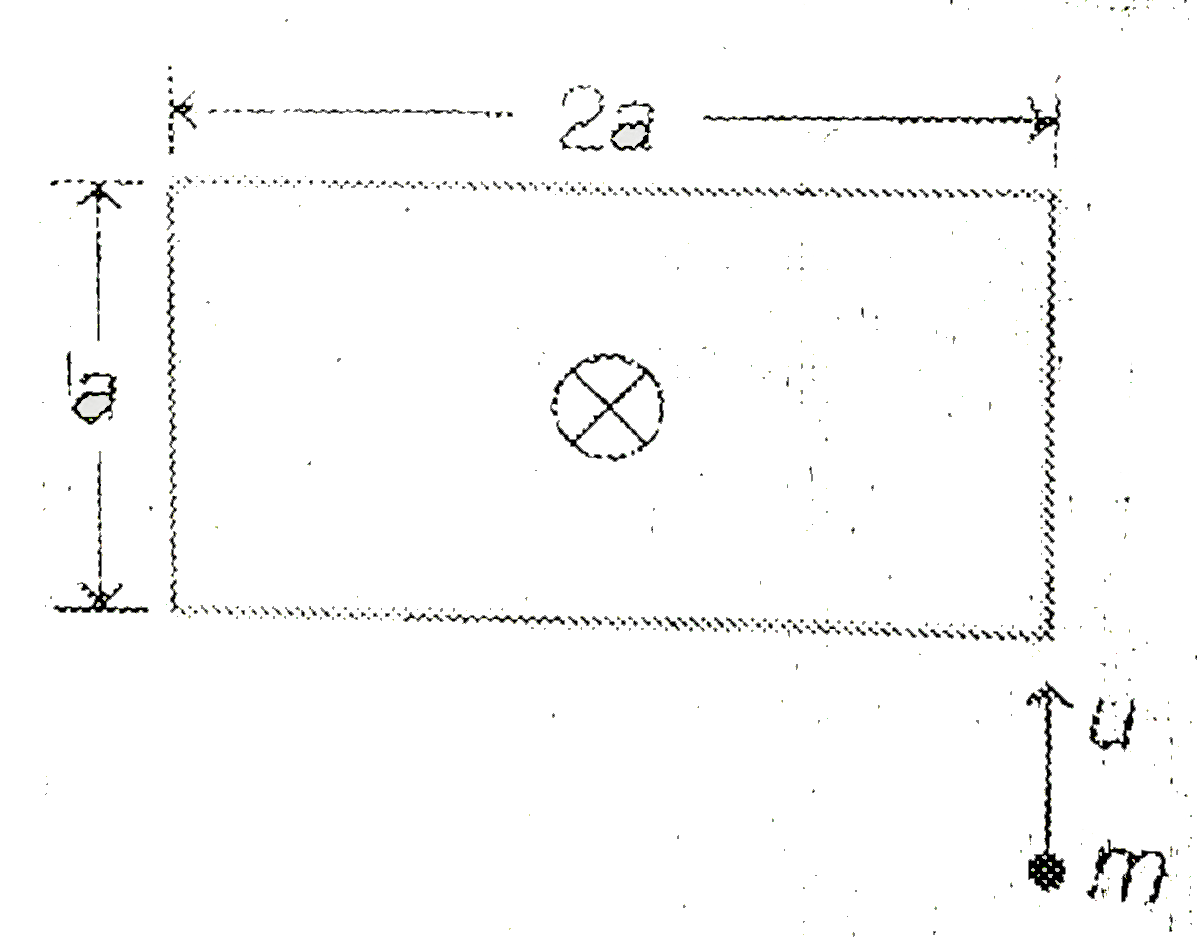
View SolutionA uniform rectangular plate of mass m which is free to rotate about the smooth vwerticle hinge passing through the centre and perpendicular to the plate, is lying on a smooth horizontal surface. A particle of mass m is moving with speed 'u' collides with the plate and sticks to it as shown in figure. The angular velocity of the platye after colli9sion will be
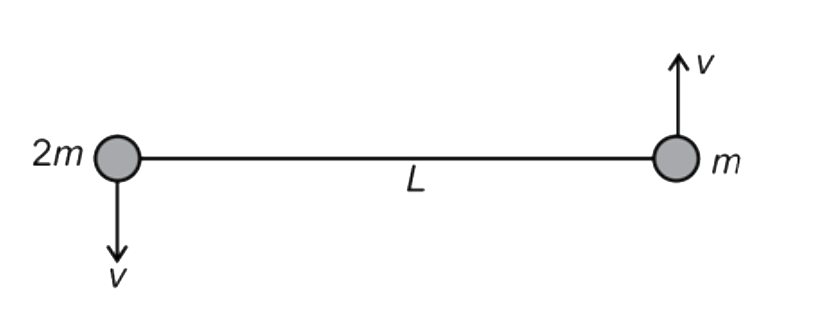
View SolutionTwo particles of mass m and 2m are connected by a string of length L and placed at rest over a smooth horizontal surface. The particles are then given velocities as indicated in the figure shown. The tension developed in the string will be
View SolutionA particle of mass m moving with a speed v hits elastically another staionary particle of mass 2m on a smooth horizontal circular tube of radius r. Find the time when the next collision will take place?
View SolutionA particle of mass m moving with a speed v hits elastically another staionary particle of mass 2m on a smooth horizontal circular tube of radius r. Find the time when the next collision will take place?
View SolutionA particle of mass m moving with a speed v hits elastically another staionary particle of mass 2m on a smooth horizontal circular tube of radius r. Find the time when the next collision will take place?
View SolutionA uniform rod of length L lies on a smooth horizontal table. The rod has a mass M. A particle of mass m moving with speed v strikes the rod perpendicularly at one of the ends of the rod sticks to it after collision.
Find the velocity of the particle with respect to C before the collision
View Solution
