A particle of mass m is projected at an angle α to the horizontal with an initial velocity u. The work done by gravity during the time it reaches its highest point is
(a) u2sin2α
(b) mu2cos2α2
(c) mu2sin2α2
(d) −mu2sin2α2
The correct Answer is:D
To find the work done by gravity on a particle of mass m projected at an angle α with an initial velocity u until it reaches its highest point, we can follow these steps:
Step 1: Understand the motion of the particle
The particle is projected at an angle α with an initial velocity u. The initial velocity can be resolved into two components:
- Horizontal component: \( u_x = u \cos \alpha \)
- Vertical component: \( u_y = u \sin \alpha \)
Step 2: Determine the highest point of the trajectory
At the highest point of its trajectory, the vertical component of the velocity becomes zero. The horizontal component remains unchanged since there are no forces acting in the horizontal direction.
Step 3: Calculate the maximum height (h)
Using the kinematic equation for vertical motion, we can find the maximum height \( h \) reached by the particle:
\(
h = \frac{u_y^2}{2g} = \frac{(u \sin \alpha)^2}{2g} = \frac{u^2 \sin^2 \alpha}{2g}
\)
Step 4: Calculate the work done by gravity
The work done by gravity can be calculated using the formula:
\(
W = -mgh
\)
Substituting the expression for \( h \):
\(
W = -mg \left( \frac{u^2 \sin^2 \alpha}{2g} \right)
\)
The \( g \) cancels out:
\(
W = -\frac{mu^2 \sin^2 \alpha}{2}
\)
Step 5: Conclusion
Thus, the work done by gravity during the time it reaches its highest point is:
\(
W = -\frac{mu^2 \sin^2 \alpha}{2}
\)
Final Answer
The work done by gravity is \( -\frac{mu^2 \sin^2 \alpha}{2} \).
---
The particle is projected at an angle α with an initial velocity u. The initial velocity can be resolved into two components:
- Horizontal component: \( u_x = u \cos \alpha \)
- Vertical component: \( u_y = u \sin \alpha \)
At the highest point of its trajectory, the vertical component of the velocity becomes zero. The horizontal component remains unchanged since there are no forces acting in the horizontal direction.
Using the kinematic equation for vertical motion, we can find the maximum height \( h \) reached by the particle:
\(
h = \frac{u_y^2}{2g} = \frac{(u \sin \alpha)^2}{2g} = \frac{u^2 \sin^2 \alpha}{2g}
\)
The work done by gravity can be calculated using the formula:
\(
W = -mgh
\)
Substituting the expression for \( h \):
\(
W = -mg \left( \frac{u^2 \sin^2 \alpha}{2g} \right)
\)
The \( g \) cancels out:
\(
W = -\frac{mu^2 \sin^2 \alpha}{2}
\)
Thus, the work done by gravity during the time it reaches its highest point is:
\(
W = -\frac{mu^2 \sin^2 \alpha}{2}
\)
The work done by gravity is \( -\frac{mu^2 \sin^2 \alpha}{2} \).
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension
55 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective
23 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
A body of mass m is thrown at an angle α to the horizontal with the initial velocity v0. Find the mean power developed by gravity over the wholetime of motion of the body, and the instantaneous power of gravity as a function of time.
View SolutionA particle of mass m is projected with velocity v at an angle θ with the horizontal. Find its angular momentum about the point of projection when it is at the highest point of its trajectory.
View Solution
Knowledge Check
A bode mass m is projected at an angle θ with the horizontal with an initial velocity u, the average power of gravitational force over the whole time of flight is.
AmgucosθB12mg√ucosθC12mgusinθDzeroA particle of mass m is projected at an angle α to the horizontal with an initial velocity u. The work done by gravity during the time it reaches its highest point is
A−mu2sin2θ2Bmu2cos2θ2Cmu2sin2θ2DZeroA ball of mass 'm' is thrown at an angle is 'θ' with the horizontal with an initial velocity 'u'.The change in its momentum during its flight in a time interval of 't' is
AmgtBm>cosθCm>sinθD1/2m>.
A body is projected at angle of 45∘ to the horizontal . What is its velocity at the highest point ?
View SolutionA particle of mass'm' is projected with velocity v0 at an angle 'α' with the horizontal. The coefficient of restitution for any of its impact with the smooth ground is e.
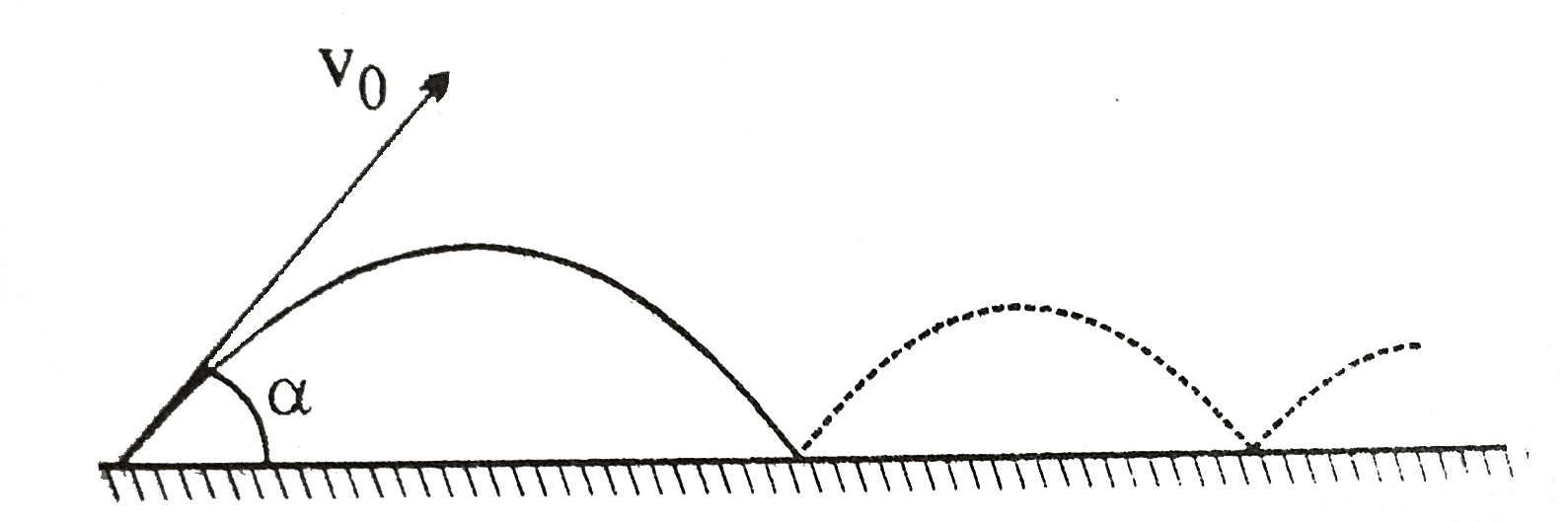
Find total time taken by the particle before it stops moving vertically?
View SolutionA ball of mass 'm' is thrown at an angle is 'θ' with the horizontal with an initial velcoity 'u'. The change in its momentum during its flight in a time interval of 't' is .
View SolutionA particle of mass m is projected with an initial velocity U at an angle θ to the horizontal. The torque of gravity on projectile at maximum height about the point of projection is
View SolutionA projectile is projected at an angle α with an initial velocity u. The time t, at which its horizontal velocity will equal the vertical velocity for the first time
View Solution
