A particle of mass m strikes a horizontal smooth floor with velocity u making an angle θ with the floor and rebound with velocity v making an angle θ with the floor. The coefficient of restitution between the particle and the floor is e. Then
The correct Answer is:A, B, C, D
To solve the problem step by step, we will analyze the motion of the particle before and after it strikes the horizontal smooth floor, taking into account the coefficient of restitution.
Step 1: Analyze Initial and Final Velocities
The particle strikes the floor with an initial velocity u at an angle θ with respect to the horizontal. The components of the initial velocity can be expressed as:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
After rebounding, the particle has a final velocity v at the same angle θ. The components of the final velocity are:
- Horizontal component: vx=vcosθ
- Vertical component: vy=−vsinθ (negative because it is directed upwards after the rebound)
Step 2: Apply the Coefficient of Restitution
The coefficient of restitution e is defined as the ratio of the relative speed after the collision to the relative speed before the collision along the line of impact. For vertical motion, we can write:
e=Speed after collisionSpeed before collision=|vy||uy|
Substituting the expressions for vy and uy:
e=vsinθusinθ
From this, we can derive the relationship between the initial and final vertical velocities:
v=eu
Step 3: Calculate the Impulse
The impulse delivered by the floor to the particle can be calculated using the change in momentum in the vertical direction:
Δpy=m(vy−uy)=m(−vsinθ−usinθ)=m(−vsinθ+usinθ)
Substituting v=eu:
Δpy=m(−eusinθ+usinθ)=musinθ(1−e)
The impulse J is equal to the change in momentum:
J=musinθ(1−e)
Step 4: Analyze the Horizontal Motion
Since the floor is smooth, there is no friction, and the horizontal component of the velocity remains unchanged:
ux=vx⟹ucosθ=vcosθ
Thus, the horizontal component of the velocity does not change during the collision.
Step 5: Calculate the Final Velocity Magnitude
The magnitude of the final velocity can be calculated using the Pythagorean theorem:
v=√(vx)2+(vy)2=√(vcosθ)2+(−vsinθ)2=√v2(cos2θ+sin2θ)=v
Step 6: Kinetic Energy Before and After Collision
The initial kinetic energy KEi and final kinetic energy KEf can be expressed as:
KEi=12mu2
KEf=12mv2=12m(eu)2=12me2u2
The ratio of final to initial kinetic energy is:
KEfKEi=e21
Summary of Results
1. The relationship between the final and initial vertical velocities is v=eu.
2. The impulse delivered by the floor is J=musinθ(1−e).
3. The horizontal component of velocity remains unchanged.
4. The ratio of final to initial kinetic energy is e2.
The particle strikes the floor with an initial velocity u at an angle θ with respect to the horizontal. The components of the initial velocity can be expressed as:
- Horizontal component: ux=ucosθ
- Vertical component: uy=usinθ
- Horizontal component: vx=vcosθ
- Vertical component: vy=−vsinθ (negative because it is directed upwards after the rebound)
The coefficient of restitution e is defined as the ratio of the relative speed after the collision to the relative speed before the collision along the line of impact. For vertical motion, we can write:
e=Speed after collisionSpeed before collision=|vy||uy|
Substituting the expressions for vy and uy:
e=vsinθusinθ
From this, we can derive the relationship between the initial and final vertical velocities:
v=eu
The impulse delivered by the floor to the particle can be calculated using the change in momentum in the vertical direction:
Δpy=m(vy−uy)=m(−vsinθ−usinθ)=m(−vsinθ+usinθ)
Substituting v=eu:
Δpy=m(−eusinθ+usinθ)=musinθ(1−e)
The impulse J is equal to the change in momentum:
J=musinθ(1−e)
Since the floor is smooth, there is no friction, and the horizontal component of the velocity remains unchanged:
ux=vx⟹ucosθ=vcosθ
Thus, the horizontal component of the velocity does not change during the collision.
The magnitude of the final velocity can be calculated using the Pythagorean theorem:
v=√(vx)2+(vy)2=√(vcosθ)2+(−vsinθ)2=√v2(cos2θ+sin2θ)=v
The initial kinetic energy KEi and final kinetic energy KEf can be expressed as:
KEi=12mu2
KEf=12mv2=12m(eu)2=12me2u2
The ratio of final to initial kinetic energy is:
KEfKEi=e21
1. The relationship between the final and initial vertical velocities is v=eu.
2. The impulse delivered by the floor is J=musinθ(1−e).
3. The horizontal component of velocity remains unchanged.
4. The ratio of final to initial kinetic energy is e2.
Topper's Solved these Questions
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Comprehension Based
4 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Comprehension Based Questions
3 Videos
- CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, LINEAR MOMENTUM AND COLLISIONEXERCISE - Level 2 Single Correct Option
1 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
- CIRCULAR MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - CIRCULAR MOTIONEXERCISE - Medical entrances s gallery
19 Videos
Similar Questions
A particle is fired from a point on the ground with speed u making an angle θ with the horizontal.Then
View SolutionA ball is thrown onto a smooth floor with speed u at angle θ=45∘. If it rebounds with a speed v at the same angle ϕ=45∘. Then the coefficient of restitution is

View SolutionA ball of a mass m hits the floor with as speed v making an angle of incidence θ with the normal. The coefficient of restitution is e. Find the speed of the reflected ball and the angle of reflection of the ball.
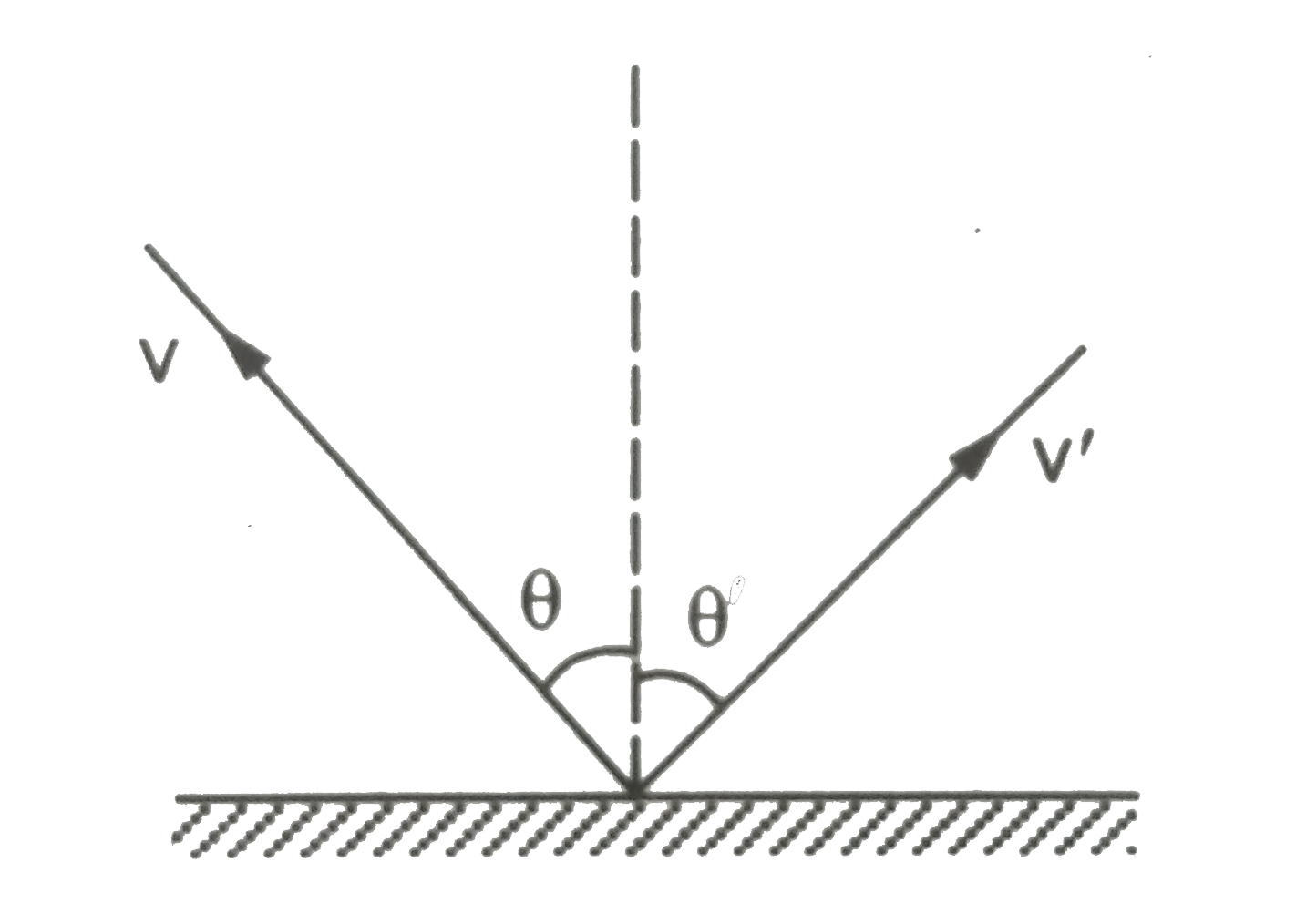
View SolutionA particle is projected from the ground with velocity u making an angle θ with the horizontal. At half of its maximum heights,
View SolutionA particle of mass m is projected form ground with velocity u making angle θ with the vertical. The de Broglie wavelength of the particle at the highest point is
View SolutionA particle is fired with velocity u making angle θ with the horizontal.What is the change in velocity when it is at the highest point?
View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. The instantaneous power of the gravitational force
View SolutionIn the figure, a ladder of mass m is shown leaning against a wall. It is in static equilibrium making an angle θ with the horizontal floor. The coefficient of friction between the wall and the ladder is μ1 and that between the floor and the ladder is μ2. the normal reaction of the wall on the ladder is N1 and that of the floor is N2. if the ladder is about to slip. than
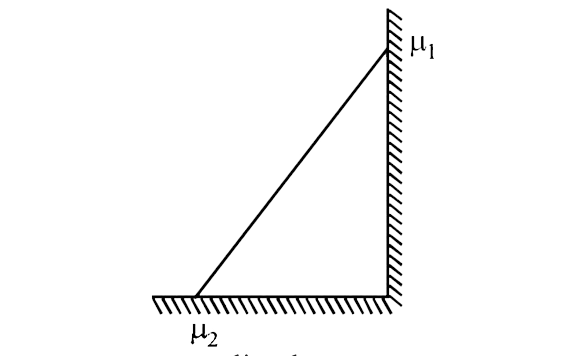
View SolutionA ball of mass m=1kg strikes a smooth horizontal floor as shown in figure. The impulse exerted on the floor is

View SolutionA particle is projected with a velocity u making an angle θ with the horizontal. At any instant its velocity becomes v which is perpendicular to the initial velocity u. Then v is
View Solution
