A particle of mass mo moves with a speed C2. Calculate its mass, momentum, total energy and kinetic energy.
To solve the problem step by step, we will calculate the mass, momentum, total energy, and kinetic energy of a particle of mass m0 moving with a speed of c2.
Step 1: Calculate the relativistic mass
The relativistic mass m can be calculated using the formula:
m=m0√1−v2c2
where:
- m0 is the rest mass,
- v is the velocity of the particle,
- c is the speed of light.
Given that v=c2, we can substitute this into the equation:
m=m0√1−(c2)2/c2=m0√1−c24c2=m0√1−14=m0√34=m0√32=2m0√3
Step 2: Calculate the momentum
The momentum p of the particle can be calculated using the formula:
p=mv
Substituting the values we have:
p=(2m0√3)(c2)=2m0c2√3=m0c√3
Step 3: Calculate the total energy
The total energy E of the particle is given by:
E=mc2
Substituting the mass we calculated in Step 1:
E=(2m0√3)c2=2m0c2√3
Step 4: Calculate the kinetic energy
The kinetic energy KE can be calculated using the formula:
KE=E−E0
where E0 is the rest mass energy given by:
E0=m0c2
Substituting the values we have:
KE=2m0c2√3−m0c2=m0c2(2√3−1)
To simplify this, we can combine the terms:
KE=m0c2(2−√3√3)
Summary of Results
1. Mass: m=2m0√3
2. Momentum: p=m0c√3
3. Total Energy: E=2m0c2√3
4. Kinetic Energy: KE=m0c2(2−√3√3)
- m0 is the rest mass,
- v is the velocity of the particle,
- c is the speed of light.
2. Momentum: p=m0c√3
3. Total Energy: E=2m0c2√3
4. Kinetic Energy: KE=m0c2(2−√3√3)
Topper's Solved these Questions
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 5EXERCISE - Section-C9videos
SELF ASSESSMENT PAPER 5
- BOOK - ICSECHAPTER - SELF ASSESSMENT PAPER 4EXERCISE - Section-D6videos
SELF ASSESSMENT PAPER 4
- BOOK - ICSECHAPTER - THERMAL CONDUCTIONEXERCISE - SELECTED PROBLEMS (Taken from the Previous Years ISC, AISSCE, HSSCE various States. Boards Roorke Qns & NCERT text) FROM EXPERIMENT TO DETERMINE K2videos
THERMAL CONDUCTION
Similar Questions
A particle of mass m has momentum p. Its kinetic energy will be
View SolutionA particle of mass m1 is moving with a velocity v1 and another particle of mass m2 is moving with a velocity v2. Both of them have the same momentum but their different kinetic energies are E1 and E2 respectively. If m1>m2 then
View SolutionA particle of mass 1kg is moving about a circle of radius 1m with a speed of 1m/s. Calculate the angular momentum of the particle.
View SolutionA particle of mass 1kg is moving about a circle of radius 1m with a speed of 1m/s. Calculate the angular momentum of the particle.
View SolutionA rod of mass m spins with an angular speed ω=√gl, Find its a. kinetic energy of rotation. b. kinetic energy of translation c. total kinetic energy.
 View Solution
View SolutionA particle of mass m and charge q is placed at rest in a uniform electric field E and then released, the kinetic energy attained by the particle after moving a distance y will be
View SolutionA particle of mass m has half the kinetic energy of another particle of mass m2. If the speed of the heavier particle is increased by 2ms−1 its new kinetic energy becomes equal to t he original kinetic energy of the lighter particle. The ratio of the orighinal speeds of the lighter and heavier particle is
View SolutionThe kinetic energy of a particle of mass m moving with speed v is given by K=12mv2. If the kinetic energy of a particle moving along x-axis varies with x as K(x)=9−x2, then The region in which particle lies is :
View SolutionA particle of mass m moves along line PC with velocity v as shown. What is the angular momentum of the particle about O?
 View Solution
View SolutionTwo particles A and B of mass 3kg and 2kg moving with speed 10m/s and 5m/s as shown in figure.
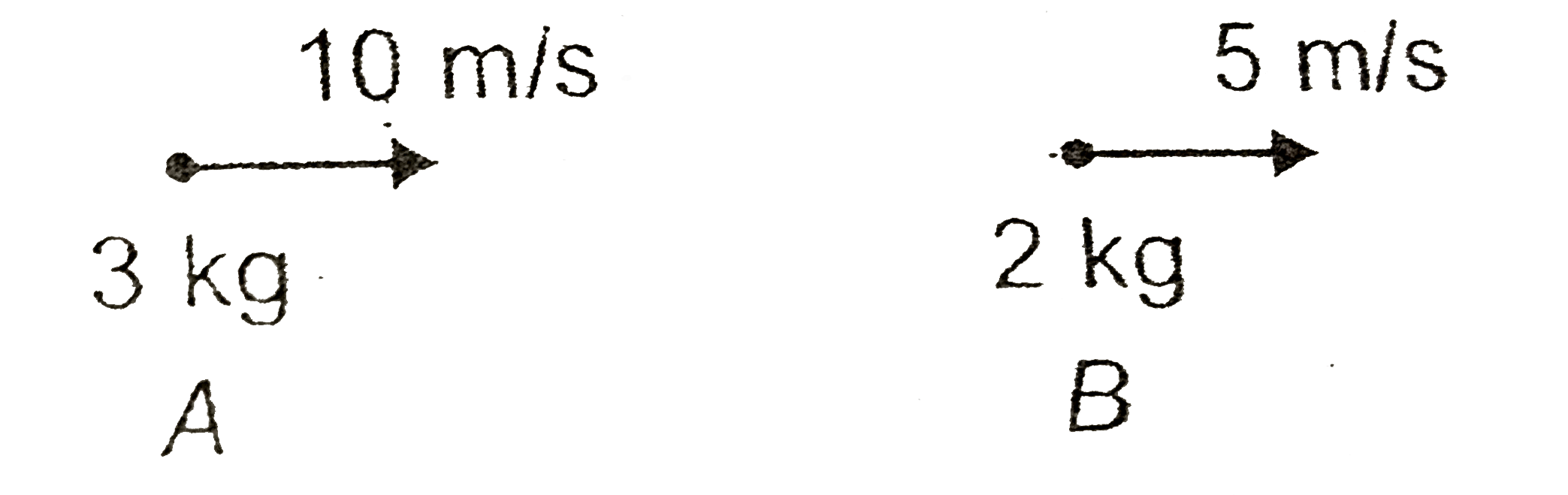
Kinetic energy of particle A when particle B has the maximum momentumView Solution
