A particle of specific charge 'α' is projected from origin at t=0 with a velocity →V=V0(ˆi−ˆk) in a magnetic field →B=−B0ˆk. Then : (Mass of particle =1 unit)
At t=παB0, velocity of the particle is −V0(ˆi−ˆk)
At t=π4αB0, speed of the particle is V0
At t=2παB0, magnitude of displacement of the particle is more than 2V0αB0
At t=2παB0, distance travelled by the particle is less than 2√2πV0αB0.
Step by step video & image solution for A particle of specific charge 'alpha' is projected from origin at t=0 with a velocity vec(V)=V_(0) (hat(i)-hat(k)) in a magnetic field vec(B)= -B_(0)hat(k). Then : (Mass of particle =1 unit) by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - U.N.P79videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - MAGNETIC EFFECTS OF CURRENT AND MAGNETISMEXERCISE - Numerical MCQ61videos
MAGNETIC EFFECTS OF CURRENT AND MAGNETISM
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - Kinetic Theory of Gases and Gas LawsEXERCISE - Unsolved Numerical Problems for Preparation of NSEP, INPhO & IPhO64videos
Kinetic Theory of Gases and Gas Laws
- BOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - NUCLEAR PHYSICS AND RADIOACTIVITYEXERCISE - Concptual MCQs48videos
NUCLEAR PHYSICS AND RADIOACTIVITY
Similar Questions
A wire forming one cycle of sine curve is moved in x-y plane with velocity →V=Vxˆi+Vyˆj. There exist a magnetic field →B=−B0ˆk.
Find the motional emf decelop across the ends PQ of wire.
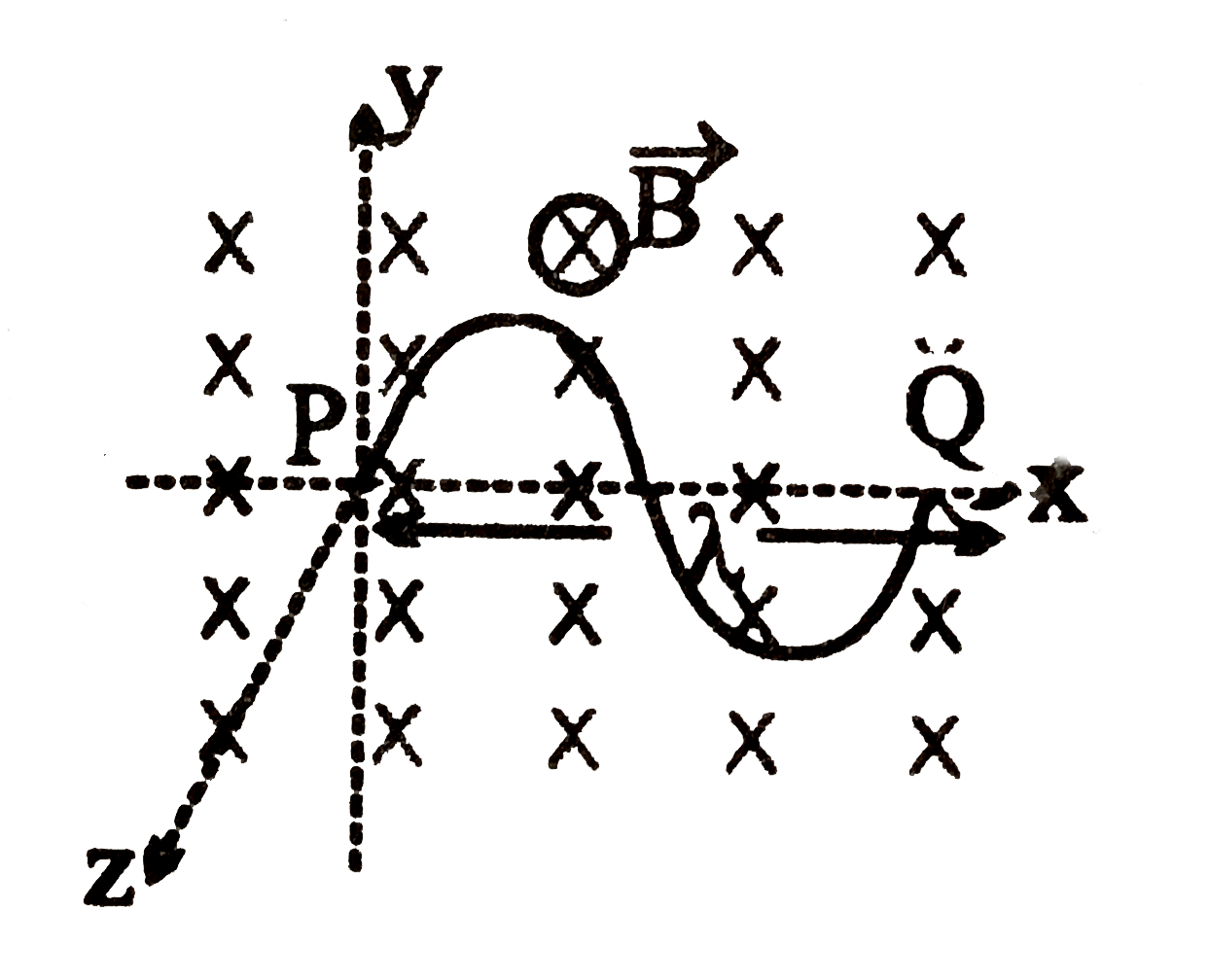 View Solution
View SolutionAn alpha particle moving with the velocity →v=uˆi+ˆj ,in a uniform magnetic field →B=Bˆk . Magnetic force on alpha particle is
View Solution
Knowledge Check
- Question 1 - Select One
A charged particle of specific charge (charge/mass) α released from origin at time t=0 with velocity →v=v0(ˆi+ˆj) in uniform magnetic field →B=B0ˆi. Coordinates of the particle at time t=π/(B0α) are
A(v02B0α,√2v0αB0,−v0B0α)B(v02B0α,0,0)C(0,2v0B0α,v0π2B0α)D(v0πB0α,0,−2v0B0α) - Question 2 - Select One
In uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Axis of helix is alongAX-axisBY-axisCNegative Y-axisDZ-axis - Question 3 - Select One
In uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Pitch of helical path described by particle isA2mv0qB0B2πmv0qB0C√2πmv0qB0D√3πmv0qB0
A particle of charge q and mass m released from origin with velocity →v=v0ˆi into a region of uniform electric and magnetic fields parallel to y-axis. i.e., →E=E0ˆjand→B=B0ˆj. Find out the position of the particle as a functions of time
Strategy : Here →E∣∣→B
The electric field accelerates the particle in y-direction i.e., component of velocity goes on increasing with acceleration
ay=Fym=Fem=qE0m
The magnetic field rotates the particle in a circle in x-z plane (perpendicular to magnetic field) The resultant path of the particle is a helix with increasing pitch.
Velocity of the particle at time t would be
→v(t)=vxˆi+vyˆj+vzˆkView SolutionIn uniform magnetic field, if angle between →vand→Bis0∘<0<90∘, the path of particle is helix. Let v1 be the component of →valong→Bandv2 be the component perpendicular to →B. Suppose p is the pitch. T is the time period and r is the radius of helix. Then
T=2πmqB,r=mv2qB,P=(v1)T
Assume a charged particle of charge q and mass m is released from the origin with velocity →v=v0ˆi−v0ˆk in a uniform magnetic field →B=−B0ˆk.
Angle between v and B isView SolutionA portion is fired from origin with velocity →v=v0ˆj+v0ˆk in a uniform magnetic field →B=B0ˆj. In the subsequent motion of the proton
View SolutionA particle of specific charge q/m=(π)C/kg is projected from the origin towards positive x-axis with a velocity of 10m/s in a uniform magnetic field →B=−2ˆK Tesla. The velocity →V of the particle after time t=1/6 s will be
View SolutionA particle of charge per unit mass α is released from origin with velocity →v=v0ˆi in a magnetic field
→B=−B0ˆk for x≤√32v0B0α
and →B=0 for x>√32v0B0α
The x-coordinate of the particle at time t(>π3B0α) would beView Solution

