A particle will leave a vertical circle of radius r when its velocity at the lowest point of the circle (upsilon L) is .
√2gr
√5gr
√3 g r
√6gr
The correct Answer is:c
To determine the velocity at the lowest point of a vertical circle that will cause a particle to leave the circle, we can follow these steps:
1. Understand the Problem:
- We have a particle moving in a vertical circle of radius r.
- We need to find the minimum velocity at the lowest point (point A) such that the particle will leave the circular path.
2. Identify Key Points:
- The lowest point of the circle is point A.
- The highest point of the circle is point B.
- The particle will leave the circle if it does not have enough velocity to reach the highest point.
3. Velocity at the Highest Point:
- For a particle to just reach the highest point (point B) of the vertical circle, it must have a minimum velocity vB at that point.
- At the highest point, the centripetal force required to keep the particle in circular motion is provided by the weight of the particle. Therefore:
mv2Br=mg
- Simplifying this gives:
v2B=gr⇒vB=√gr
4. Energy Conservation:
- We can use the conservation of mechanical energy to find the velocity at the lowest point (point A).
- The total mechanical energy at the lowest point (potential energy + kinetic energy) must equal the total mechanical energy at the highest point.
- At the lowest point (point A):
- Potential energy UA=0 (taking this as the reference point)
- Kinetic energy KA=12mv2A
- At the highest point (point B):
- Potential energy UB=mg(2r) (height is 2r)
- Kinetic energy KB=12mv2B
5. Setting Up the Energy Equation:
- Using conservation of energy:
KA+UA=KB+UB
12mv2A+0=12mv2B+mg(2r)
6. Substituting for vB:
- Substitute vB=√gr into the energy equation:
12mv2A=12m(gr)+mg(2r)
12mv2A=12mgr+2mgr
12mv2A=12mgr+42mgr=52mgr
7. Solving for vA:
- Multiply both sides by 2:
mv2A=5mgr
- Divide by m:
v2A=5gr
- Taking the square root:
vA=√5gr
8. Conclusion:
- The minimum velocity at the lowest point for the particle to leave the vertical circle is:
vA=√5gr
Final Answer:
The particle will leave the vertical circle when its velocity at the lowest point is √5gr.
- We have a particle moving in a vertical circle of radius r.
- We need to find the minimum velocity at the lowest point (point A) such that the particle will leave the circular path.
- The lowest point of the circle is point A.
- The highest point of the circle is point B.
- The particle will leave the circle if it does not have enough velocity to reach the highest point.
- For a particle to just reach the highest point (point B) of the vertical circle, it must have a minimum velocity vB at that point.
- At the highest point, the centripetal force required to keep the particle in circular motion is provided by the weight of the particle. Therefore:
mv2Br=mg
- Simplifying this gives:
v2B=gr⇒vB=√gr
- We can use the conservation of mechanical energy to find the velocity at the lowest point (point A).
- The total mechanical energy at the lowest point (potential energy + kinetic energy) must equal the total mechanical energy at the highest point.
- At the lowest point (point A):
- Potential energy UA=0 (taking this as the reference point)
- Kinetic energy KA=12mv2A
- At the highest point (point B):
- Potential energy UB=mg(2r) (height is 2r)
- Kinetic energy KB=12mv2B
- Using conservation of energy:
KA+UA=KB+UB
12mv2A+0=12mv2B+mg(2r)
- Substitute vB=√gr into the energy equation:
12mv2A=12m(gr)+mg(2r)
12mv2A=12mgr+2mgr
12mv2A=12mgr+42mgr=52mgr
- Multiply both sides by 2:
mv2A=5mgr
- Divide by m:
v2A=5gr
- Taking the square root:
vA=√5gr
- The minimum velocity at the lowest point for the particle to leave the vertical circle is:
vA=√5gr
The particle will leave the vertical circle when its velocity at the lowest point is √5gr.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Fill in the blanks
20 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Problems for practice
112 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Value Based Questions with Answers
5 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
A particle is projected along the inner surface of a smooth vertical circle of radius R, its velocity at the lowest point being (1/5)(√95gR). If the particle leaves the circle at an angular distance cos−1(x/5) from the highest point, the value of x is.
View SolutionThe linear speed of a particle revolved in a vertical circle of radius r is √7gr at the lowest point. What is the linear speed half way to the top?
View Solution
Knowledge Check
A fighter plane is moving in a vertical circle of radius 'r'. Its minimum velocity at the highest point of the circle will be
A√3grB√2grC√grD√gr/2A body of mass m tied to a string is moved in a vertical circle of radius r. The difference in tensions at the lowest point and the highest point is
A2mgB6mgC4mgD8mgThe velocity of a body moving in a vertical circle of radius r is √7gr at the lowest point of the circle. What is the ratio of maximum and minimum radius r is tension?
A4:1B√7:1C3:1D2:1
A stone of mass m tied to one end of a string is revolved in a vertical circle of radius R the net forces at the lowest (FL and highest (FH) points of the circle directed vertically downwards are :
(a) FL=mg−T1FH=mg+T2
(b) FL=mg+T1,FH=mg−T2
(c) FL=mg+T1−mυ21R,FH=mg−T2+mυ21R
(d) FL=mg−T1−mυ21R,FH=mg+T2+mυ12R
Choose corret alternative T1,υ1 denote the tension and speed at the lowest point T2,υ2 denote corresponding valuse at the highest point .
View SolutionA bob is attached to a string of length l whose other end is fixed and is given horizontal velocity at the lowest point of the circle so that the bob moves in a vertical plane. Match the velocity given at the lowest point of circle in column I with tension and velocity at the highest point of the circle corresponding to velocity of column I of column II
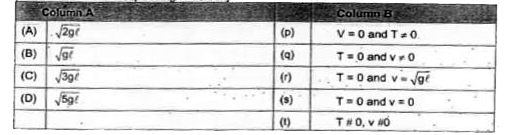
View SolutionThe velocity of a body moving in a vertical circle of radius r is √7gr at the lowest point of the circle. What is the ratio of maximum and minimum tension ?
View SolutionA particle of mass m attached to an inextensible light string is moving in a vertical circle of radius r. The critical velocity at the highest point is v0 to complete the vertical circle. The tension in the string when it becomes horizontal is
View SolutionA main revolves a stone of mass m tied to the end of a string in a circle of radius R The net force at the lowest and height point of the circle directed vertical downward are
Here T1,T2 and (v1,v2) denote the tension in the string (and the speed of the stone) at the lowest and highest points, respectively.
View Solution