A person holds a spring balance with a mass m hanging from it goes up and up in a helicopter, then reading of weight of body as indicated by spring balance will.
be increasing
be decreasing
first increase and then decrease
remain the same.
The correct Answer is:D
To solve the problem of how the reading of the spring balance changes when a mass m is hanging from it in a helicopter that is ascending, we can analyze the forces acting on the mass and apply Newton's laws of motion.
1. Identify the Forces Acting on the Mass:
- The mass m experiences two forces:
- The gravitational force acting downward, which is \( F_g = mg \) (where \( g \) is the acceleration due to gravity).
- The tension \( T \) in the spring balance acting upward.
2. Consider the Acceleration of the Helicopter:
- When the helicopter ascends, it accelerates upward. Let’s denote the upward acceleration of the helicopter as \( a \).
3. Apply Newton's Second Law:
- According to Newton's second law, the net force acting on the mass is equal to the mass times its acceleration.
- The net force \( F_{net} \) acting on the mass can be expressed as:
\(
F_{net} = T - mg
\)
- Since the helicopter is accelerating upward, the net force is also given by:
\(
F_{net} = ma
\)
4. Set Up the Equation:
- Setting the two expressions for net force equal gives:
\(
T - mg = ma
\)
5. Solve for Tension (T):
- Rearranging the equation to solve for the tension \( T \):
\(
T = mg + ma
\)
- This can be factored as:
\(
T = m(g + a)
\)
6. Interpret the Result:
- The reading on the spring balance (which measures the tension) will be \( T = m(g + a) \).
- This indicates that the reading of the weight of the body as indicated by the spring balance will increase when the helicopter ascends, as it is the sum of the gravitational force and the additional force due to the upward acceleration.
Final Answer:
The reading of the weight of the body as indicated by the spring balance will increase and be equal to \( m(g + a) \).
- The mass m experiences two forces:
- The gravitational force acting downward, which is \( F_g = mg \) (where \( g \) is the acceleration due to gravity).
- The tension \( T \) in the spring balance acting upward.
- When the helicopter ascends, it accelerates upward. Let’s denote the upward acceleration of the helicopter as \( a \).
- According to Newton's second law, the net force acting on the mass is equal to the mass times its acceleration.
- The net force \( F_{net} \) acting on the mass can be expressed as:
\(
F_{net} = T - mg
\)
- Since the helicopter is accelerating upward, the net force is also given by:
\(
F_{net} = ma
\)
- Setting the two expressions for net force equal gives:
\(
T - mg = ma
\)
- Rearranging the equation to solve for the tension \( T \):
\(
T = mg + ma
\)
- This can be factored as:
\(
T = m(g + a)
\)
- The reading on the spring balance (which measures the tension) will be \( T = m(g + a) \).
- This indicates that the reading of the weight of the body as indicated by the spring balance will increase when the helicopter ascends, as it is the sum of the gravitational force and the additional force due to the upward acceleration.
The reading of the weight of the body as indicated by the spring balance will increase and be equal to \( m(g + a) \).
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - AIIMS Questions
26 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
The Earth is not a perfect sphere. Due to the flattening of Earth at the poles, the radius of Earth is minimum at the poles and hence the value of g is maximum at the poles. One the other hand, the radius of the Earth is maximum at the equator of the Earth. As the mass and radius of moon are smaller than that of the Earth, so the value of g on moon is 1.63m/s2 . As we go up from the surface of the Earth, the distance from the centre of the Earth increases and hence the value of g decreases. The value of g decreases as we go down inside the Earth and it become zero at the centre of the Earth .
If a person with a spring balance and a body hanging from it goes up and up in an aeroplane, then the reading of the weight of the body as indicated by the spring balance will beAincreasesBdecreasesCremain sameDfirst increases and then decreasesThe readings of the spring balance will be :
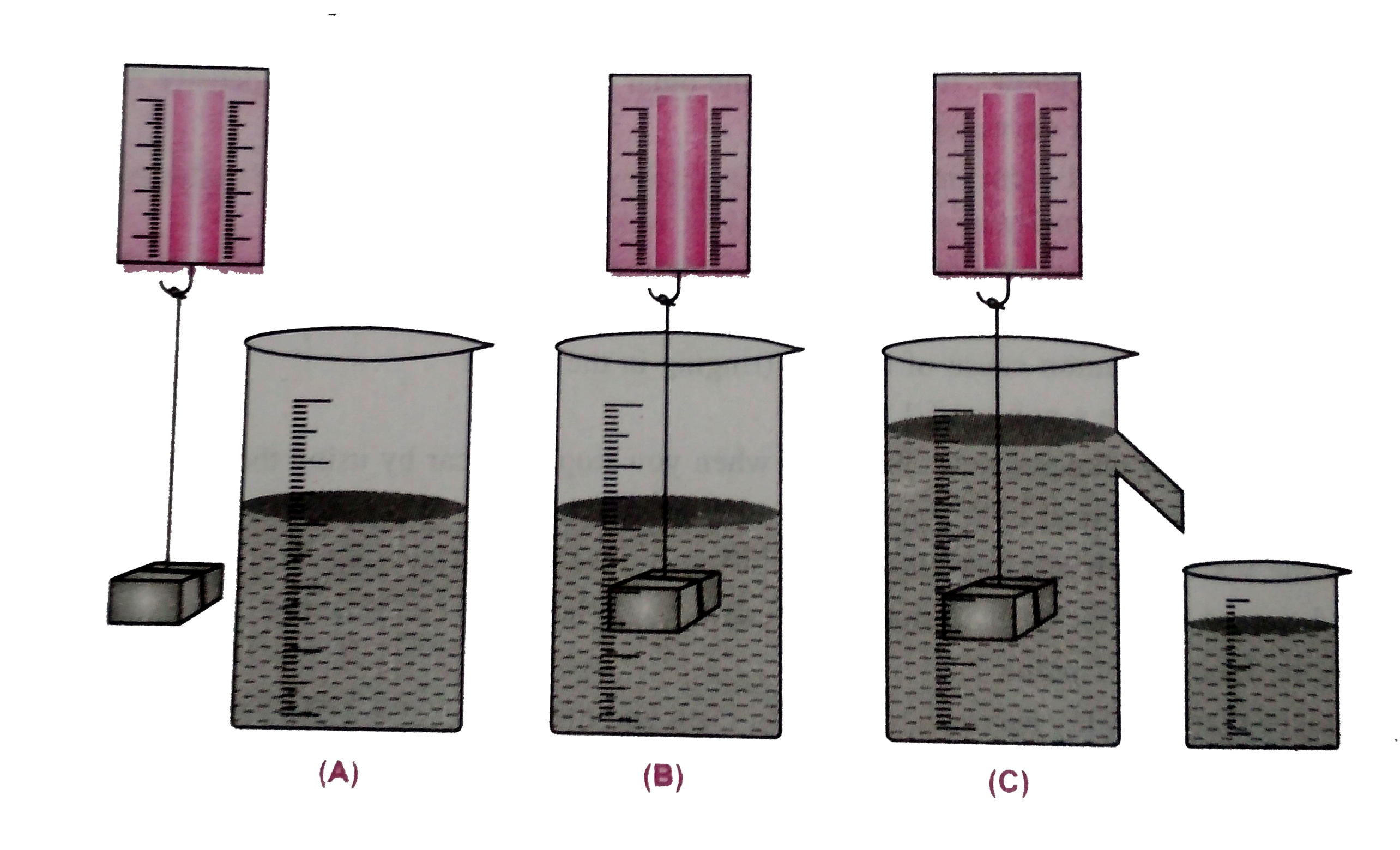 Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case.
Aequal to each other in all cases A,BandC.Bequal to each other in cases AandC only.Cequal to each other in cases BandC only.Ddifferent in every case.The spring balance inside a lift suspends an object. As the lift begins to ascent, the reading indicated by the spring balance will
AIncreaseBDecreaseCRemain unchangedDDepend on the speed of ascend
An object weights W newton on earth. It is suspended from the lower end of a spring balance whose upper end is fixed to the ceiling of a space capsule in a stable orbit around the earth. The reading of the spring balance will be
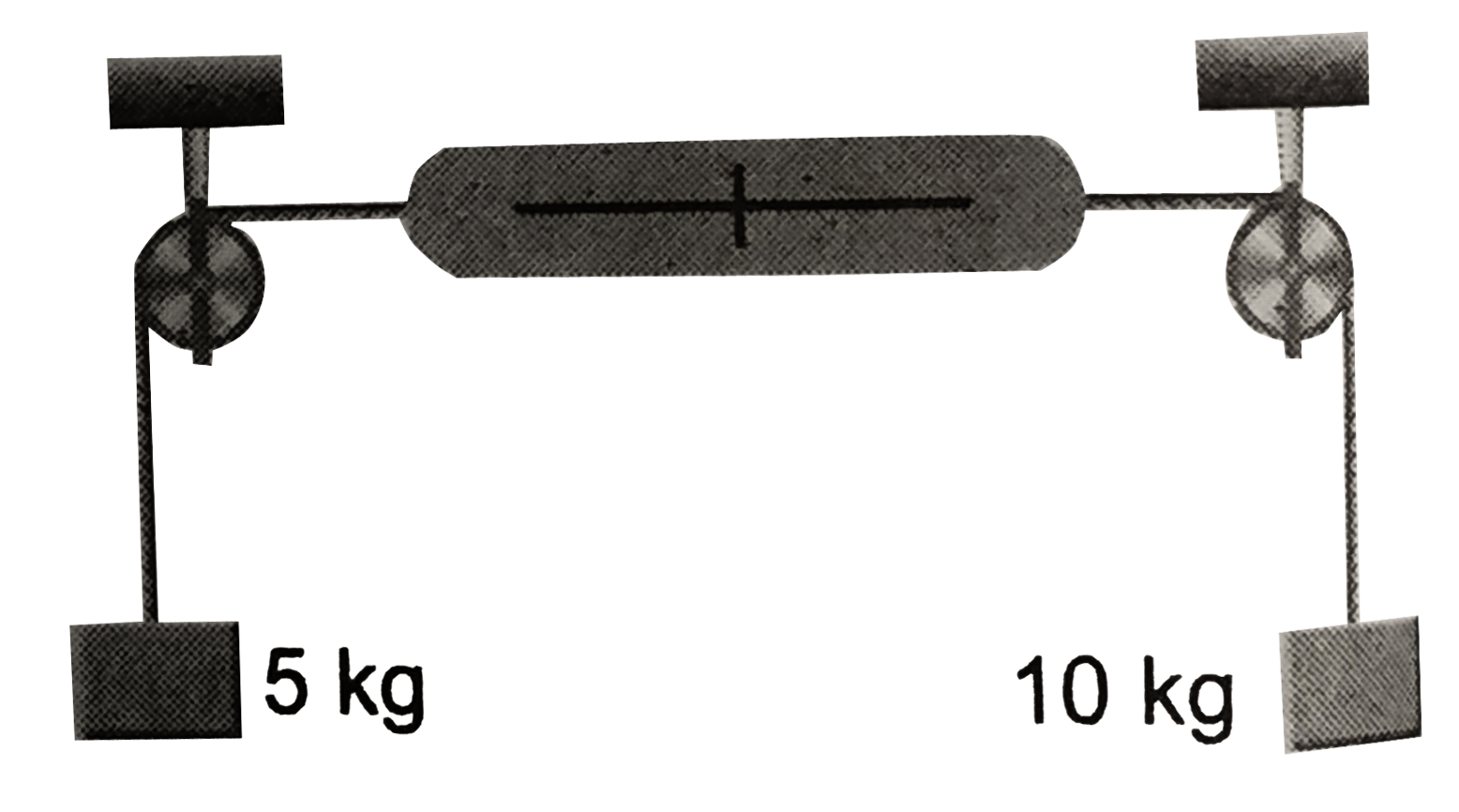
View SolutionFor the arrangement shown in the figure, the reading of spring balance is
View SolutionA mass is hanging on a spring balance which is kept in a lift. The lift ascends. The spring balance will show in its reading
View SolutionAn air-tight cage with a parrot sitting in it is suspended from the spring balance. The parrot starts flying. The reading of the spring balance will
View SolutionAn air tight cage with are parrot sitting in it is suspended from the spring balance. The parrot starts flying. The reading of the spring balance will
View Solution

