A person stands on a spring balance at the equator.
(a) How much is the reduction in weight as measured by the balance.
(b) If the speed of earth's rotation is changed such that the balance reading is half of its true weight, what will be the length of the day in this case?
Step by step video & image solution for A person stands on a spring balance at the equator. (a) How much is the reduction in weight as measured by the balance. (b) If the speed of earth's rotation is changed such that the balance reading is half of its true weight, what will be the length of the day in this case? (g=10m//s^(2),R_(e)=6400km) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - Practice Exercise
42 Videos
- WORK, ENERGY AND POWERBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - WORK, ENERGY AND POWEREXERCISE - Discussion Question
31 Videos
- RIGID BODIES AND ROTATIONAL MOTIONBOOK - PHYSICS GALAXY - ASHISH ARORACHAPTER - RIGID BODIES AND ROTATIONAL MOTIONEXERCISE - Unsolved Numerical
63 Videos
Similar Questions
A person stands on a spring balance at the equator. a.By wht fraction is the balance reading less than his true weight? b.If the speed of earth's rotation is increased by such an amount that the balance reading is half the true weight, what will be the lenght o the day in this case?
View SolutionThe systems shown in are in equilibrium if the spring balance is calibrated in newtons, what does it record in each case? (g=10m/s2)
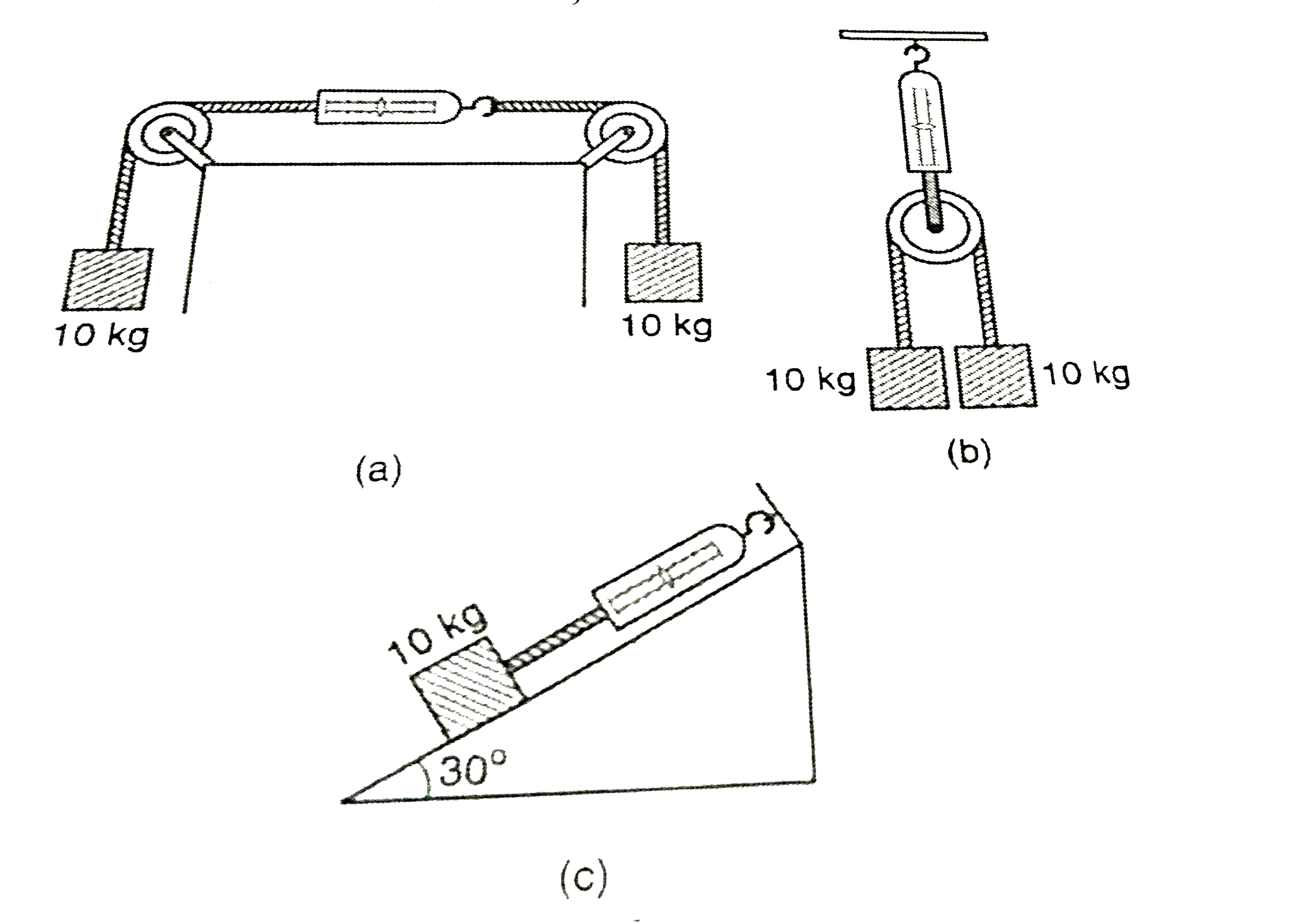
View Solution
Knowledge Check
A spring balance fastened to the roof of a lift accelerating upwards indicates 120N as the weight of a 80N body. The acceleration of the lift is (g=10m/s2).
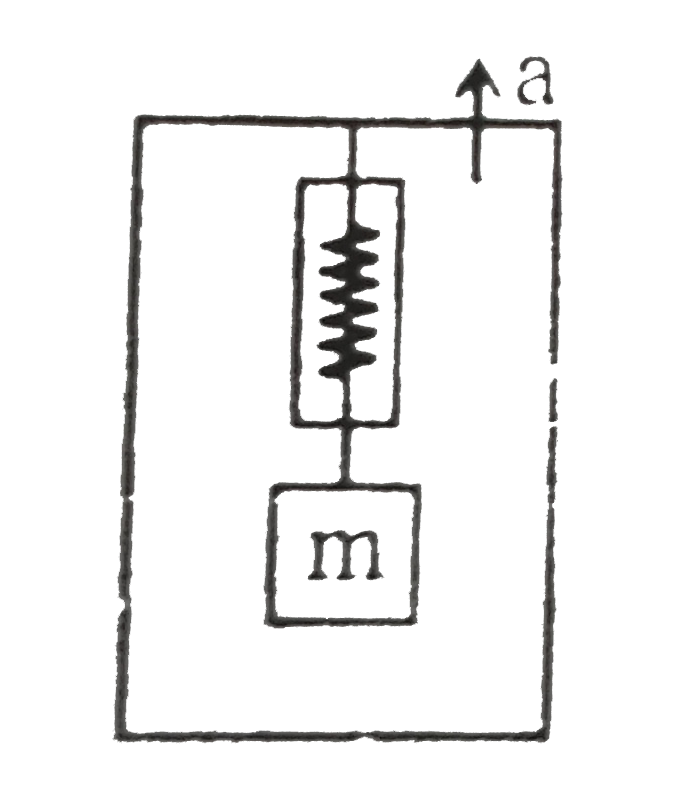 A5m/s2 upwardsB203m/s2 upwardsC103m/s2 upwardsD5m/s2 downwards
A5m/s2 upwardsB203m/s2 upwardsC103m/s2 upwardsD5m/s2 downwardsIf the earth has no rotational motion, the weight of a person on the equation is W. Detrmine the speed with which the earth would have to rotate about its axis so that the person at the equator will weight 34 W. Radius of the earth is 6400 km and g = 10 m/s2.
A0.63×10−3rad/sB0.28×10−3rad/sC1.1×10−3rad/sD0.83×10−3rad/sWhat should be the velocity of earth due to rotation about its own axis so that the weight at equator become 3/5 of initial value. Radius of earth on equator is 6400 km
A8.7×10−1 rad/sB7.8×10−4 rad/sC6.7×10−4 rad/sD7.4×10−3 rad/s
A body suspended on a spring balance in a ship weighs W0 when the ship is at rest. When the ship begins to move along the equator with a speed v, show that the scale reading is very close to W0(1±2ωV/g), where ω is the angular speed of the earth.
View SolutionA body weights 98 N on a spring balance at the north pole. What will be its weight recorded on the same scale if it is shifted to te equator? Use g=GMR2=9.8ms2 and the radius of the earth R=6400 km.
View SolutionHow many times faster than its present speed the earth should rotate so that the apparent weight of an object at equator becomes zero ? Given radius of the earth = 6.37×106 m . What would be the duration of the day in that case ?
View SolutionA body hangs from a spring balance supported from the roof of an elevator.
(a) If the elevator has an upward acceleration of 2.45ms−2 and the balance reads 50N, what is the true weight of the body?
(b) Under what circumstances will the balance read 30N?
(c) What will be the balance reading if the elevator, cable breaks?
View SolutionFigure shows a light spring balance connected to two blocks of mass 20 kg each. The graduations in the balance measure the tension in the spring. A. What is the reading of the balance? b.Will the reading change if the balance is heavy, say 2.0 kg? c. What will hapen if the spring is light but the blocks the unequal masses?

View Solution
