A person throws ball with velocity from the top of a building in vertically upward direction the ball reaches the ground with a speed of 3v then the height of the building is :Solution in Kannada
4v2g
3v2g
6v2g
9v2g
The correct Answer is:a
Let h be the height and x be the maximum height of the ball above the building to which it rise. Then we have
x=v22g and h+x=(3v)22g
∴H=9v22g−v22g=4v2g
Topper's Solved these Questions
- DESCRIPTION OF MOTION IN ONE DIMENSION BOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN ONE DIMENSION EXERCISE - MULTIPLE CHOICE QUESTION (LEVEL-II)
54 Videos
- DESCRIPTION OF MOTION IN ONE DIMENSION BOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN ONE DIMENSION EXERCISE - Recent Competitive Question
12 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - RECENT COMPETITIVE QUESTIONS
32 Videos
- DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONBOOK - MODERN PUBLICATIONCHAPTER - DESCRIPTION OF MOTION IN TWO AND THREE DIMENSIONEXERCISE - Revision TEST
36 Videos
Similar Questions
A man moves along the path abode as shown in the Fig. What is his displacement from the point of start?
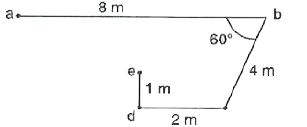
View SolutionA train is moving slowly on a straight track with a constant speed of 2 m s−1 . A passengers in the train starts walking at a stready speed of 2 m s−1 to the back of the train in the opposite direction of the motion of the train. So to an observer standing on the platform directly in front of that passenger, the velocity of the passenger appears to be
View SolutionA battery of emf E has an internal resistance r. A variable resistance R is connected to the terminals of the battery. A current i is drawn from the battery. V is the terminal potential difference. If R alone is gradually reduced to zero, which of the following best describes i and V?
View SolutionThe torque of a force →F=−3ˆi+ˆj+5ˆk acting at a point is →τ. If the position vector of the point vector of the point is 7ˆi+3ˆj+ˆk, then →τ is :
View SolutionA person standing near the edge of the top of a building throws two balls A and B. The ball A is thrown vertically upward and B is thrown vertically downward with the same speed. The ball A hits the ground with speed vA and the ball B hits the ground wiht a speed vB. We have
View SolutionBall A is dropped from the top of a building. At the same instant ball B is thrown vertically upwards from the ground. When the balls collide, they are moving in opposite direction and the speed of A is twice the speed of B. At what fraction of the height of the building did the collision occurs ?
View SolutionA ball is thrown vertically upwards with the speed of 19.6 ms^(-1) from the top of building and reaches the earth in 6 s. Find the height of the building .
View SolutionA ball A is dropped from the top of a building and at the same time an identical ball B is thrown vertically upward from the ground. When the balls collide the speed of A is twice that of B. At what fraction of the height of the building did the collison occur?
View SolutionA ball is thrown vertically upwards with a velocity of 20ms^-1 from the top of a multistorey building. The height of the point from where the ball is thrown is 25.0 m from the ground. How high will the ball rise?
View SolutionA ball is thrown vertically upwards from ground with such a speed so that it just reaches the top of a building and then falls back. If it takes 7s for the ball to return to the hands of thrower. Calculate the height of the building approximately.
View SolutionA person standing near the edge of the top of a building throws two balls A and B. The ball A is thrown vertically upward and B is thrown vertically downward with the same speed. The ball A hits the ground with speed vA and the ball B hits the ground wiht a speed vB. We have
View SolutionA ball A is dropped from the top of a building and at the same time an identical ball B is thrown vertically upward from the ground. When the balls collide the speed of A is twice that of B. At what fraction of the height of the building did the collison occur?
View SolutionBall A is dropped from the top of a building and at the same instant that a ball B is thrown vertically upward from the ground. When the balls collide, they are moving in opposite directions and the speed of A is twice the speed of B. At what fraction of the height of the building did the collision occur?
View SolutionBall A is dropped from the top of a building and at the same instant that a ball B is thrown vertically upward from the ground. When the balls collide, they are moving in opposite directions and the speed of A is twice the speed of B. At what fraction of the height of the building did the collision occur?
View SolutionBall A is dropped from the top of a building at the same instant that a ball B is thrown vertically upward from the ground . When the balls collide, they are moving in opposite directions and the speed of A is twice the speed of B . At what fraction of the height of the building did the collsion occur?
View Solution
