Question

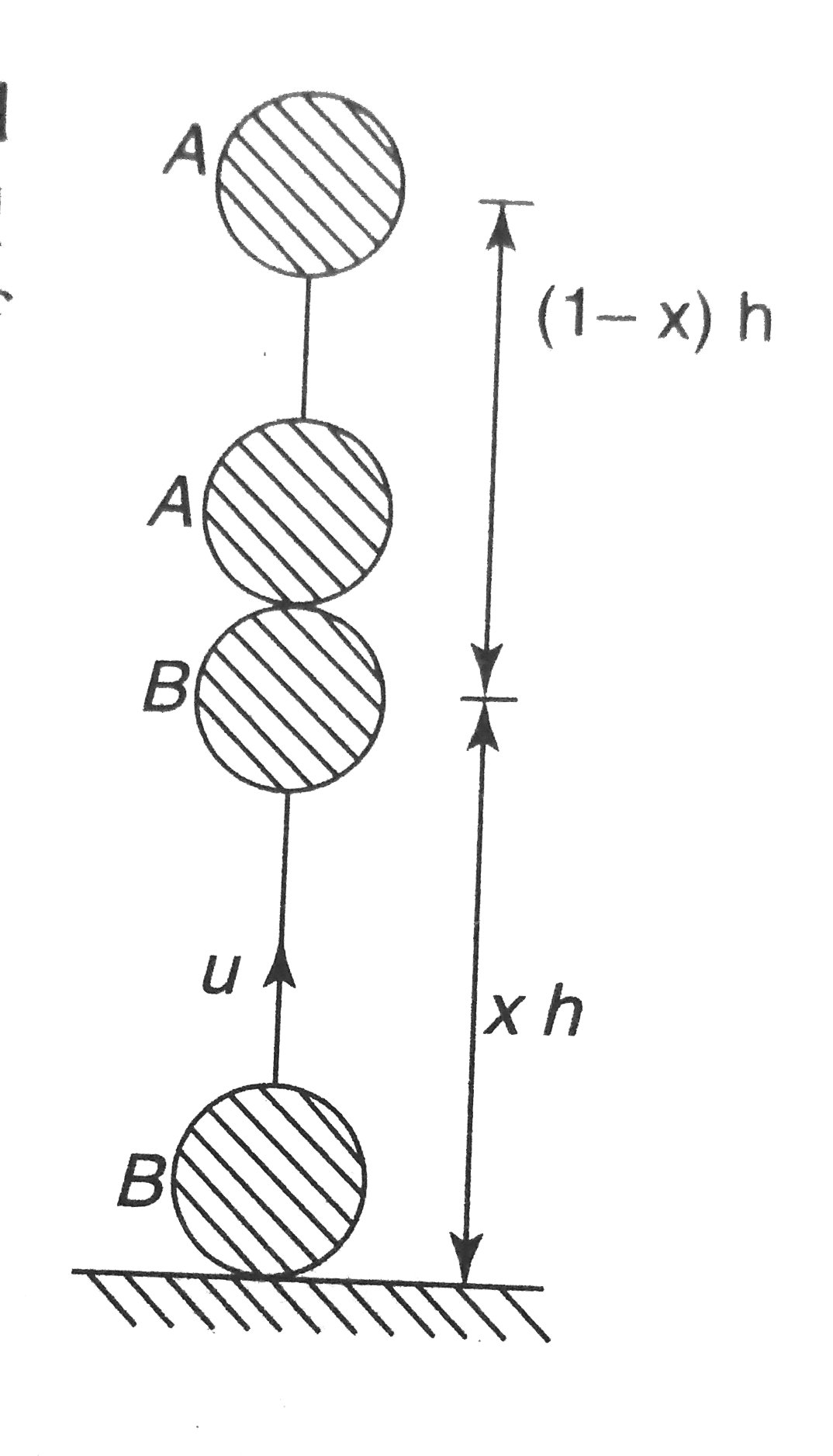
Ball $A$ is dropped from the top of a building. At the same instant ball $B$ is thrown vertically upwards from the ground. When the balls collide, they are moving in opposite direction and the speed of $A$ is twice the speed of $B$. At what fraction of the height of the building did the collision occurs ?

Options

A. `1//3`
B. `2//3`
C. `1//4`
D. `2//5`

Answer

B

Explanation

Let `h` be the total height and `x` the desired fraction. Initial velocity of ball `B` is `u` at time of collision it is `v_(B)`. Then
`(1 - x) h = (1)/(2) g t^2` …(1)
or `t = sqrt((2(1 - x)h)/(g))` …(2)
Also, `xh = ut - (1)/(2) g t^2`
or `xh=u sqrt(2(1 - x)h)/(g) - (1 - x)h`
or `u = sqrt((gh)/(2(1 - x)))`
Now `v_A = 2v_B` (at the time of collision)
or `v_A^2 = 4v_B^2`
:. `2g(1 - x) h = 4 {u^2 - 2gxh}`
or `2g(1 - x) h = 4 {(gh)/(2(2 - x))- 2gxh}`
or `(1 - x)=(1)/(1 - x) -4x`
or `1 + x^2 - 2x = 1 - 4x + 4x^2`
or `x = (2)/(3)`.