A pipe of length l1, closed at one end is kept in a chamber of gas of density ρ1. A second pipe open at both ends is placed in a second chamber of gas of density ρ2. The compressibility of both the gases is equal. Calculate the length of the second pipe if frquency of first overtone in both the cases is equal.
43l1√ρ2ρ1
43l1√ρ1ρ2
l1√ρ2ρ1
l1√ρ1ρ2
The correct Answer is:B
To solve the problem, we need to analyze the frequencies of the first overtone for both the closed and open pipes and set them equal to each other.
1. Understand the Frequencies:
- For a closed pipe (closed at one end), the frequency of the first overtone (n=2) is given by:
f1=34VL1
- For an open pipe (open at both ends), the frequency of the first overtone (n=3) is given by:
f2=4L2V
2. Identify the Speed of Sound:
- The speed of sound V in both gases can be expressed in terms of the compressibility β and density ρ:
V=√βρ
- Since the compressibility of both gases is equal, we can denote it as β.
3. Substituting the Speed of Sound:
- For the closed pipe:
f1=341L1√βρ1
- For the open pipe:
f2=4L2√βρ2
4. Setting Frequencies Equal:
- Since the frequencies of the first overtone are equal:
341L1√βρ1=4L2√βρ2
5. Canceling Common Terms:
- We can cancel √β from both sides:
341L1√ρ1=4L2√ρ2
6. Cross-Multiplying:
- Cross-multiplying gives:
3L2√ρ2=16L1√ρ1
7. Solving for L2:
- Rearranging the equation to find L2:
L2=16L1√ρ13√ρ2
Final Result:
The length of the second pipe L2 is given by:
L2=16L1√ρ13√ρ2
- For a closed pipe (closed at one end), the frequency of the first overtone (n=2) is given by:
f1=34VL1
- For an open pipe (open at both ends), the frequency of the first overtone (n=3) is given by:
f2=4L2V
- The speed of sound V in both gases can be expressed in terms of the compressibility β and density ρ:
V=√βρ
- Since the compressibility of both gases is equal, we can denote it as β.
- For the closed pipe:
f1=341L1√βρ1
- For the open pipe:
f2=4L2√βρ2
- Since the frequencies of the first overtone are equal:
341L1√βρ1=4L2√βρ2
- We can cancel √β from both sides:
341L1√ρ1=4L2√ρ2
- Cross-multiplying gives:
3L2√ρ2=16L1√ρ1
- Rearranging the equation to find L2:
L2=16L1√ρ13√ρ2
The length of the second pipe L2 is given by:
L2=16L1√ρ13√ρ2
Topper's Solved these Questions
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Multiple Correct
22 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - Comprehension
12 Videos
- ARCHIVES 2 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 2 VOLUME 6EXERCISE - True/False
4 Videos
- ARCHIVES 1 VOLUME 6BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ARCHIVES 1 VOLUME 6EXERCISE - Integer
4 Videos
- BASIC MATHEMATICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - BASIC MATHEMATICSEXERCISE - Exercise 2.6
20 Videos
Similar Questions
The second overtone of an open pipe has the same frequency as the first overtone of a closed pipe 2 m long. The length of the open pipe is
View SolutionA pipe 1 m length is closed at one end. Taking the speed of sound in air as 320ms−1 .the air column in the pipe cannot resonate for the frequency (in Hz )
View SolutionAn organ pipe open at one end is vibrating in first overtone and is in resonance with another pipe open at both ends and vibrating in third harmonic. The ratio of length of two pipes is–
View SolutionA pipe of length 1.5 m closed at one end is filled with a gas and it resonates at 30∘C in its fundamental with a tuning fork. Another pipe of the same length but open at both ends and filled with air and it resonates in its fundamental with the same tuning fork. Calculate the velocity of sound at 0∘C in the gas, given that the velocity of sound in air is 360ms−1 at 30∘.
View SolutionAn organ pipe A closed at one end vibrating in its fundamental frequency and another pipe B open at both ends is vibrating in its second overtone are in resonance with a given tuning fork . The ratiio of length of pipe A to that of B is
View SolutionCompare the length of a closed organ pipe and an open organ pipe, if the second overtone of the open pipe is unison with the second overtone of the closed organ pipe.
View SolutionA closed pipe of length 10 cm has its fundamental frequency half that of the second overtone of an open pipe . The length of the open pipe .
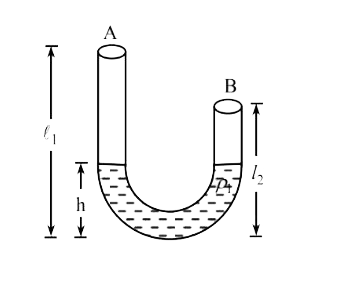
View SolutionA tube U-shaped has a uniform cross-sectionn with arm length l1andl2(l1>l2). Tube has a liquid of density ρ1 filled to a height h. another liquid of density ρ2=(ρ1)14 is poured in arm A. both liquids are immiscible. the length of second liquid that should be poured in A so that first overtone of A is in unisom with fundamental tone of B is:
View SolutionTwo open organ pipes of lengths 60 cm and 60.5 cm produce 2 beats per second. Calculate the velocity of sound in air.?
View SolutionTwo narrow cylindrical pipes AandB have the same length. Pipe A is open at both ends and is filled with a monoatomic gas of molar mass MA. Pipe B is open at one end and closed at the other end, and is filled with a diatomic gas of molar mass MB. Both gases are at the same temperature.
(a) If the frequency of the second harmonic of the fundamental mode in pipe A is equal to the frequency of the third harmonic of the fundamental mode in pipe B, determine the value of MB/MB.
(b) Now the open end of pipe B is also closed (so that the pipe is closed at both ends). Find the ratio of the fundamental frequency in pipe A to that in pipe B.
View Solution