A point charge q is placed at a distance of r from cebtre of an uncharged conducting sphere of rad R(<r). The potential at any point on the sphere is
a. zero
b. 14πε0qr
c.14πε0qRr2
d. 14πε0qr2R
The correct Answer is:B
To solve the problem, we need to find the potential at any point on the surface of an uncharged conducting sphere when a point charge q is placed at a distance r from the center of the sphere, where R<r.
1. Understanding the System:
- We have a point charge q located at a distance r from the center of an uncharged conducting sphere of radius R.
- Since R<r, the point charge is outside the conducting sphere.
2. Properties of Conductors:
- In electrostatics, the electric field inside a conductor in electrostatic equilibrium is zero.
- The potential throughout the conducting material and on its surface is constant.
3. Calculating the Potential at the Surface of the Sphere:
- The potential V at any point due to a point charge q at a distance r is given by the formula:
V=q4πϵ0r
- Here, r is the distance from the charge to the point where we are calculating the potential.
4. Applying the Formula:
- For any point on the surface of the conducting sphere (which is at a distance R from the center), the potential V can be calculated as:
V=q4πϵ0r
- Since the point charge is outside the sphere, the potential at the surface of the sphere is the same as the potential at the location of the charge q at distance r.
5. Conclusion:
- Therefore, the potential at any point on the surface of the sphere is:
V=q4πϵ0r
Final Answer:
The potential at any point on the sphere is q4πϵ0r.
- We have a point charge q located at a distance r from the center of an uncharged conducting sphere of radius R.
- Since R<r, the point charge is outside the conducting sphere.
- In electrostatics, the electric field inside a conductor in electrostatic equilibrium is zero.
- The potential throughout the conducting material and on its surface is constant.
- The potential V at any point due to a point charge q at a distance r is given by the formula:
V=q4πϵ0r
- Here, r is the distance from the charge to the point where we are calculating the potential.
- For any point on the surface of the conducting sphere (which is at a distance R from the center), the potential V can be calculated as:
V=q4πϵ0r
- Since the point charge is outside the sphere, the potential at the surface of the sphere is the same as the potential at the location of the charge q at distance r.
- Therefore, the potential at any point on the surface of the sphere is:
V=q4πϵ0r
The potential at any point on the sphere is q4πϵ0r.
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced
44 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Comprehension
36 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
Similar Questions
A point charge Q is situated (outside) at a distance r from the centre of an uncharged conducting solid sphere of radius R. The potential due to induced charges (on the conductor) at a point B inside the conductor whose distance form the point charge is d is ______
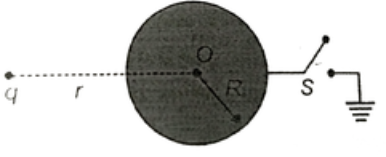
View SolutionA point charge q , is placed at a distance r from the centre of an aluminium sphere, of radius R (r>R) . Selectr the correct alternatives.
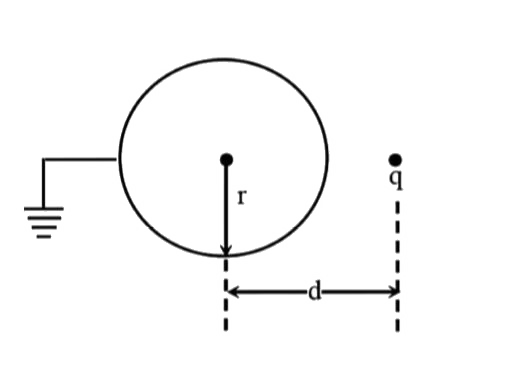
View SolutionA point charge q is placed at some distance d away from the centre of a grounded conducting sphere of radius r, as shown in the figure. The charge that flows the earth to the sphere is
View Solution
A point charge q'q is placed at distance 'a' from the centre of an uncharged thin spherical conducting shell of radius R=2a. A point 'P' is located at a distance 4a from the centre of the conducting shell as shown. The electric potential due to induced charge on the inner surface of the conducting shell at point 'P' is
View SolutionA charge q is placed at a distance of 2R from the centre of a spherical conducting shell of radius R. What is electrical potential, due to charges induced on the shell, at a point which is farthest from the point charge ?
View SolutionA point charge q is placed at a distance of r from the centre O of an uncharged spherical shell of inner radius R and outer radius 2R. The electric potential at the centre of the shell will be

View SolutionA point charge q is placed at a distance of r from the centre O of an uncharged spherical shell of inner radius R and outer radius 2R.The distance r<R.The electric potential at the centre of the shell will be

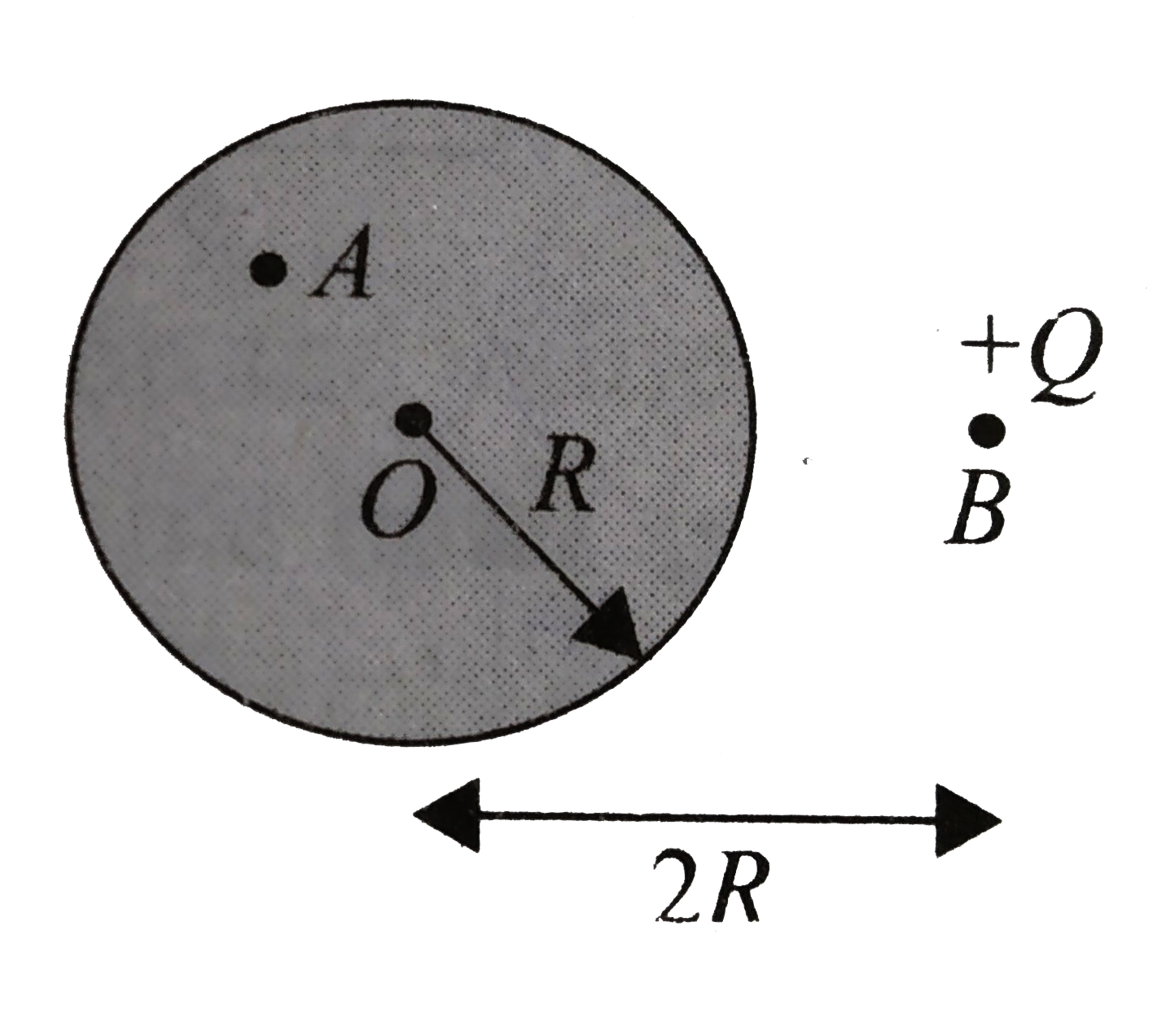
View SolutionA point charge +Q is placed at point B at a distance 2R from the center O of an uncharged thin conducting shell of radius R as shown in figure. If VA be the potential at point A, which is at a radial distance of R/2 from the center of the shell, then
View SolutionA solid conducting sphere of radius 'a' is surrounded by a thin uncharged concentric conducting shell of radius 2a. A point charge q is placed at a distance 4a from common centre of conducting sphere and shell. The inner sphere is then grounded
The potential of outer shell is
View SolutionA solid conducting sphere of radius 'a' is surrounded by a thin uncharged concentric conducting shell of radius 2a. A point charge q is placed at a distance 4a from common centre of conducting sphere and shell. The inner sphere is then grounded
The charge on solid sphere is
View Solution
