A solid conducting sphere of radius 'a' is surrounded by a thin uncharged concentric conducting shell of radius 2a. A point charge q is placed at a distance 4a from common centre of conducting sphere and shell. The inner sphere is then grounded
The potential of outer shell is
q32πε0a
q16πε0a
q8πε0a
q4πε0a
The correct Answer is:A
To solve the problem of finding the potential on the outer shell of a system consisting of a solid conducting sphere, a concentric conducting shell, and a point charge, we can follow these steps:
1. Understand the Configuration:
- We have a solid conducting sphere of radius a.
- This sphere is surrounded by a thin uncharged concentric conducting shell of radius 2a.
- A point charge q is placed at a distance 4a from the common center of the conducting sphere and shell.
- The inner sphere is grounded.
2. Identify the Effects of Grounding:
- Grounding the inner conducting sphere means that its potential is set to zero. This will influence the charge distribution on the sphere.
3. Calculate the Potential at the Surface of the Inner Sphere:
- The potential V at the surface of the inner sphere due to the point charge q is given by:
V=14πϵ0⋅q4a+Q1a
where Q1 is the induced charge on the inner surface of the conducting sphere.
4. Set the Potential to Zero:
- Since the inner sphere is grounded, we set the total potential to zero:
0=14πϵ0⋅q4a+Q1a
- Rearranging gives:
Q1=−q4
5. Determine the Charge on the Outer Shell:
- By conservation of charge, the total charge on the outer shell must be equal and opposite to the charge on the inner surface. Therefore, the charge on the outer surface of the shell is:
Qouter=+q4
6. Calculate the Potential on the Outer Shell:
- The potential V0 at the outer shell (radius 2a) is due to the point charge q and the charge on the outer shell:
V0=14πϵ0⋅q4a+14πϵ0⋅Qouter2a
- Substituting Qouter=q4:
V0=14πϵ0⋅q4a+14πϵ0⋅q42a
- Simplifying:
V0=14πϵ0(q4a+q8a)
V0=14πϵ0⋅2q+q8a=3q8πϵ0a
7. Final Expression for Potential:
- Therefore, the potential at the outer surface of the conducting shell is:
V0=3q8πϵ0a
- We have a solid conducting sphere of radius a.
- This sphere is surrounded by a thin uncharged concentric conducting shell of radius 2a.
- A point charge q is placed at a distance 4a from the common center of the conducting sphere and shell.
- The inner sphere is grounded.
- Grounding the inner conducting sphere means that its potential is set to zero. This will influence the charge distribution on the sphere.
- The potential V at the surface of the inner sphere due to the point charge q is given by:
V=14πϵ0⋅q4a+Q1a
where Q1 is the induced charge on the inner surface of the conducting sphere.
- Since the inner sphere is grounded, we set the total potential to zero:
0=14πϵ0⋅q4a+Q1a
- Rearranging gives:
Q1=−q4
- By conservation of charge, the total charge on the outer shell must be equal and opposite to the charge on the inner surface. Therefore, the charge on the outer surface of the shell is:
Qouter=+q4
- The potential V0 at the outer shell (radius 2a) is due to the point charge q and the charge on the outer shell:
V0=14πϵ0⋅q4a+14πϵ0⋅Qouter2a
- Substituting Qouter=q4:
V0=14πϵ0⋅q4a+14πϵ0⋅q42a
- Simplifying:
V0=14πϵ0(q4a+q8a)
V0=14πϵ0⋅2q+q8a=3q8πϵ0a
- Therefore, the potential at the outer surface of the conducting shell is:
V0=3q8πϵ0a
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Matrix Matching
2 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - Integer
17 Videos
- ELECTROSTATICSBOOK - DC PANDEY ENGLISHCHAPTER - ELECTROSTATICSEXERCISE - JEE Advanced
44 Videos
- ELASTICITYBOOK - DC PANDEY ENGLISHCHAPTER - ELASTICITYEXERCISE - Medical entrances s gallery
21 Videos
- EXPERIMENTSBOOK - DC PANDEY ENGLISHCHAPTER - EXPERIMENTSEXERCISE - Subjective
15 Videos
Similar Questions
A solid conducting sphere of radius 'a' is surrounded by a thin uncharged concentric conducting shell of radius 2a. A point charge q is placed at a distance 4a from common centre of conducting sphere and shell. The inner sphere is then grounded
The charge on solid sphere is
View SolutionA solid conducting sphere of radius 'a' is surrounded by a thin uncharged concentric conducting shell of radius 2a. A point charge q is placed at a distance 4a from common centre of conducting sphere and shell. The inner sphere is then grounded
Pick up the correct statement.
View SolutionA conducting sphere of radius R, carrying charge Q, lies inside uncharged conducting shell of radius 2R. If they are joined by a metal wire,
View SolutionInside a charged thin conducting shell of radius R, a point charge +Q is placed as shown in figure potential of shell may be
View SolutionA point charge q is placed at a distance of r from cebtre of an uncharged conducting sphere of rad R(<r). The potential at any point on the sphere is
View Solution
A point charge q'q is placed at distance 'a' from the centre of an uncharged thin spherical conducting shell of radius R=2a. A point 'P' is located at a distance 4a from the centre of the conducting shell as shown. The electric potential due to induced charge on the inner surface of the conducting shell at point 'P' is
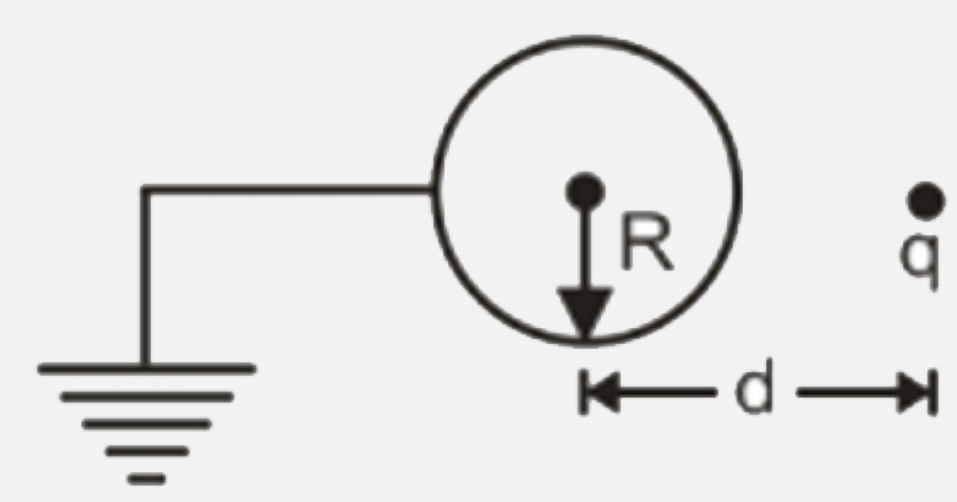
View SolutionIn the shown figure, the charge appearing on the conducting shell (grounded) of Radius R, when a point charge q is kept at a distance of d from the centre of the sphere is:
View SolutionA point charge q is placed at a distance of r from the centre O of an uncharged spherical shell of inner radius R and outer radius 2R. The electric potential at the centre of the shell will be

View SolutionA common test to find the genotype of a hybrid is by
View SolutionA conducting sphere of radius R and carrying a charge Q is joined to an uncharged conducting sphere of radius 2R. The charge flowing between them will be
View Solution
