(a) Pressure decreases as one ascends the atmosphere. If the density of air is ρ, What is the change in pressure dp over a differential height dh ? (b) Considering the pressure p to be proportional to the density, find the pressure p at a height h if the pressure on the sureface of the earth is p0. (c ) If p0=1.03×105Nm−2,ρ0=1.29kgm−3andg=9.8ms−2, at what height will the pressure drop to (1/10) the value at the surface of the earth ? (d) This model of the atmosphere works for relatively small distance. Identify the underlying assumption that limits the model.
Consider a horizontal parcel of air with cross-section A and height dh.
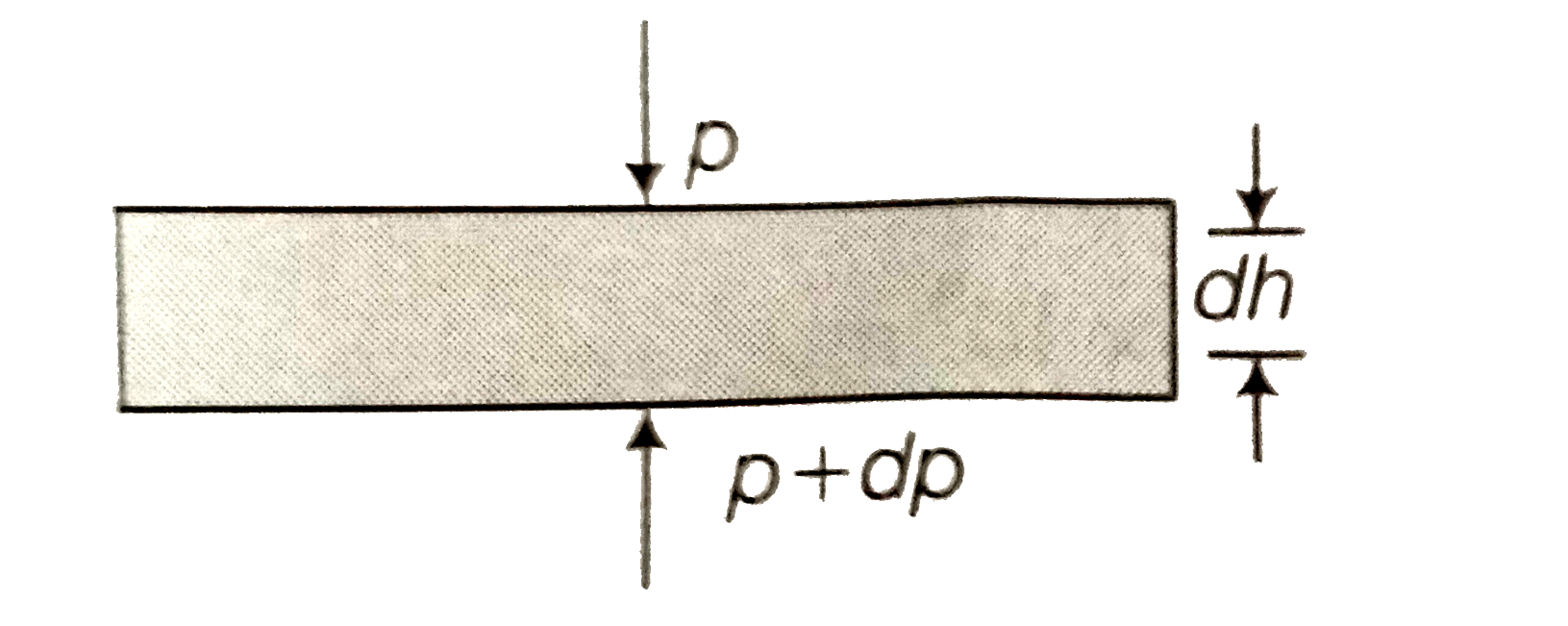
Let the pressure on the top surface and bottom surface be p and p+ dp. If the parcel is in equilibrium , then the net upward force must be balanced by the weight.
i.e., (p+dp)A−pA=−ρgAdh (∵ weight =Density × Volume ×g)
=−ρ×Adh×g
⇒dp=−ρgdh. (ρ= density of air )
Negative sign shows that pressure decreases with height .
(b) Let p∘ be the density of air on the surface of the earth.
As per question, pressure ∝ density
⇒pp∘=ρρ∘
⇒ρ=ρ∘p∘pdh [∵dp=−ρgdh]
⇒dpp=−ρ∘gp∘dh
⇒p∫p∘dpp=−ρ∘gp∘h∫0dh [∵at h=0r=p∘and at h=hp=p]
⇒Inpp∘=−ρ∘gp∘h
By removing log, p=p∘e(−ρ∘ghp∘)
(c) As p=p∘e−ρ∘ghp∘,
⇒Inpp∘=−ρ∘ghP∘
By question,
p=110p∘
⇒In⎛⎝110p∘p∘⎞⎠=−ρ∘gp∘h
⇒In110=−ρ∘gp∘hρ∘
h=−p∘ρ∘gIn110=−p∘p∘gIn(10)−1=p∘p∘gIn10
=p∘ρ∘g×2.303 [∵ In (x)=2.303log10(x)]
=1.013×1051.22×9.8×2.303=0.16×105m
=16×103m
(d) We know that p∝ρ (when T=constant i.e., isothermal pressure)
Temperature (T) remains constant only near the surface of the earth , not at greater heights.
Topper's Solved these Questions
- BOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF FLUIDSEXERCISE - Short Answer Type Questions5videos
MECHANICAL PROPERTIES OF FLUIDS
- BOOK - NCERT EXEMPLARCHAPTER - LAWS OF MOTIONEXERCISE - Long answer Type Questions9videos
LAWS OF MOTION
- BOOK - NCERT EXEMPLARCHAPTER - MECHANICAL PROPERTIES OF SOLIDSEXERCISE - LONG SHORT ANSWER TYPE QUESTION16videos
MECHANICAL PROPERTIES OF SOLIDS
Similar Questions
Knowledge Check
- Question 1 - Select One
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depth - Question 2 - Select One
It P is the pressure and ρ is the density of a gas, then P and ρ are realted as :
AP∝ρBP∝ρ2CP∝1/ρDP∝1/ρ2 - Question 3 - Select One
If P is the pressure of a gas and ρ is its density , then find the dimension of velocity in terms of P and ρ .
AP1/2ρ−1/2BP1/2ρ1/2CP−1/2ρ−1/2DP−1/2ρ−1/2
The density of air at one atmospheric pressure is 0.9kgm−3 What will be its density at 4 atmospheric pressure,when temperature remains constant?
View SolutionA small air bubble of radius r in water is at depth h below the water surface. If P0 is the atmospheric pressure, ρ is the density of water and T is the surface tension of water, what is the pressure inside the bubble?
View SolutionIf the atompspheric pressure is Pa then the jpressure P at depth a below the surface of a liquid of density ρ open to the atmosphere is
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
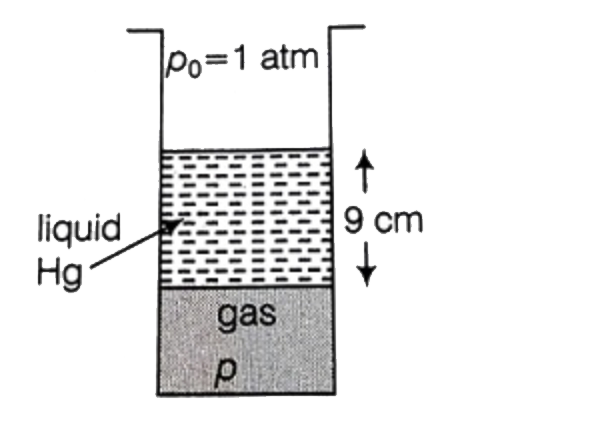 View Solution
View SolutionA beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
View Solution


