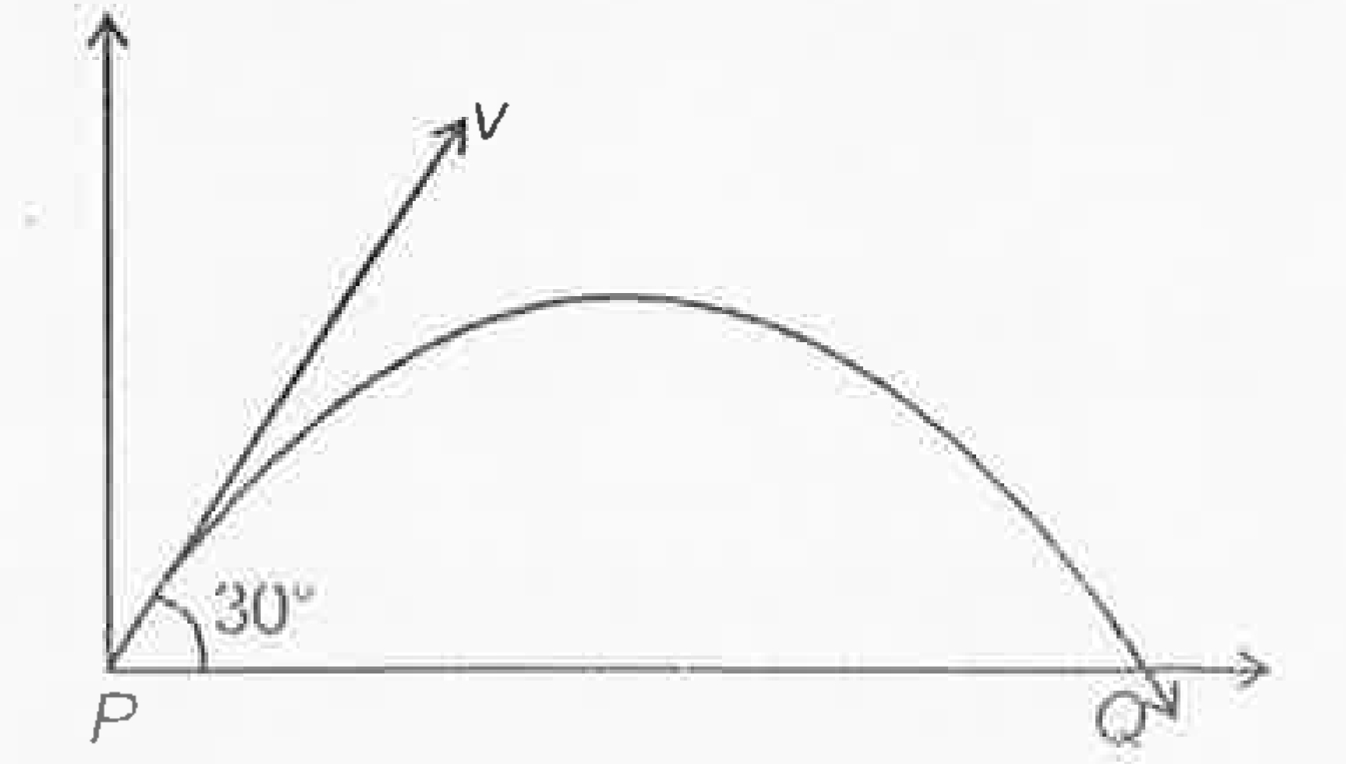
A projectile of mass m is fired with a velocity v from point P at an angle 45∘. Neglecting air resistance, the magnitude of the change in momentum leaving the point P and arriving at Q is

zero
mv√2
mv√2
2mv
The correct Answer is:C
Δp=pf−pi=m(vf−vi
=m[(v√2)ˆi−(v√2)ˆj)−(v√2)ˆi+(v√2)ˆj)]
−√2mvˆj
therfore|Δp|=√2mv
Topper's Solved these Questions
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Assertion and reason
21 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Match the coloumns
9 Videos
- CENTRE OF MASSBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASSEXERCISE - Check points
50 Videos
- CALORIMETRY AND HEAT TRANSFERBOOK - DC PANDEY ENGLISHCHAPTER - CALORIMETRY AND HEAT TRANSFEREXERCISE - Medical entrance s gallery
38 Videos
- CENTRE OF MASS, IMPULSE AND MOMENTUMBOOK - DC PANDEY ENGLISHCHAPTER - CENTRE OF MASS, IMPULSE AND MOMENTUMEXERCISE - Comprehension type questions
15 Videos
Similar Questions
A projectile of a mass m is fired with velocity v from point a as shown in figure neglecting air resistance, what is the change in momentum in x direction when leaving from P and arriving at Q?
View SolutionA particle of mass m is projected with a velocity v making an angle of 45∘ with the horizontal. The magnitude of the angular momentum of the projectile abut the point of projection when the particle is at its maximum height h is.
View SolutionA particle of mass m is projected with velocity v moving at an angle of 45∘ with horizontal. The magnitude of angular momentum of projectile about point of projection when particle is at maximum height, is
View SolutionA particle of mass m strikes elastically on a wall with velocity v, at an angle of 60∘ from the wall then magnitude of change in momentum of ball along the wall is
View SolutionA particle of mass m is projected with velocity making an angle of 45∘ with the horizontal When the particle lands on the level ground the magnitude of the change in its momentum will be .
View SolutionA particle of mass m is projected with a velocity v at an angle of θ with horizontal. The angular momentum of the particle at the highest point of its trajectory is equal to :
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View SolutionA particle of mass m is projected from the ground with an initial speed u at an angle α. Find the magnitude of its angular momentum at the highest point of its trajector about the point of projection.
View SolutionA particle of mass m carrying charge q is projected with velocity v from point P toward aninfinite line of charge from a distance a . Its speed reduces to zero momentarily at point Q, which is at a distance a/2 from the line of charge. If another particle with mass m and charge −q is projected with the same velosity v from P toward the line of charge, what will be its speed at Q ?
View SolutionA particle is projected at time t=0 from a point P wilth a speed v0 at an angle of 45∘ to the horizontal. Find the magnitude and the direction of the angular momentum of the particle about the point P at time t=v0g
View Solution
