A ray of light is falling on a glass sphere of μ=√3 such that the incident ray and the emergent ray, when produced, intersect at a point on the surface of the sphere. Find the value of angle of incidence.
The correct Answer is:60∘
To solve the problem, we need to find the angle of incidence (I) for a ray of light falling on a glass sphere with a refractive index (μ) of √3. The condition given is that the incident ray and the emergent ray intersect at a point on the surface of the sphere when produced.
1. Understanding the Geometry:
- Let point A be the point where the ray of light strikes the surface of the sphere.
- OA is the normal to the surface at point A.
- The angle of incidence (I) is the angle between the incident ray and the normal OA.
2. Refraction at Point A:
- When the ray enters the sphere, it refracts at point A, making an angle of refraction (R) with the normal.
- According to Snell's Law:
μ1sinI=μ2sinR
where μ1=1 (for air) and μ2=√3 (for glass). Thus:
sinI=√3sinR
3. Emergence at Point B:
- The ray travels through the sphere and emerges at point B, making an angle of emergence (E) with the normal at point B.
- Given that the angle of incidence equals the angle of emergence (I = E), we can denote the angle of emergence as E.
4. Isosceles Triangle OAB:
- In triangle OAB, OA = OB (radii of the sphere).
- Therefore, angles OAB and OBA are equal. Let both be R.
- The angle AOB can be expressed as:
Angle AOB=180∘−2R
5. Angles at Point D:
- When the incident ray and emergent ray are produced, they intersect at point D on the sphere.
- By vertically opposite angles, we have:
Angle DAO=IandAngle DBA=E=I
- Therefore, angle ADB can be expressed as:
Angle ADB=I−R
6. Cyclic Quadrilateral ADBE:
- The angles in cyclic quadrilateral ADBE satisfy:
Angle ADB+Angle AEB=180∘
- We can express angle AEB as:
Angle AEB=12Angle AOB=12(180∘−2R)=90∘−R
- Thus:
(I−R)+(90∘−R)=180∘
- Simplifying gives:
I−2R+90∘=180∘⟹I−2R=90∘⟹I=90∘+2R
7. Substituting into Snell's Law:
- From Snell's Law, we have:
sinI=√3sinR
- Substituting I=90∘+2R into the sine function gives:
sin(90∘+2R)=cos(2R)
- Thus:
cos(2R)=√3sinR
8. Using Trigonometric Identities:
- We can express sinR in terms of cosR:
sinR=√1−cos2R
- Substitute this into the equation to solve for R.
9. Finding the Angle of Incidence:
- After solving the equations, we find:
I=60∘
Final Answer:
The angle of incidence I is 60∘.
- Let point A be the point where the ray of light strikes the surface of the sphere.
- OA is the normal to the surface at point A.
- The angle of incidence (I) is the angle between the incident ray and the normal OA.
- When the ray enters the sphere, it refracts at point A, making an angle of refraction (R) with the normal.
- According to Snell's Law:
μ1sinI=μ2sinR
where μ1=1 (for air) and μ2=√3 (for glass). Thus:
sinI=√3sinR
- The ray travels through the sphere and emerges at point B, making an angle of emergence (E) with the normal at point B.
- Given that the angle of incidence equals the angle of emergence (I = E), we can denote the angle of emergence as E.
- In triangle OAB, OA = OB (radii of the sphere).
- Therefore, angles OAB and OBA are equal. Let both be R.
- The angle AOB can be expressed as:
Angle AOB=180∘−2R
- When the incident ray and emergent ray are produced, they intersect at point D on the sphere.
- By vertically opposite angles, we have:
Angle DAO=IandAngle DBA=E=I
- Therefore, angle ADB can be expressed as:
Angle ADB=I−R
- The angles in cyclic quadrilateral ADBE satisfy:
Angle ADB+Angle AEB=180∘
- We can express angle AEB as:
Angle AEB=12Angle AOB=12(180∘−2R)=90∘−R
- Thus:
(I−R)+(90∘−R)=180∘
- Simplifying gives:
I−2R+90∘=180∘⟹I−2R=90∘⟹I=90∘+2R
- From Snell's Law, we have:
sinI=√3sinR
- Substituting I=90∘+2R into the sine function gives:
sin(90∘+2R)=cos(2R)
- Thus:
cos(2R)=√3sinR
- We can express sinR in terms of cosR:
sinR=√1−cos2R
- Substitute this into the equation to solve for R.
- After solving the equations, we find:
I=60∘
The angle of incidence I is 60∘.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Single Correct221videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Assertion-Reasoninig2videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.721videos
GEOMETRICAL OPTICS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.315videos
ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYS
- BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit27videos
HEATING EFFECT OF CURRENT
Similar Questions
A ray of light is incident on a glass sphere of refractive index 3/2. What should be the angle of incidence so that the ray which enters the sphere does not come out of the sphere ?
View SolutionThe angle between the incident ray and emergent ray, when the ray is passing through an equilateral prism is called angle of _____.
View Solution
Knowledge Check
- Question 1 - Select One
Light is incident on a glass surface at polarising angle of 57.5∘ Then the angle between the incident ray and the refracted ray is
A57.5∘B115∘C205∘D145∘ - Question 2 - Select One
A ray of light is incident at small angle I on the surface of prism of small angle A and emerges normally from the oppsite surface. If the refractive index of the material of the prism is mu, the angle of incidence is nearly equal to
AAμBA2μCμADμA2
A horizontal ray of light is incident on a solid glass sphere of radius R and refractive index μ . What is net deviation of the beam when it emerged from the other side of the sphere?
View SolutionA ray of light is incident at small angle I on the surface of prism of small angle A and emerges normally from the oppsite surface. If the refractive index of the material of the prism is mu, the angle of incidence is nearly equal to
View SolutionA ray of light is incident at small angle I on the surface of prism of small angle A and emerges normally from the oppsite surface. If the refractive index of the material of the prism is mu, the angle of incidence is nearly equal to
View SolutionA ray of light is incident on the surface of a transparent sphere of R.I.μ=√72. After refraction is is internally reflected and refracted out of the sphere as shown in the figure. Find the angle of incidence 'I' so that the total deviation produced is minimum. 'C' is the centre of the sphere.
 .View Solution
.View SolutionA ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is
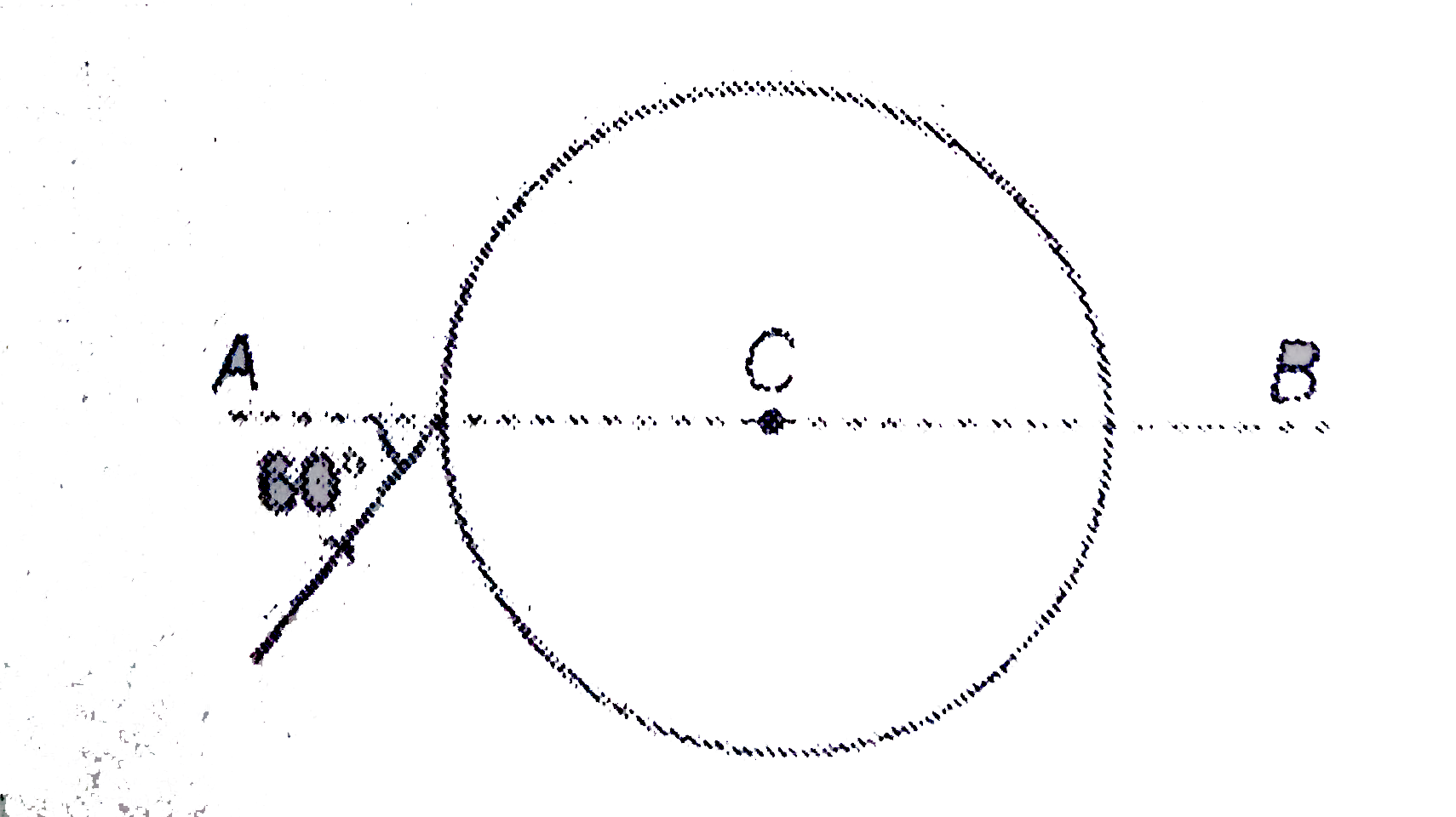 View Solution
View SolutionA ray of light falls on a transparent sphere with centre at C as shown in figure. The ray emerges from the sphere parallel to line AB. The refractive index of the sphere is
 View Solution
View Solution
