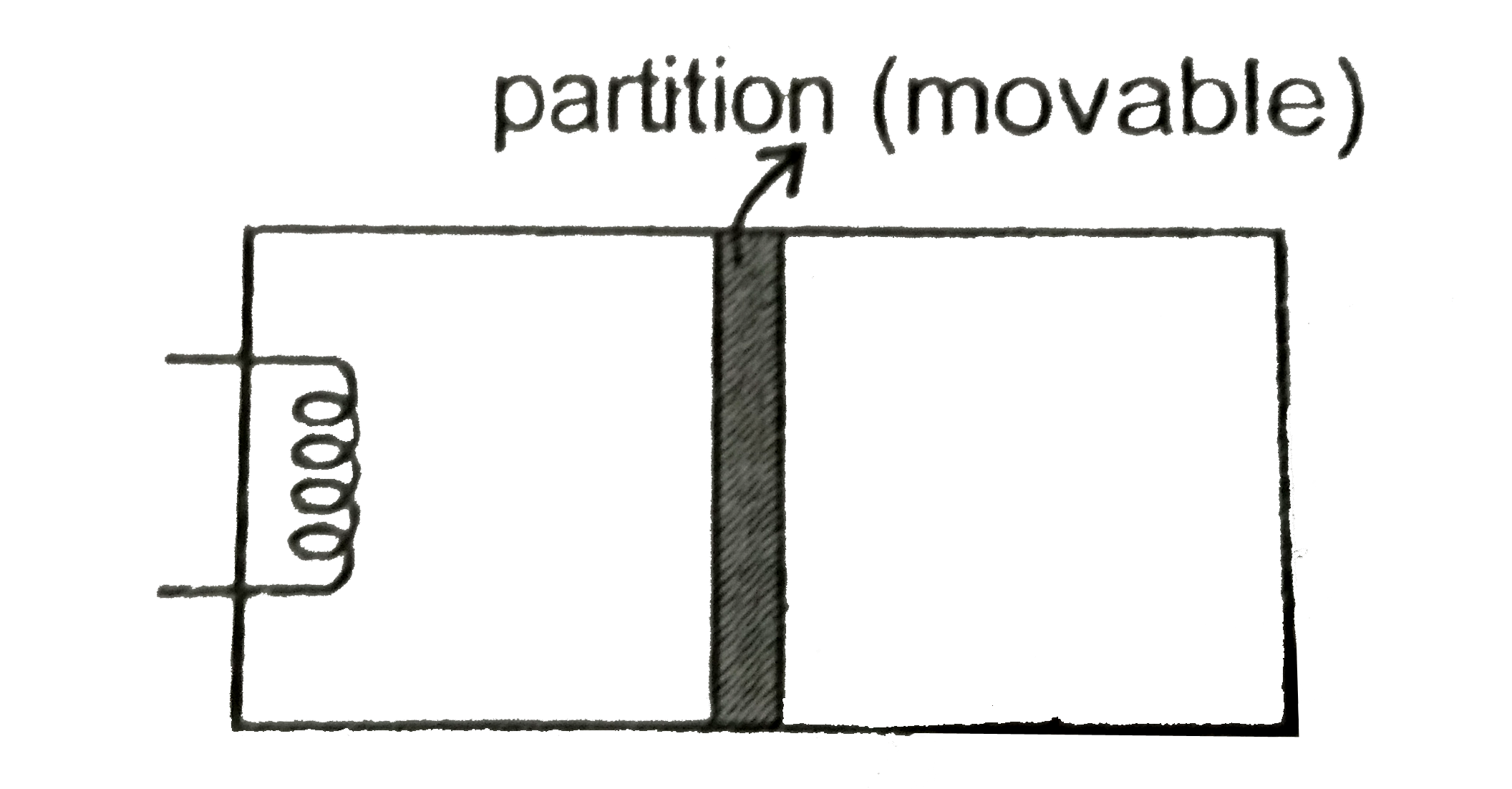
A rectangualr box (shown in figure) has a movable and smooth portition which can slide along the length of the box. Both chambers contains 1 mole of monoatomic gas ( $γ = \frac{5}{3}$ ) at a pressure walls of box and partition are thermally insulated. Due to heating, gas in left chamber expands until pressure in both chambers become $32 P_{0}$ determine
(a) The final temperature of gas in each chamber
(b) The work done by the gas in the right chamber.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B

For the left chamber
$\frac{P_{0} V_{0}}{T_{0}} = \frac{P_{0} \times 243}{32 \times T_{1}} \times V_{1}$
$\Rightarrow T_{1} = \frac{234}{32} \times \frac{V_{1} T_{0}}{V_{0}} \dots . . (i)$
For the right chamber for adiabatic compression
We get, $P_{0} V_{0}^{γ} = P_{0} \times \frac{243}{32} \times V_{2}^{γ}$
$\Rightarrow \frac{V_{2}}{V_{0}} = {(\frac{32}{243})}^{3 / 5} \Rightarrow V_{2} = \frac{8}{27} V_{0}$
But $V_{1} + V_{2} = 2 V_{0}$
$∴ V_{1} = 2 V_{0} - V_{2} = 2 V_{0} - \frac{8}{27} V_{0} = \frac{46}{27} V_{0} ... . (i i)$
From (i) and (ii) $T_{1} = \frac{243}{32} \times \frac{46 \times V_{0}}{V_{0} \times 27} \times T_{0}$
or, $T_{1} = \frac{207}{16} T_{0} = 12.9 T_{0} (\approx .) < b r > T o f \in d t h e t e m p e r a t u r e \in t h e sec o n d c h a m b e r (), w e < b r > a p p l y < b r >$ ((T_1)/(T_2))^(gamma)=((P_2)/(P_1))^(1-gamma) $< b r >$ rArr((T_0)/(T_2))^(5//3)=((243P_0)/(32P_0))^(1-5//3) rArr T_2=2.25 T_0 $< b r > W or k d o \neq \in r i g h t c h a m b e r (a d i a b a t i c p r o c e s s) < b r >$ W=1/(1-gamma)(P_2V_2-P_0V_0) $< b r >$ =-3/2[243/32P_0xx8/27V_0-P_0V_0] $< b r >$ =-3/2(9/4-1)P_0V_0=-15/8xxRT_0=-15.8T_0

|

Updated on:21/07/2023

Class 11 PHYSICS THERMODYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One or More
A closed box contains an ideal gas if temperature of gas increased which
Amean free path remain same
Bmean free path decreases
Crelaxation time decreases
Drelaxation time remain same
Question 2 - Select One
The pressure of the gas in a cylindrical chamber is $p_{0}$ . The vertical force exerted by the gas on its hemispherical end is

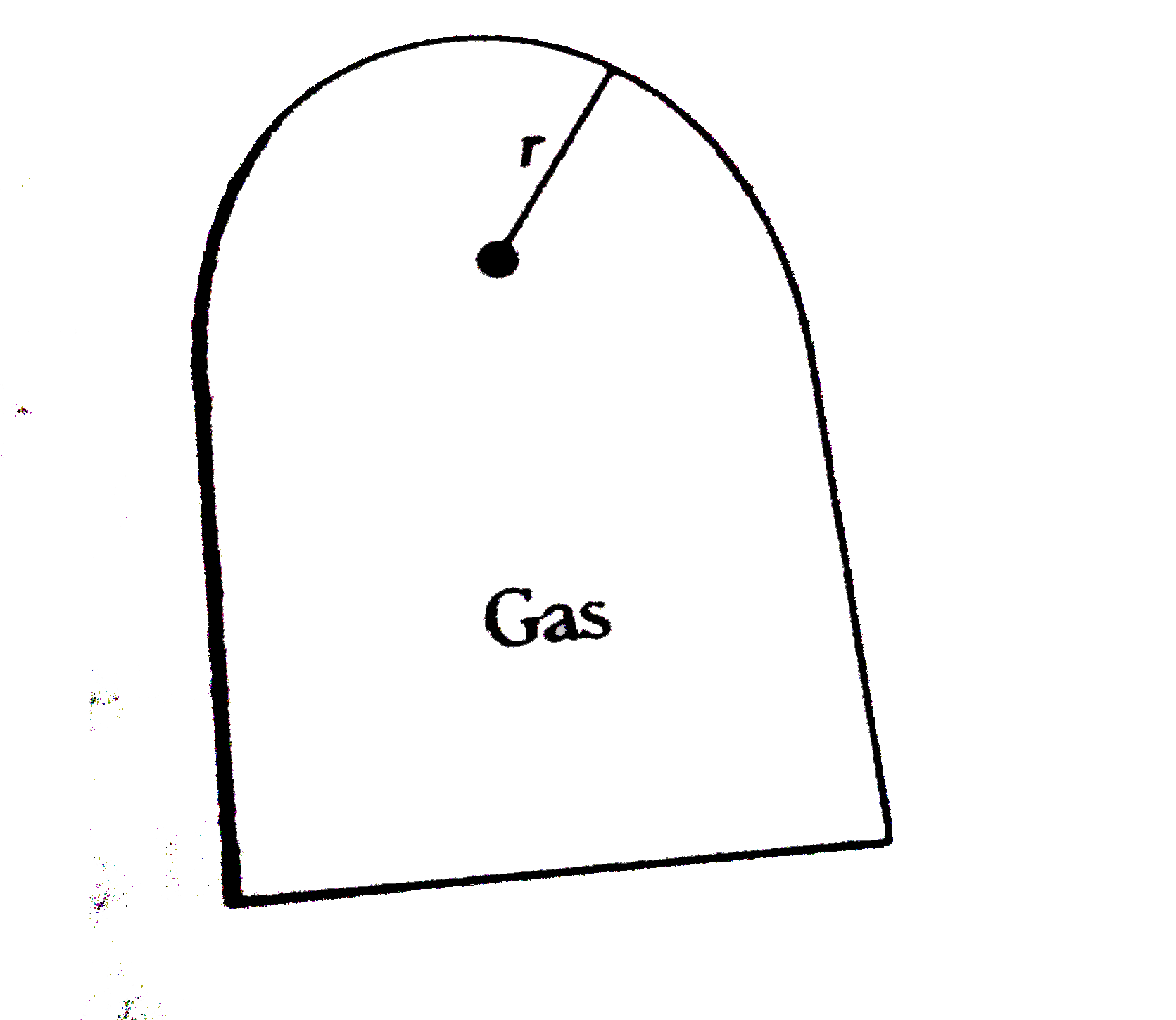A $p_{0} r^{2}$
B $4 p_{0} π r^{2}$
C $2 p_{0} π r^{2}$
D $p_{0} π r^{2}$
Question 3 - Select One
One box containing $1$ mole of $H e$ is $7 / 3 T_{0}$ and other box containing $1$ mole of a polyatomic gas $(γ = 1.33)$ at $T_{0}$ are placed together to attain thermal equilibrium. The final temperature $T_{f}$ . Then :
A $T_{f} = \frac{9}{13} T_{0}$
B $T_{f} = \frac{13}{9} T_{0}$
C $T_{f} = \frac{T_{0}}{2}$
D $T_{f} = \frac{3}{2} T_{0}$

SUNIL BATRA (41 YEARS IITJEE PHYSICS)-HEAT AND THERMODYNAMICS-JEE Main And Advanced

Home
Ask Doubt
Profile