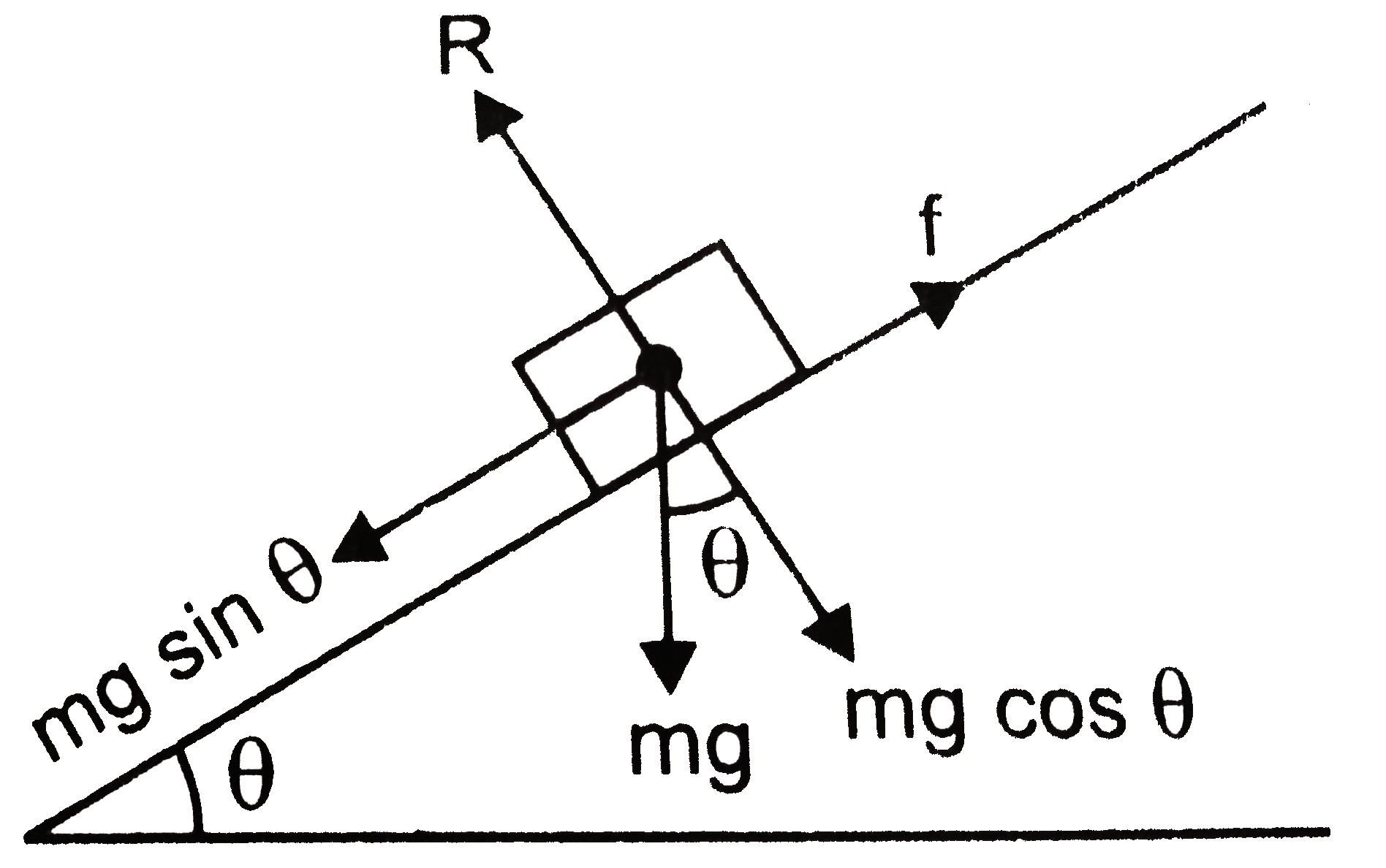
A rectangular box lies on a rough inclined surface . The coefficient of friction between the surface and the box is μ. Let the mass of the box be m .
(a) At what angle of inclination θ of the plane to the horizontal will the box just start to slide down the plane ?
(b) What is the force acting on the box down the plane , if the angle of inclination of the plane is increased to α>θ ?
(c) What is the force needed to be applied upwards along the plane to make the box either remain stationary or just move up with uniform speed ?
(d) What is the force needed to be applied upwards along the plane to make the box move up the plane with acceleration a ?
(a) As the box tends to slide down force of friction will act up the plane
As is clear from box will just start slidding down , when
mgsinθ=f=μR=μmgcosθ
or tanθ=μorθ=tan−1(μ)
(b) When angle of inclination is increased to α>θ then net force acting on the box down the plane is
F1=mgsinα−f=mgsinα+f=mg(sinα+μcosα)
(In this case friction would act down the plane ) (d) If the box is to be moved with an upward acceleration a then upward force needed
F3=mg(sinα+μcosα)+ma
 .
.
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Curiosity Question
1 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Short Answer Questions
26 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Very short answer questions
73 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 1 NCERT Comprehension
4 Videos
- MATHEMATICAL TOOLSBOOK - PRADEEPCHAPTER - MATHEMATICAL TOOLSEXERCISE - Fill in the blanks
5 Videos
Similar Questions
A rectangular box lies on a rough inclined surface. The coefficient of friction between the surface and the box is (μ) Let the mass of the box be m.
At what angle of inclination θ of the plane to the horizontal will the box just start to slide down the plane ?
View SolutionA rectangular box lies on a rough inclined surface . The coefficient of friction between the surface and the box is μ. Let the mass of the box be m .
(a) At what angle of inclination θ of the plane to the horizontal will the box just start to slide down the plane ?
(b) What is the force acting on the box down the plane , if the angle of inclination of the plane is increased to α>θ ?
(c) What is the force needed to be applied upwards along the plane to make the box either remain stationary or just move up with uniform speed ?
(d) What is the force needed to be applied upwards along the plane to make the box move up the plane with acceleration a ?
View Solution
Knowledge Check
A block of mass m rests on a rough inclined plane. The coefficient of friction between the surface and the block is µ. At what angle of inclination θ of the plane to the horizontal will the block just start to slide down the plane?
Aθ=tan−1μBθ=cos−1μCθ=sin−1μDθ=sec−1μA rectangular box lies on a rough inclined surface The co-efficient of friction beteen the surface and the box is μ. Le the mass of the box ne m What is the force acting on the box down the plane if the angle of inclination of the plane is increased to α>θ ? .
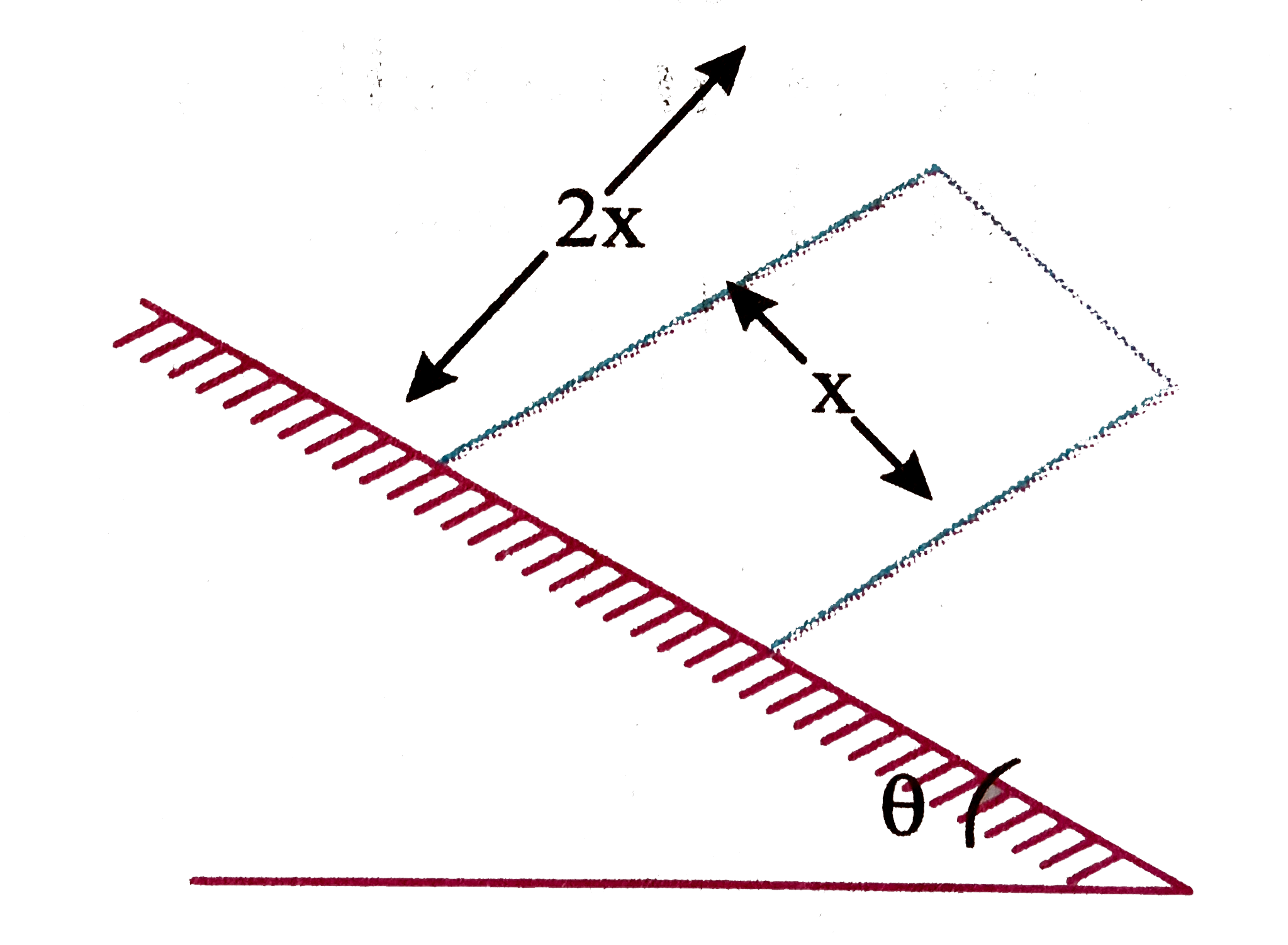
Amg(Sinα+μCosα)BmgCosθCmg(Sinα−μCosα)DmgSinθ+fA uniform box is kept on a rough inclined plane. It begins to topple when θ is equal to:
 A30∘B60∘Ctan−1(12)D45∘
A30∘B60∘Ctan−1(12)D45∘
A rectangular box lies on a rough inclined surface The co-efficient of friction beteen the surface and the box is μ. Le the mass of the box ne m What is the force acting on the box down the plane if the angle of inclination of the plane is increased to α>θ ? .
View SolutionA body is sliding down a rough inclined plane The coefficient of friction between the body and the plane is 0.5.The ratio of the net force required for the body to slide down and the normal reaction on the body is 1:2 Then the angle of the inclined plane is
View SolutionA body is sliding down a rough inclined plane The coefficient of friction between the body and the plane is 0.5.The ratio of the net force required for the body to slide down and the normal reaction on the body is 1:2 Then the angle of the inclined plane is
View SolutionThe angle which the rough inclined plane makes with the horizontal when the body placed on it just starts sliding down is called
View SolutionA body weighing 20 kg just slides down a rough inclined plane that rises 5 in 12. The coefficient of friction is
View Solution
