A rectangulat block of refractive index is placed on a printed page lying on a horizontal surface as shown in Fig. , Find the minimum value of so that the letter L on the page is not visible from any of the vertical sides.
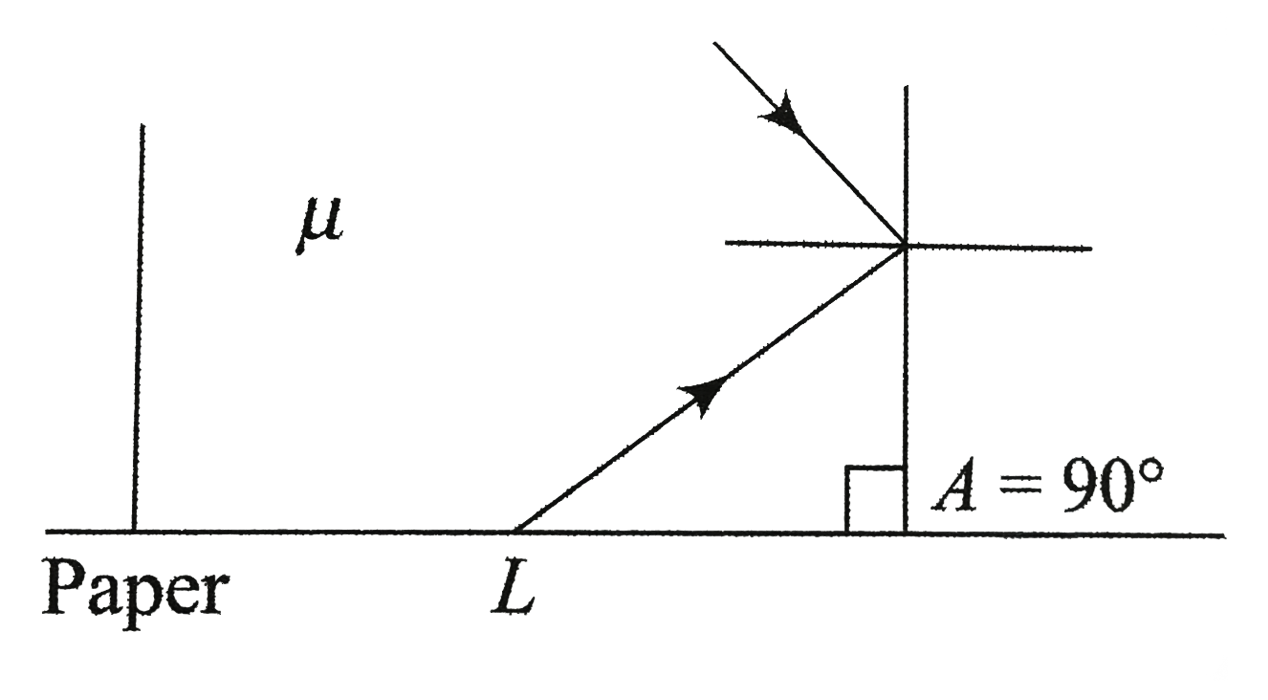
The correct Answer is:A, D
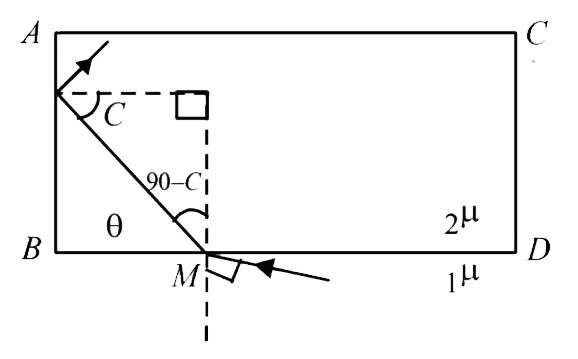
For a grazing incident ray at BD for which the angle of refraction is maximum. For this C is least. Let C is greater than the critical angle.
Applying Snell's law at M
....(i)
Also ....(ii)
When C is the critical angle.
From (i) and (ii), (1)/(cosC)=(1)/(sinC)impliesC=45degree:'_1^2mu=(1)/(sin45degree)=sqrt2=1.41
Topper's Solved these Questions
Similar Questions
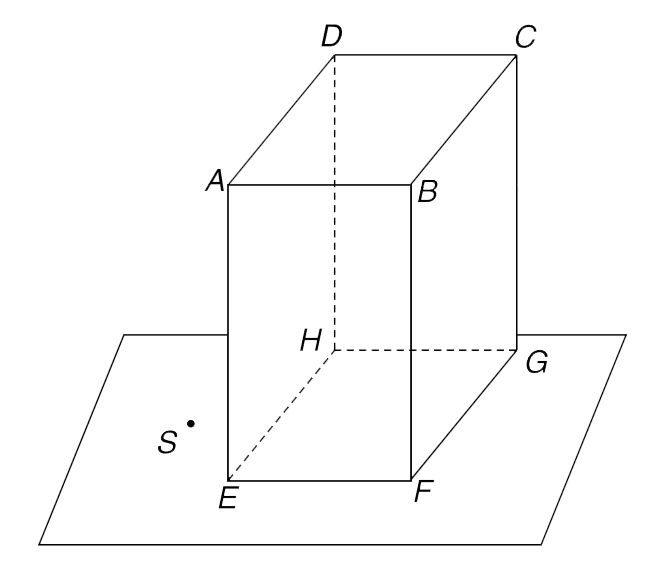
A large rectangular glass block of refractive index μ is lying on a horizontal surface as shown in Figure. Find the minimum value of μ so that the spot S on the surface cannot be seen through top plane ‘ABCD’ of the block.
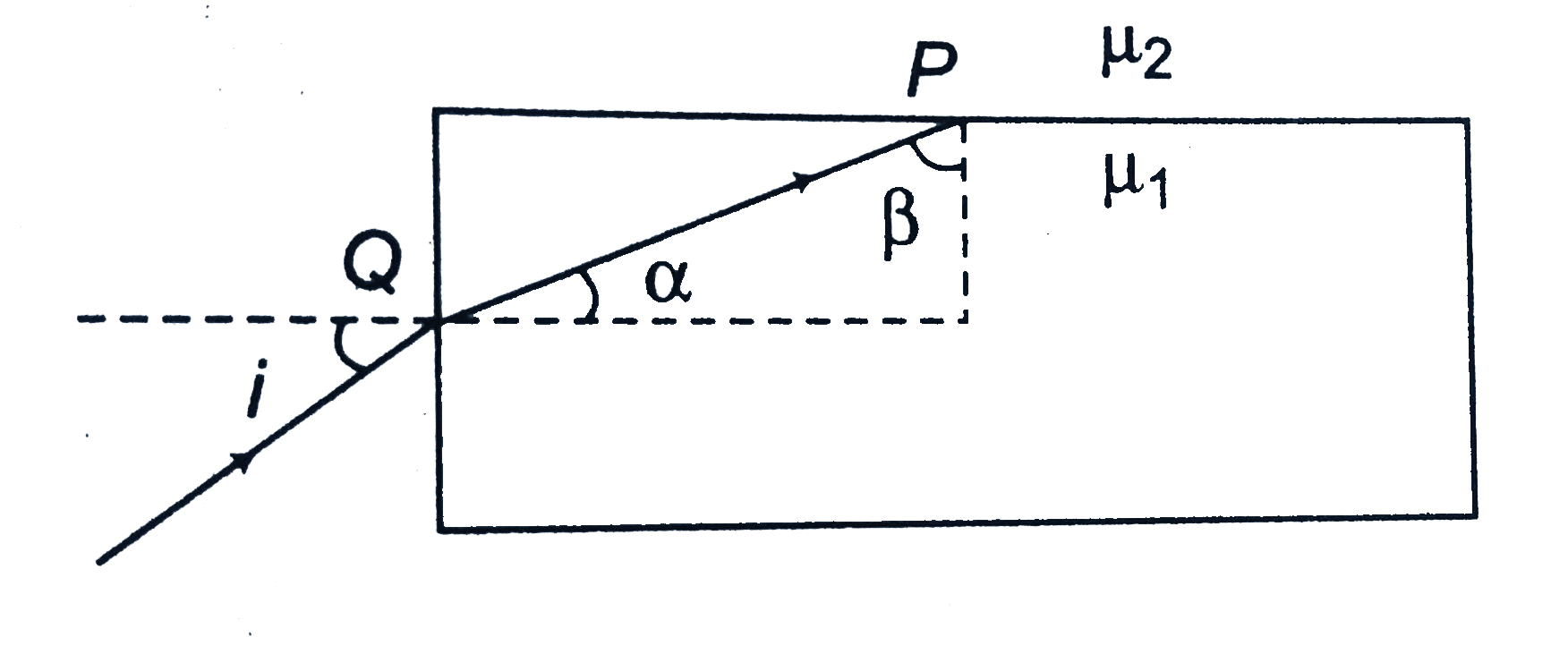
View SolutionIn the figure shown, μ1>μ2. Find minimum value of i so that TIR never takes place at P.
View Solution
Knowledge Check
A cubical transparent slab is used as a paper weight. What should be the minimum refractive index of the material of the slab so that letters below it are not visible from any of its vertical faces.
A√2B√3C√5D√5/√3Word ‘Newton’s printed on a paper and is placed on a horizontal surface below a cubical glass. The minimum value of refractive index of a cubical glass for which letters are not visible from any vertical faces, of the glass, is (Critical angle =45°)
A√3B0.5C1D√2In Fig. 7.150 , coefficient of friction between m2 and the horizontal surface is μ , minimum value of m so that the system has zero acceleration , is :
 Aμ(m1+m2)Bμ(m1+m2)Cm2−m1μDm1μ−m2
Aμ(m1+m2)Bμ(m1+m2)Cm2−m1μDm1μ−m2
A block is rotating in contact with vertical wall (rotor) as shown. Find the minimum value of ω so that the block does not slide down.

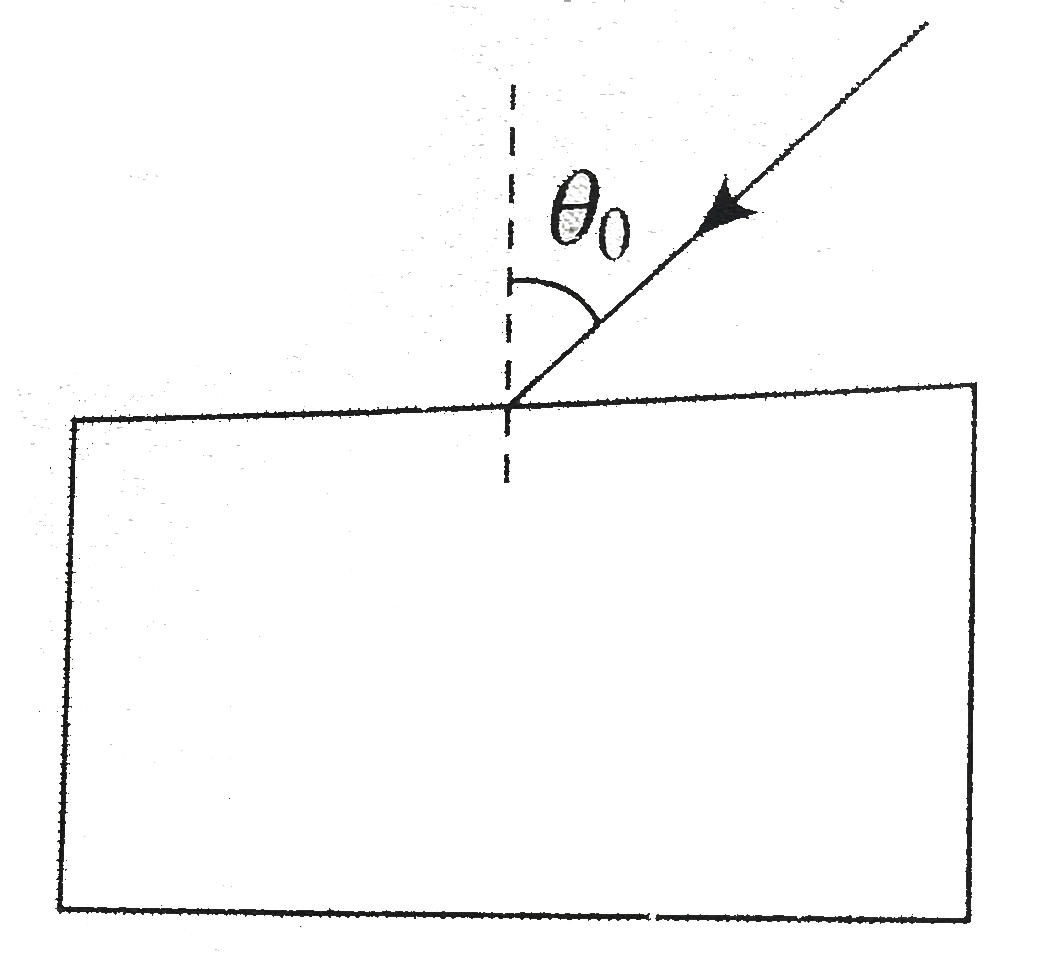
View SolutionA slab of refractive index μ is placed in air and light is incident at maximum angle θ0 from vertical. Find minimum value of μ for which total internal reflection takes place at the vertical surface.
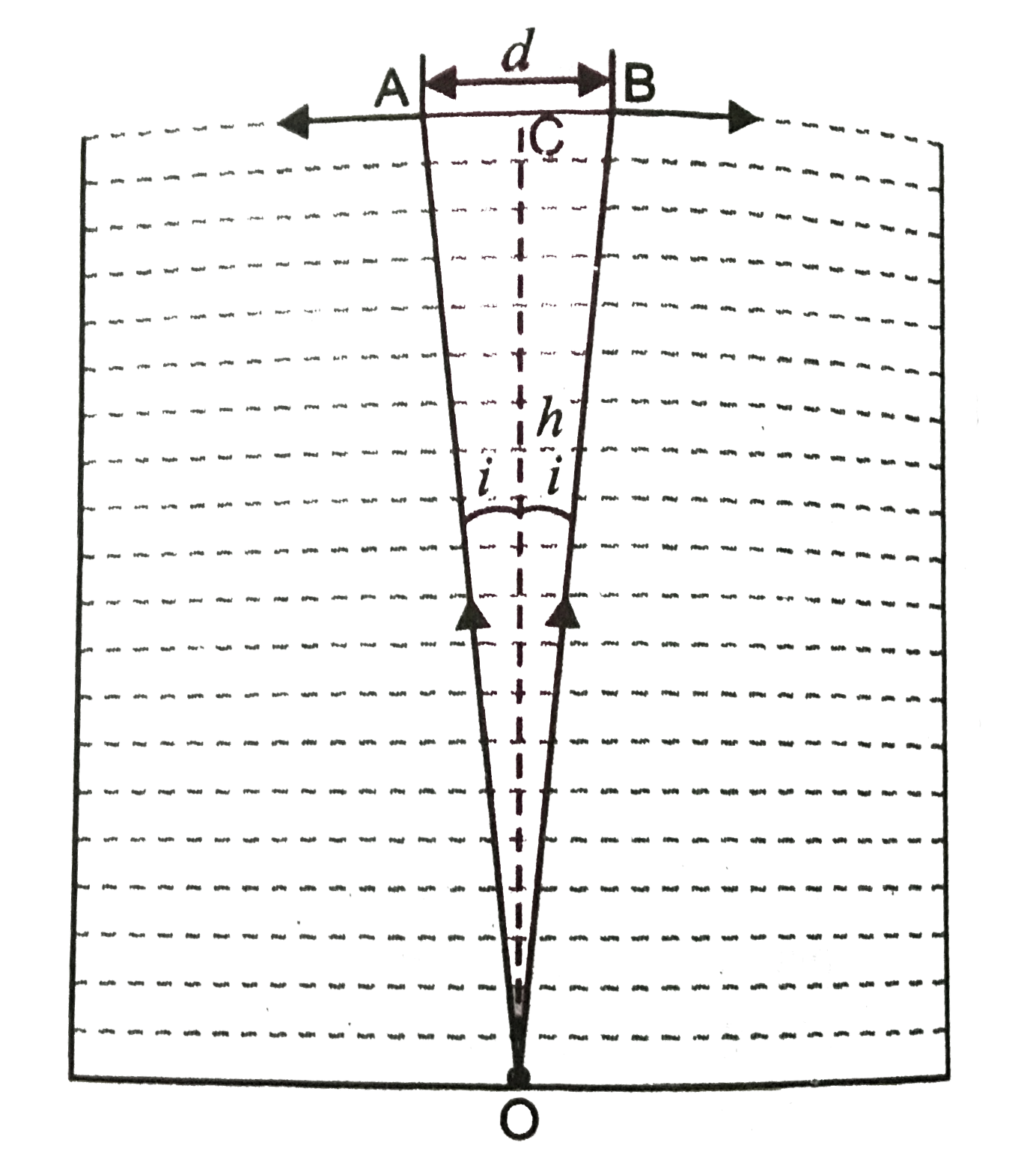
View SolutionA jar of height h is filled wih a transparent liquid of refractive index μ, Fig. At the centre of the jar on the botom surface is a dot. Find the minimum diameter of a disc, such that when placed on the top surface symmetrically about the centre, the dot is invisible.
 .
.
View SolutionA block of mass m is placed on another block of mass M lying on a smooth horizontal surface as shown in the figure. The co-efficient of friction between the blocks is μ. Find the maximum horizontal force F (in Newton) that can be applied to the block M so that the blocks together with same acceleration? (M=3kg,m=2kg,μ=0.1,g=10m/s2)

View SolutionTwo blocks of masses 1 kg and 2 kg are placed in contact on a smooth horizontal surface as shown in fig. A horizontal force of 6N is applied on a 1-kg block. Find the force of interaction of the blocks.

View Solution
