A resistance of 25Ω is connected to a 12V battery. Calculate the heat energy in joules generated per minute.
The correct Answer is:345.6 J
To solve the problem of calculating the heat energy generated per minute by a resistance of 25 ohms connected to a 12V battery, we can follow these steps:
1. Identify the given values:
- Resistance (R) = 25 Ω
- Voltage (V) = 12 V
2. Use Ohm's Law to find the current (I):
- Ohm’s Law states that I=VR.
- Substitute the values:
I=12 V25 Ω=0.48 A
3. Calculate the heat energy (H) generated using the formula:
- The formula for heat energy generated due to resistance is given by Joule's law:
H=I2R
- Substitute the current value into the formula:
H=(0.48 A)2×25 Ω
- Calculate I2:
(0.48)2=0.2304
- Now calculate H:
H=0.2304×25=5.76 J/s
- This value (5.76 J/s) represents the heat energy generated per second.
4. Convert the heat energy to per minute:
- Since we need the heat energy generated per minute, multiply the value by 60 (the number of seconds in a minute):
Hper minute=5.76 J/s×60 s=345.6 J
5. Final Result:
- The heat energy generated per minute is 345.6 joules.
- Resistance (R) = 25 Ω
- Voltage (V) = 12 V
- Ohm’s Law states that I=VR.
- Substitute the values:
I=12 V25 Ω=0.48 A
- The formula for heat energy generated due to resistance is given by Joule's law:
H=I2R
- Substitute the current value into the formula:
H=(0.48 A)2×25 Ω
- Calculate I2:
(0.48)2=0.2304
- Now calculate H:
H=0.2304×25=5.76 J/s
- This value (5.76 J/s) represents the heat energy generated per second.
- Since we need the heat energy generated per minute, multiply the value by 60 (the number of seconds in a minute):
Hper minute=5.76 J/s×60 s=345.6 J
- The heat energy generated per minute is 345.6 joules.
Topper's Solved these Questions
- ELECTRICITY BOOK - LAKHMIR SINGH & MANJIT KAURCHAPTER - ELECTRICITY EXERCISE - Very Short Answer Type Questions
1 Videos
- ELECTRICITY BOOK - LAKHMIR SINGH & MANJIT KAURCHAPTER - ELECTRICITY EXERCISE - Short Answer Type Questions
2 Videos
- ELECTRICITY BOOK - LAKHMIR SINGH & MANJIT KAURCHAPTER - ELECTRICITY EXERCISE - Sample Problem
1 Videos
- MAGNETIC EFFECT OF ELECTRIC CURRENT BOOK - LAKHMIR SINGH & MANJIT KAURCHAPTER - MAGNETIC EFFECT OF ELECTRIC CURRENT EXERCISE - NCERT BOOK
2 Videos
Similar Questions
Knowledge Check
Three equal resistances, each of RΩ are connected as shown in figure. A battery of emf 2 V and internal resistance 0.1Ω is connected across the circuit. Calculate the value of R for which the heat generated in the circuit is maximum?
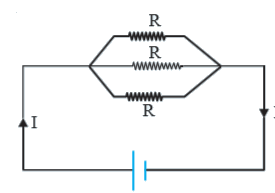 A0.2ΩB0.3ΩC0.4ΩDnone of these
A0.2ΩB0.3ΩC0.4ΩDnone of theseA coil o finductanc e8.4mH and resistance 6Ω is connected to a 12V battery. The current in the coil is 1.0A in the time (approx .)
A500secB20secC35msecD1msecA coil of inductance 8.4mH and resistance 6 Ω is connected to a 12V battery. The current in the coil is 1.0A at approximately the time.
A500secB20 secC35 millisecD1 millisec
A conductor of resistance 25Ω of diameter 0.2 mm is connected to a battery of 200 V . Calculate the current density for the conductor.
View SolutionThree equal resistace, each of R ohm, are connectedd as shown in the figure. A battery of 2V and of internal resistance 0.1 ohm is connected across the circuit. The value of R for which the heat generated in the circuit maximum will be
View SolutionA coll of resistance 10 Ω and inductance 5 H is connected at a 100 V battery. Then the energy stored in the coil is
View SolutionA coil of inductance 8.4 mH and resistance 6(Ω) is connected to a 12 V battery. The current in the coil is 1.0 A at approximately the time
View SolutionA resistive coil of self-inductance 2 H and resistance 5Ω is connected across a battery of 50 V. How much energy will be stored in the inductor?
View Solution
