A rigid ball traveling in a straight line thex−aξs hits a soled wall and suddenly rebounds during a brief instant . The vx−t grap in . shows this ball s velocty as a function of time. During the first 20s of its motion, find (a) its displacement (b) the total destance the ball moves, and (c ) skerch a graph of ax−t for this ballsmotion.(d)Isthegraphshownreallyvertcalat5 s?
Explain.
 .
.
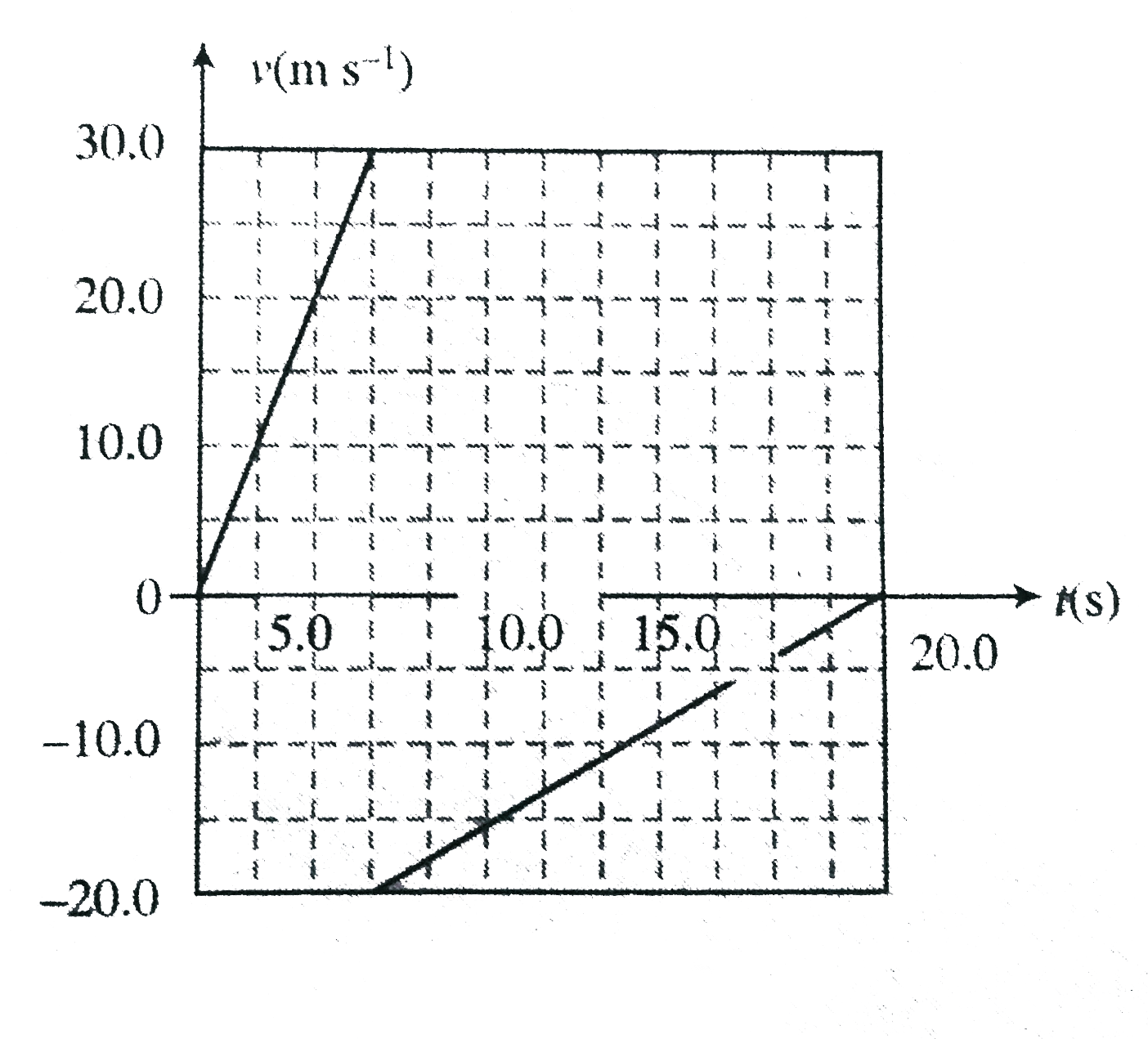
For t=0 to 5s,vx, positive and the ball moves in the-x direction. The acceleration ax is negative ball moves in the-x direction. The acceleration ax is the slope of the vx versus t graph.
a. Far t=0 to 5s Displacement=Change in position
=Area under vx−t graph
x5−x0=(v0_(x)+vx2)t=((0+3)2)(5)=75m
The ball travels a distance of 75m.
For t=5s to 20s displacement=Changein position
x20−x5=(−20+02)(15)=−150m
The total displacement is x20−x0=55+(−150m)=−75m.
The ball ends up 75m in the negative x direction from where it graph.
b. The total distance traveled is 75m+15m=225m,
c. Instantanceons acceleration is the slope of velocity-time graph.
For t=0 to 5s,
ax=30−05=6ms−2
For
 .
.
t=5s to 20s, ax=0−(−20)15=43ms−2
The ball of ax versis t is given in .
d. The ball is in contact with the floor for a small but non-zero period of time and the direction of the velocity does change instantaneously. So, no the actul graph of vx t is not really verticalcal at 5s.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Subjective
29 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Single Correct
52 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.3
15 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
- KINEMATICS-2BOOK - CENGAGE PHYSICS ENGLISHCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
Knowledge Check
A ball is travelling with uniform translatory motion . This means that
Ait is at restBhe path can be a straight line or circular and the ball travels with uniform speed.Call parts of the ball have the same velocity (magnitude and direction) and the velocity is constantDthe centre of the ball moves with constant velocity and the ball spins about its centre uniformly.A ball is travelling with uniform translatory motion . This means that
Ait is at rest.Bthe path can be a straight line or circular and the ball travels with uniform speed.Call parts of the ball have the same velocity (magnitude and direction) and the velocity is constant.Dthe centre of the ball moves with constant velocity and the ball spins about its centre uniformly.
A ball is thrown vertically upward with a speed of 20 m/s. Draw a graph showing the velocity of the ball as a function of time as it goes up and then comes back.
View SolutionA particle moving in a straight line has its velocit varying with time according to relation v=t2−6t+8(m/s) where t is in seconds. The CORRECT statement(s) about motion of this particle is/are:-
View SolutionA particle is moving in a striaght line and at a given time, its displacement is S=t3−6t2+9t, where t is in seconds and S is in meter . The total distance travelled by the particle during the first five seconds is
View SolutionA ball of mass 0.2 kg travelling in a straight line with a speed of m/s along negative x- axis id deflected by a bat 15m/s along negative x - axis is deflected by a bat at an angle of 30∘ If the speed of the ball after deflection is 10m/s, find the impulse on the ball.
View SolutionA ball is projected from ground with speed u, at an angle θ above horizontal. Let v be its speed at any moment t and s be the total distance covered by it till this moment, the correct graph (s) is/are.
View SolutionA ball moves on a smooth floor in a straight line with a uniform velocity 10 m s−1 for 6 s. At t = 6 s, the ball hits a wall and comes back along the same line to the starting point with same speed. Draw the velocity-time graph and use it to find the total distance travelled by the ball and its displacement.
View Solution
