A ring is rolling, without slipping on a horizontal surface with constant velocity. Speed of point A (at the top) is vA. After an interval T, the speed of point A again becomes vA. During what fraction of the interval T speed of point A was greater than √32vA
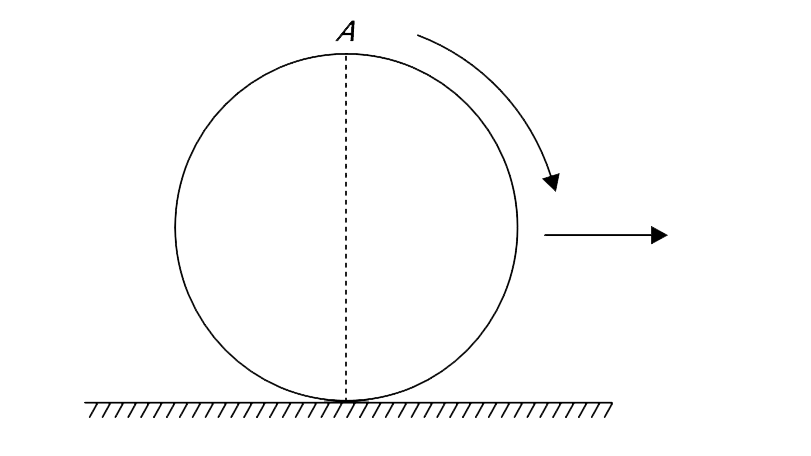
The correct Answer is:13
Step by step video, text & image solution for A ring is rolling, without slipping on a horizontal surface with constant velocity. Speed of point A (at the top) is v_(A). After an interval T, the speed of point A again becomes v_(A). During what fraction of the interval T speed of point A was greater than (sqrt3)/(2) v_(A) by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0 . We consider a point A on the surface of the disc. Then, the acceleration of point A is
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View Solution
Knowledge Check
A ring performs pure rolling on a horizontal fixed surface with its centre moving with speed v.
AMaximum possible speed that any particle of ring may have is v/√2BMinimum possible speed that any particle of ring may have is v/√2Cparticles on the ring may have speed anywhere between 0 and 2u.DNone of theseA wheel rolls without slipping on a horizontal surface such that its velocity of center of mass is v. The velocity of a particle at the highest point of the rim is
AzeroBvC2vD√2vA symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
Av/RBContinuously increasingCDependent on mass MDIndependent of radius (R)
A circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View SolutionA disc rolls without slipping on a horizontal surface such that its velocity of center of the mass is v. Find the velocity of points A,B,C and D.
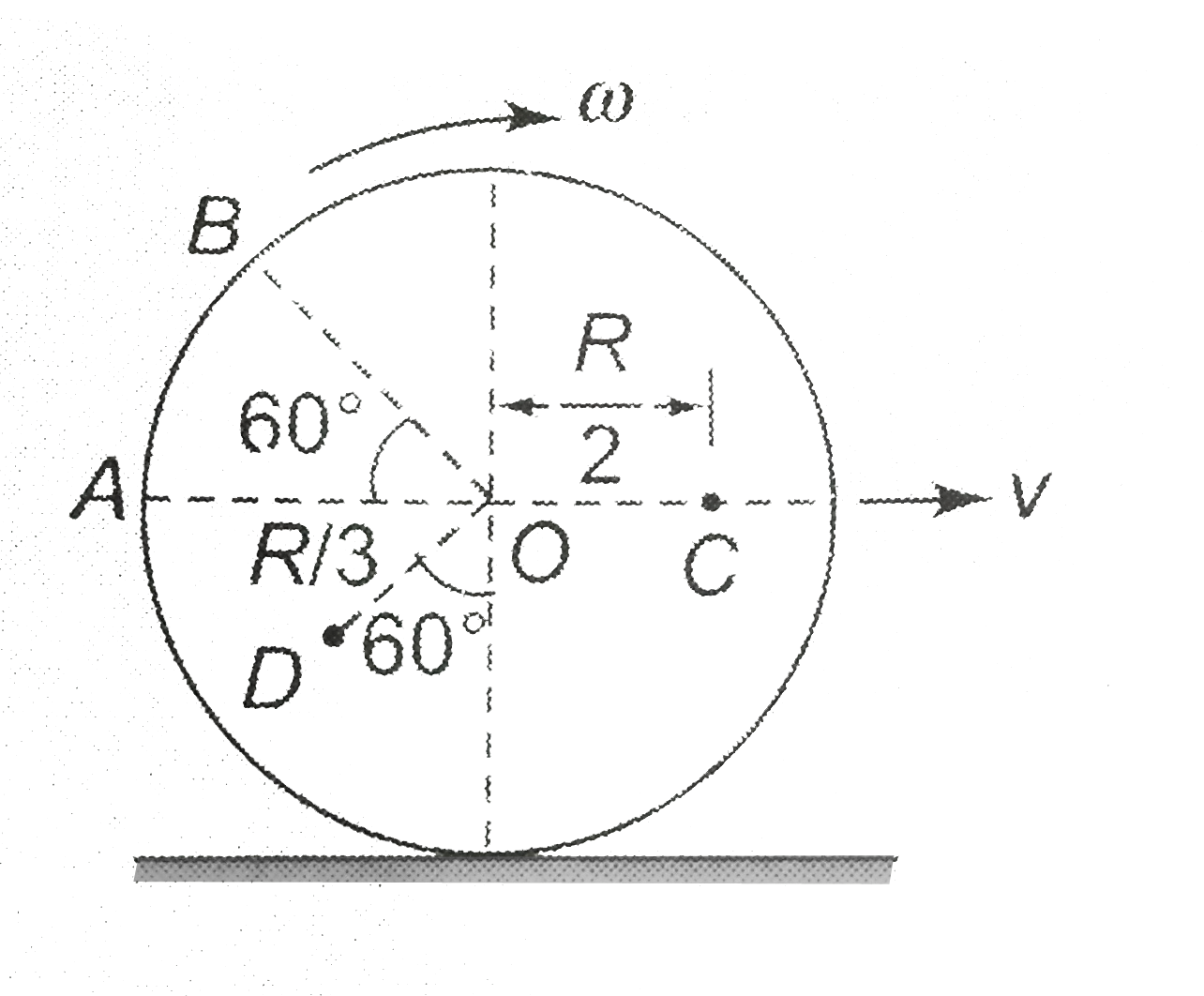
View SolutionDuring rolling without slipping, what is the velocity of the point in contact with the surface.
View SolutionA disc of radius R is rolling without sliding on a horizontal surface at a constant speed of v
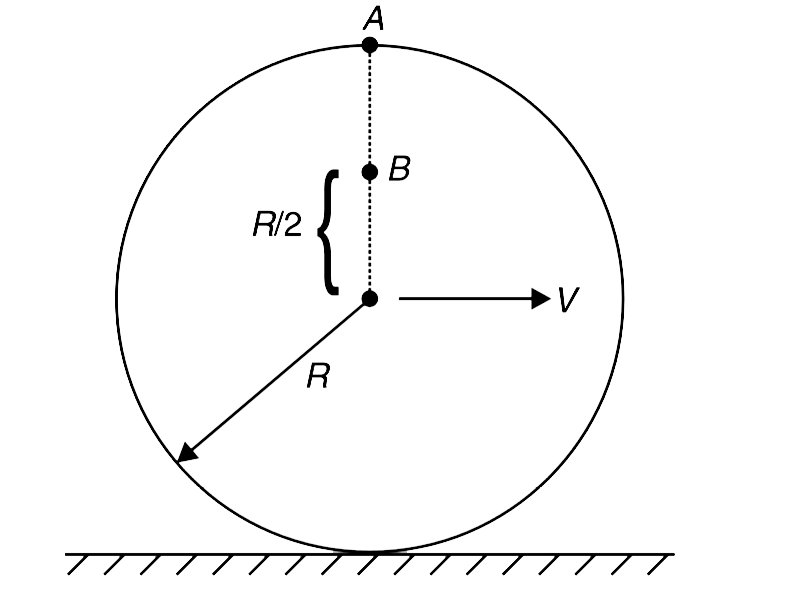
(a) What is speed of points A and B on the vertical diameter of the disc ? Given AB=R2
(b) After what time, for the first time, speed of point A becomes equal to present speed (i.e., the speed at the instant shown in the figure) of point B?
View SolutionA disc of radius R rolls without slipping at speed v along positive x-axis. Velocity of point P at the instant shown in Fig. is
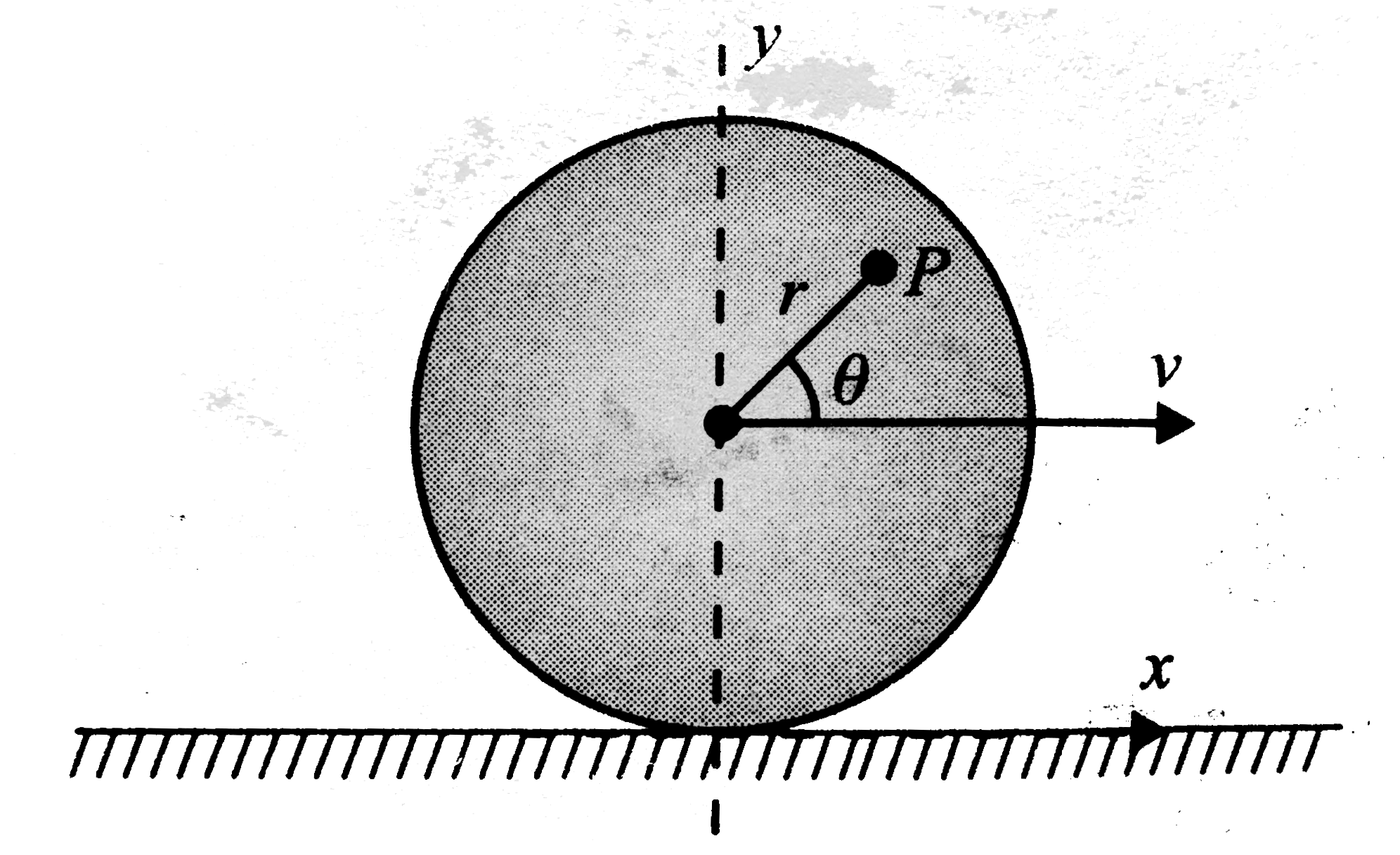
View Solution
