A disc of radius R is rolling without sliding on a horizontal surface at a constant speed of v
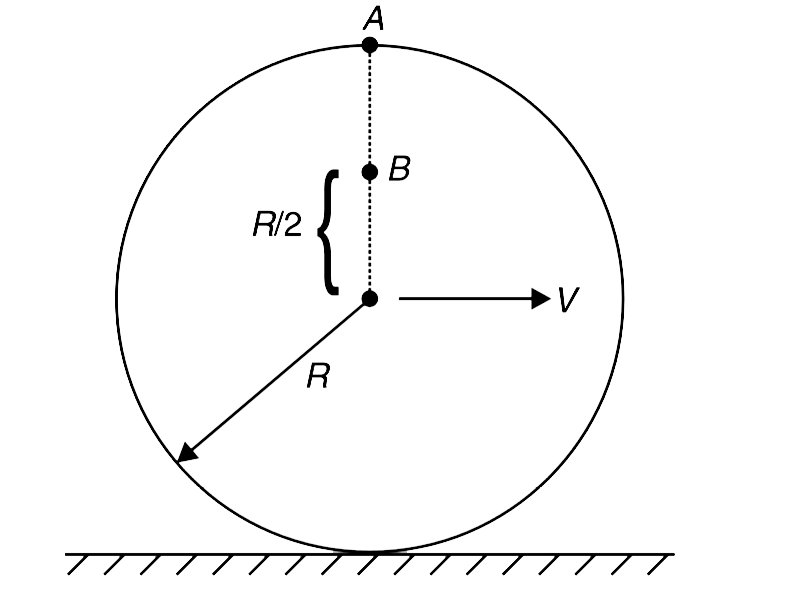
(a) What is speed of points A and B on the vertical diameter of the disc ? Given AB=R2
(b) After what time, for the first time, speed of point A becomes equal to present speed (i.e., the speed at the instant shown in the figure) of point B?
The correct Answer is:(a) VA=2V,VB=3V2
(b) t=2RVcos−1(34)
Step by step video, text & image solution for A disc of radius R is rolling without sliding on a horizontal surface at a constant speed of v (a) What is speed of points A and B on the vertical diameter of the disc ? Given AB = (R)/(2) (b) After what time, for the first time, speed of point A becomes equal to present speed (i.e., the speed at the instant shown in the figure) of point B? by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
A circular disc of radius r is rolling without slipping on a horizontal surface. What is the ratio of the translational KE and rotational KE of disc ?
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0 . We consider a point A on the surface of the disc. Then, the acceleration of point A is
View Solution
Knowledge Check
A symmetrical body of mass M and radius R is rolling without slipping on a horizontal surface with linear speed v. Then its angular speed is
Av/RBContinuously increasingCDependent on mass MDIndependent of radius (R)A disc is rolling without sliding on a horizontal surface. Velocity of the centre of the disc is V. Then the maximum relative sped of any point on the circumference of the disc with respect to point P is.
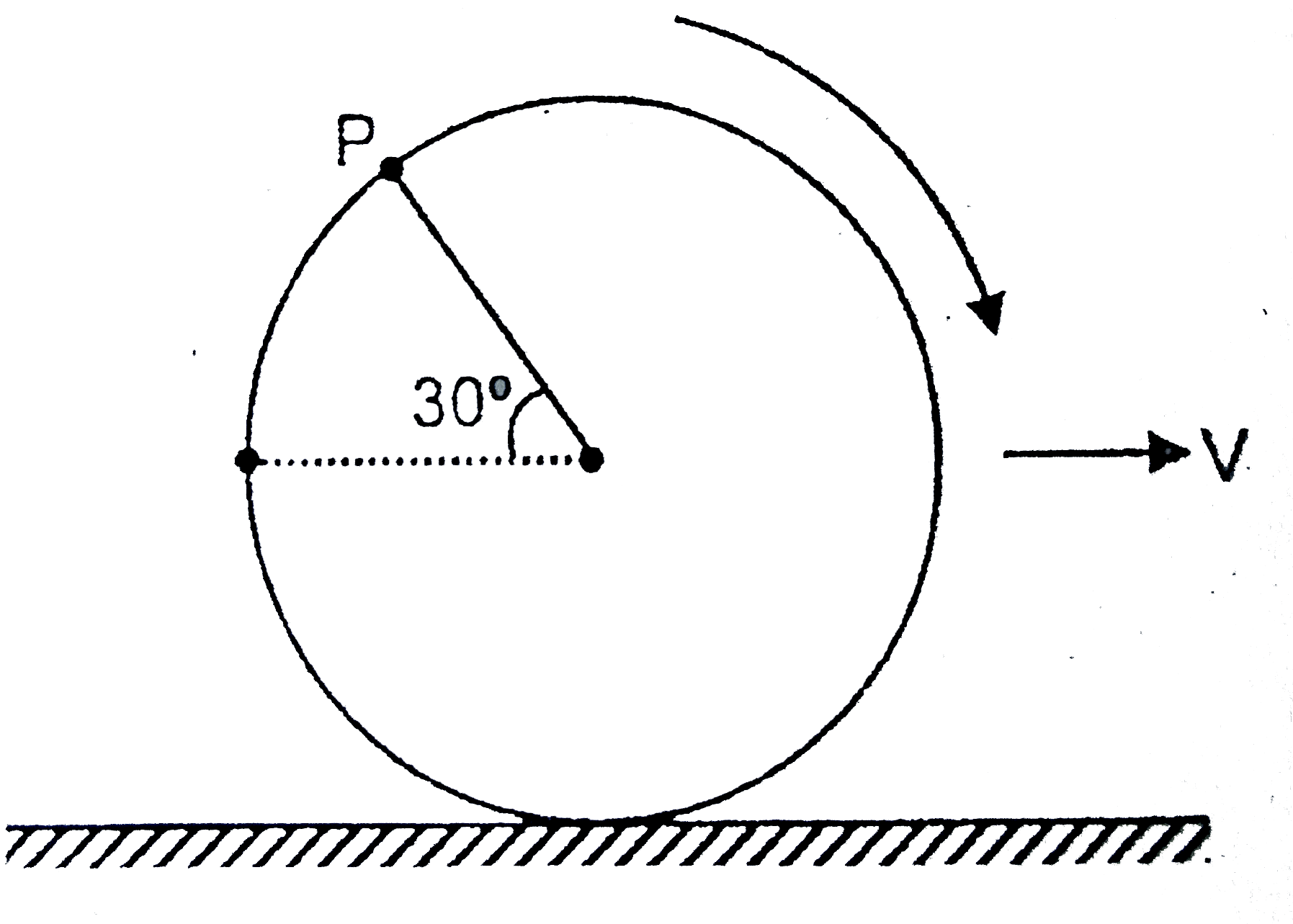 AVB2VC3VD2V3
AVB2VC3VD2V3A disc having radius R is rolling without slipping on a horizontal (x−z) plane. Centre of the disc has a velocity v and acceleration a as shown.

Speed of point P having coordinates (x,y) isAv√x2+y2RBv√x2+(y+R)2RCv√x2−(y−R)2RDnone
A circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
View SolutionA circular disc of radius R rolls without slipping along the horizontal surface with constant velocity v0. We consider a point A on the surface of the disc. Then the acceleration of the point A is:
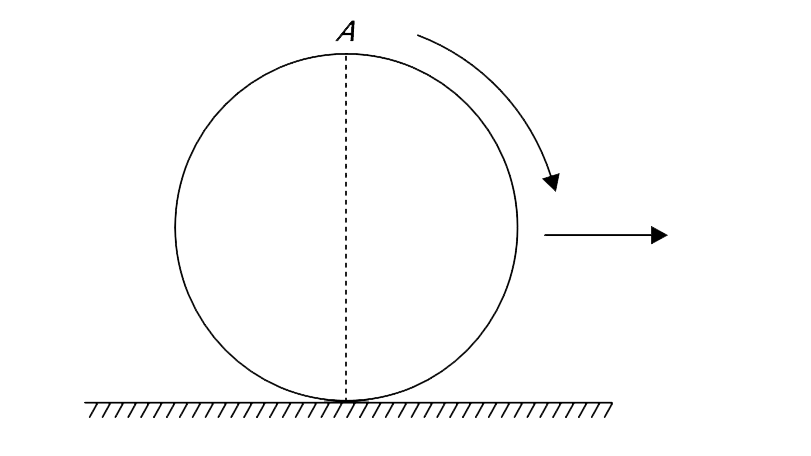
View SolutionA ring is rolling, without slipping on a horizontal surface with constant velocity. Speed of point A (at the top) is vA. After an interval T, the speed of point A again becomes vA. During what fraction of the interval T speed of point A was greater than √32vA
View SolutionA disc of radius R is moving on a rough horizontal surface with velocity v and angular speed ω at an instant as shown in figure. Choose the correct statement.

View SolutionIf a disc of mass 400 gm is rolling on a horizontal surface with uniform speed of 2 m/s, then its rolling kinetic energy will be
View Solution
