A ring of thin wire with active resistance R and inductance L rotates with constant angular velocity ω in the external uniform magneitce field perpendicular to the rotation axis. In the process , the flux of magnetic induction of external field across the ring varies with time as Φ=Φ0cosωt. Demonstrate that
(a) the inductive current in the ring varies with time as I=Imsin(ωt−φ), where Im=.mΦ/√R2+ω2L2 with tanφ=ωL/R,
(b) the mean mechanical is defined by the formula P=1/2ω2Φ20R/(R2+ω2L2).
(a) We have
ε=−dΦdt=ωΦ0sinωt=L.I+RI
Put I=Imsin(ωt−φ). Then
ωΦ0sinωt=ωΦ0{sin(ωt−φ)cosφ+cos(oω−φ)sinφ}
=LImωcos(ωt−φ)+RImsin(ωt−φ)
so RIM=ωΦ0cosφ and LIm=Φ0sinφ
or Im=ωΦ0√R2+ω2L2 and tanφ=ωLR
(b) Mean mechanical power required to maintain rotation = energy loss per unit time
=1T∫T0RI2dt=12RI2m=12ω2Φ20RR2+ω2L2
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Elastic Waves
39 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OSCILLATIONS AND WAVESBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OSCILLATIONS AND WAVESEXERCISE - Electromagnetic Waves, Radiation
36 Videos
- OPTICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - OPTICSEXERCISE - Exercise
2 Videos
- PHYSICAL FUNDAMENTALS OF MECHANICSBOOK - IE IRODOV, LA SENA & SS KROTOVCHAPTER - PHYSICAL FUNDAMENTALS OF MECHANICSEXERCISE - Relativistic Mechanics
49 Videos
Similar Questions
A ring of mass m and radius R is being rotated about its axis with constant angular velocity ω in the gravity free space. Find tension in the ring.
View SolutionA ring rotates with angular velocity ω about an axis perpendicular to the plane of the ring passing through the center of the ring.A constant magnetic field B exists parallel to the axis.Find the emf induced in the ring.
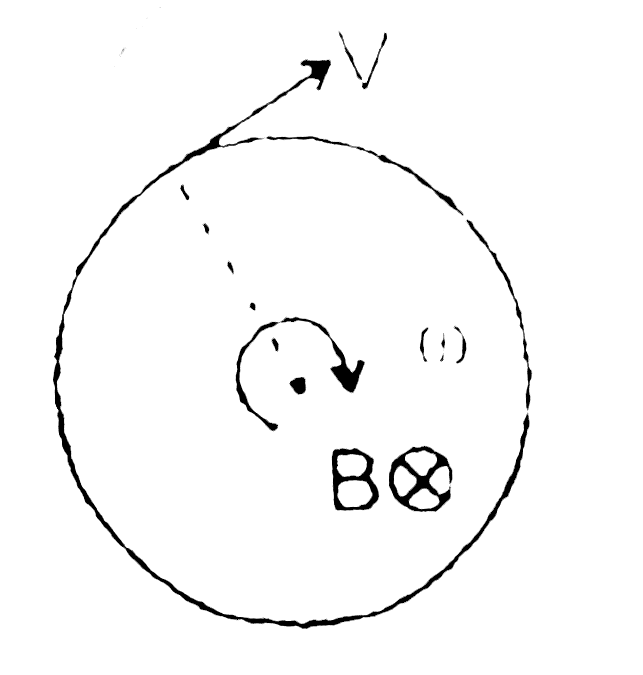
View Solution
Knowledge Check
A horizontal ring of radius r spins about its axis with an angular velocity ω in a uniform vertical magnetic field of magnitude B. The emf induced in the ring is
AzeroBπr2ωBC12Br2ωDBr2ωA thin circular ring of mass M and radius R is rotating about its axis with constant angular velocity ω. The objects each of mass m are attached gently to the ring. The wheel now rotates with an angular velocity.
AωM(m+M)Bω(M−2M)(M+2m)CωM(M+2m)Dω(M+2m)MA conducting ring of radius R has charge Q distributed unevenly over it. If it rotates with an angular velocity ω, the equivalent current will be
AzeroBQωCQω2πDQω2πR
A conducting ring of radius r having charge q is rotating with angular velocity ω about its axes. Find the magnetic field at the centre of the ring.

View SolutionA ring rotates with angular velocity ω about an axis perpendicula to the plane of the ring passing through the center of the ring (Fig. 3.77). A constant magnetic field B exists parallel to the axis. Find the emf induced in the ring.
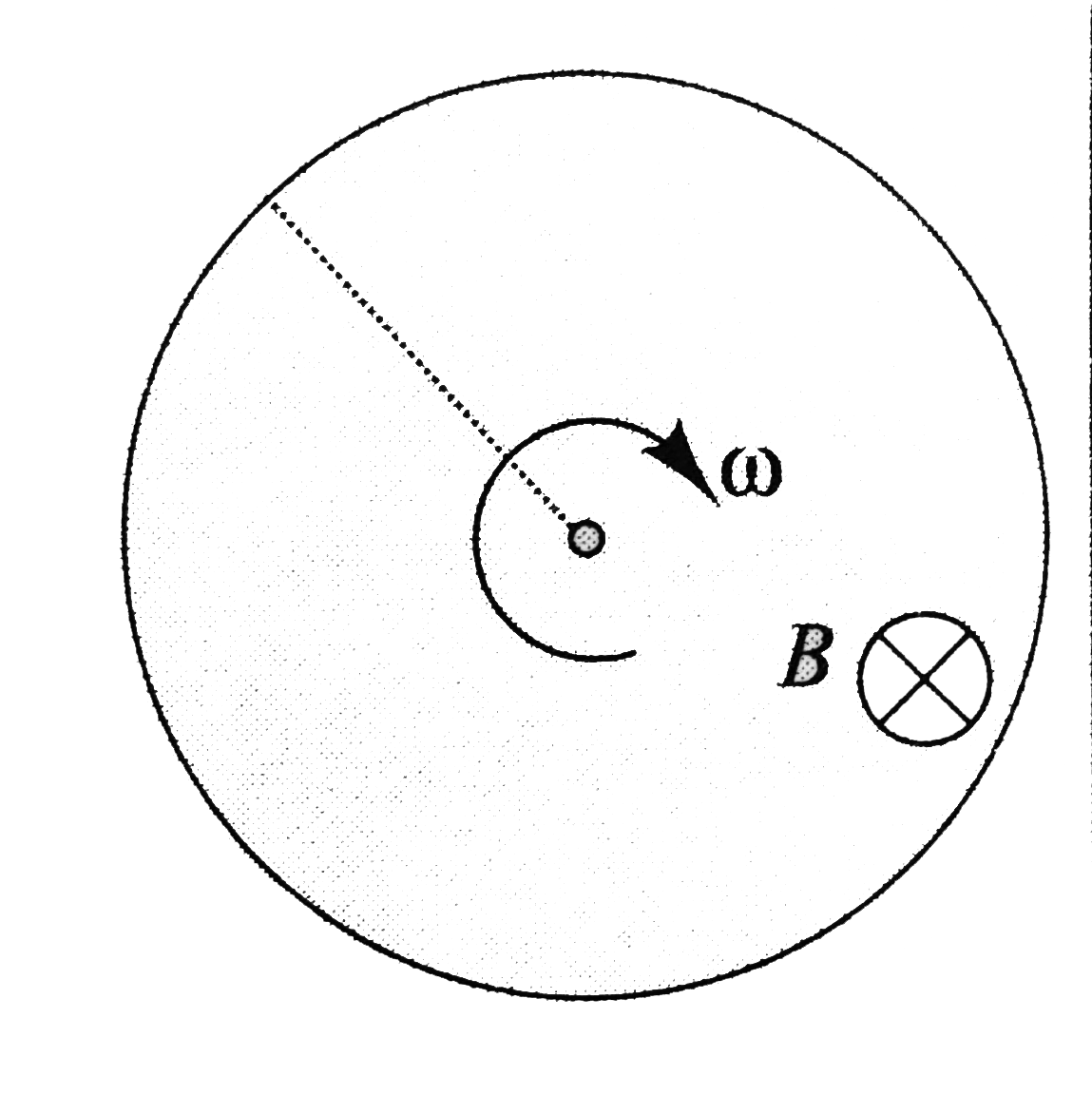
View SolutionA ring of mass m and radius R is being rotated about its axis with angular velocity ω. If a increases then tension in ring
View SolutionA wire is bent to form a semicircle of the radius a. The wire rotates about its one end with angular velocity ω . Axis of rotation is perpendicular to the plane of the semicircle . In the space , a uniform magnetic field of induction B exists along the aixs of rotation as shown in the figure . Then -
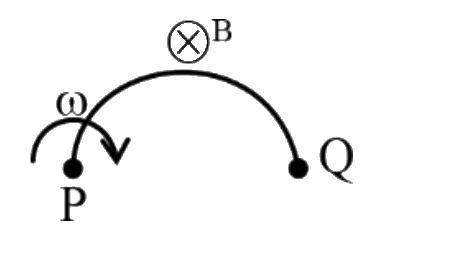
View SolutionA ring of mass m and radius R is given a charge q. It is then rotated about its axis with angular velocity ω.Find
(ii) Magnetic field produced at the centre of ring.
View Solution
