A rod of weight W is kept horizontally on two knife edges with a distance d between them. Centre of gravity of the rod is at a distance x from A. Find the normal reactions at points A and B Fig.

Let the centre of gravity of the rod be C. Normal reactions at A and B are R1 and R2. Weight W through C acting downwards and R1 and R2 are the three coplanar forces that keep the rod in equilibrium.
Hence, W - R1−R2=0 or,W=R1+R2
Taking the moment of the forces about C,
−R1.x+R2(d−x)=0or,R1x=R2(d−x)
or, x(R1+R2)=R2d or,Wx=R2d
∴ R2=xdW
and R1=W−R2=W−Wxd=W(1−xd).
Topper's Solved these Questions
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
18 Videos
- STATICSBOOK - CHHAYA PUBLICATIONCHAPTER - STATICSEXERCISE - EXERCISE (Multiple Choice Questions )
14 Videos
- SIMPLE HARMONIC MOTIONBOOK - CHHAYA PUBLICATIONCHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - CBSE SCANNER
16 Videos
- SUPERPOSITION OF WAVESBOOK - CHHAYA PUBLICATIONCHAPTER - SUPERPOSITION OF WAVESEXERCISE - CBSE Scanner
14 Videos
Similar Questions
A rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at distance x from A. The normal reaction on A is.. And on B is......
View SolutionA rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at distance x from A. The normal reaction on A is.. And on B is......
View Solution
Knowledge Check
A rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at distance x from A. The normal reaction on A is.. And on B is......
A1 . wd−xd ,wxd,B2. wd,wxdC3. wd,wx−ddD4.(xwd−x),xwdA rod of weight W is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position . The knives are at a distance d from each other . The centre of mass of the rod is at distance x from A . The normal reaction on A is
AW(d−x)xBW(d−x)dCWxdDWdxA rod of length 3m and its mass par unit length is directly proportional to the distance x from its one end . The centre of gravity of the rod from that end will be at :-
A1.5 mB2 mC2.5mD3.0 m
A rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at a distance x from A.
View SolutionA rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at distance x from A. The normal reaction on A is.. And on B is......
View SolutionA uniform rod of length 2a is placed horizontally on the edge of a table. Initially the centre of mass of the rod is at a distance a/3 from the edge. The rod is released from rest. If the rod slips after it turned an angle θ, find the coefficient of friction between the rod and the table.
View SolutionA baggage cart of weight w rests on a wall of height h. Its centre of gravity is at a distance L from the wheel as shown in fig.
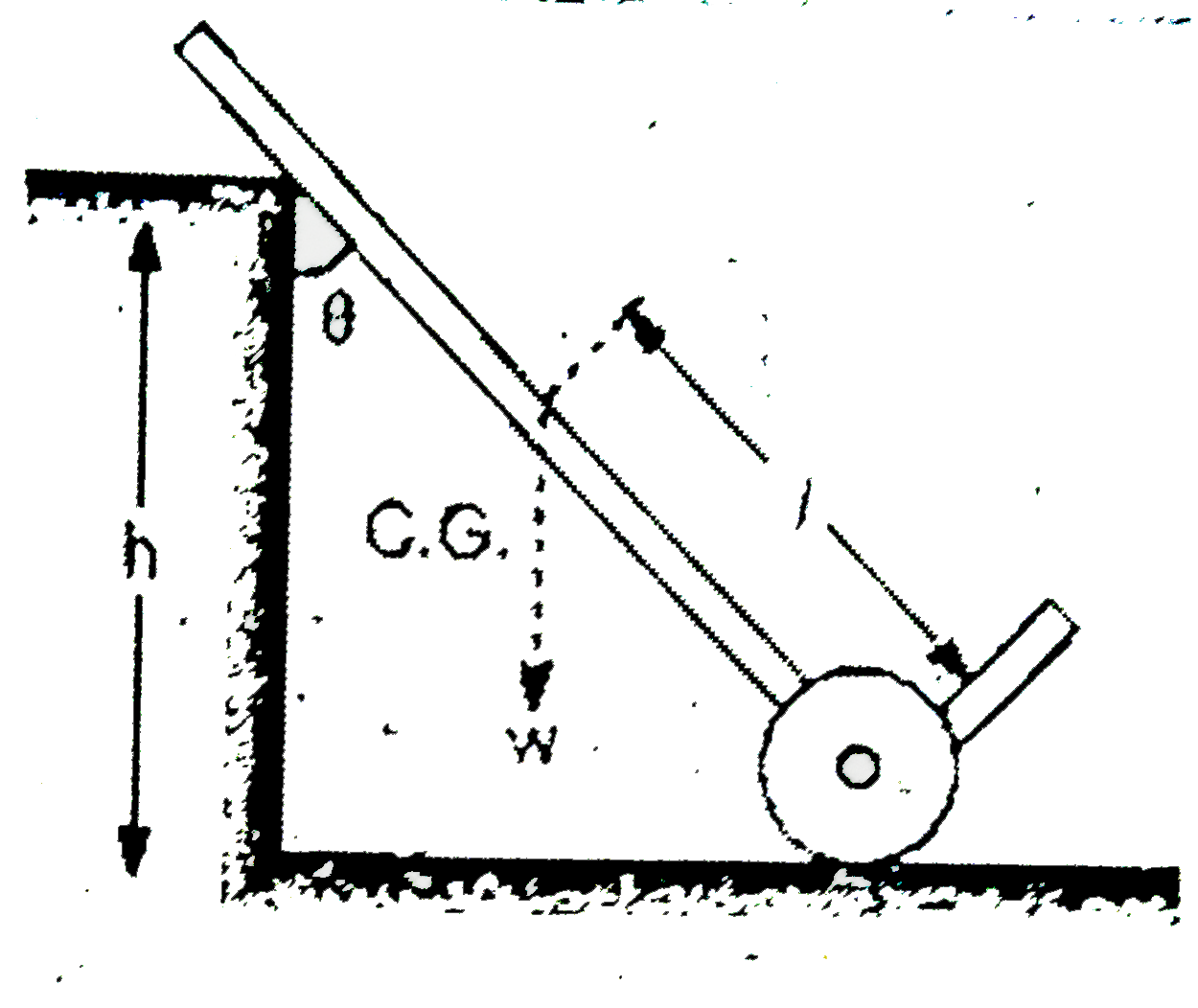
View SolutionA rod of weight w is supported by two parallel knife edges A and B and is in equilibrium in a horizontal position. The knives are at a distance d from each other. The centre of mass of the rod is at a distance x from A.
View Solution
