A rolling body of mass m=4kg, radius R and radius of gyration k=R/√3 is placed as a plank which moves with an acceleration a0=1ms−2. Find the frictional force acting on body if it rolls without sliding. (in N).
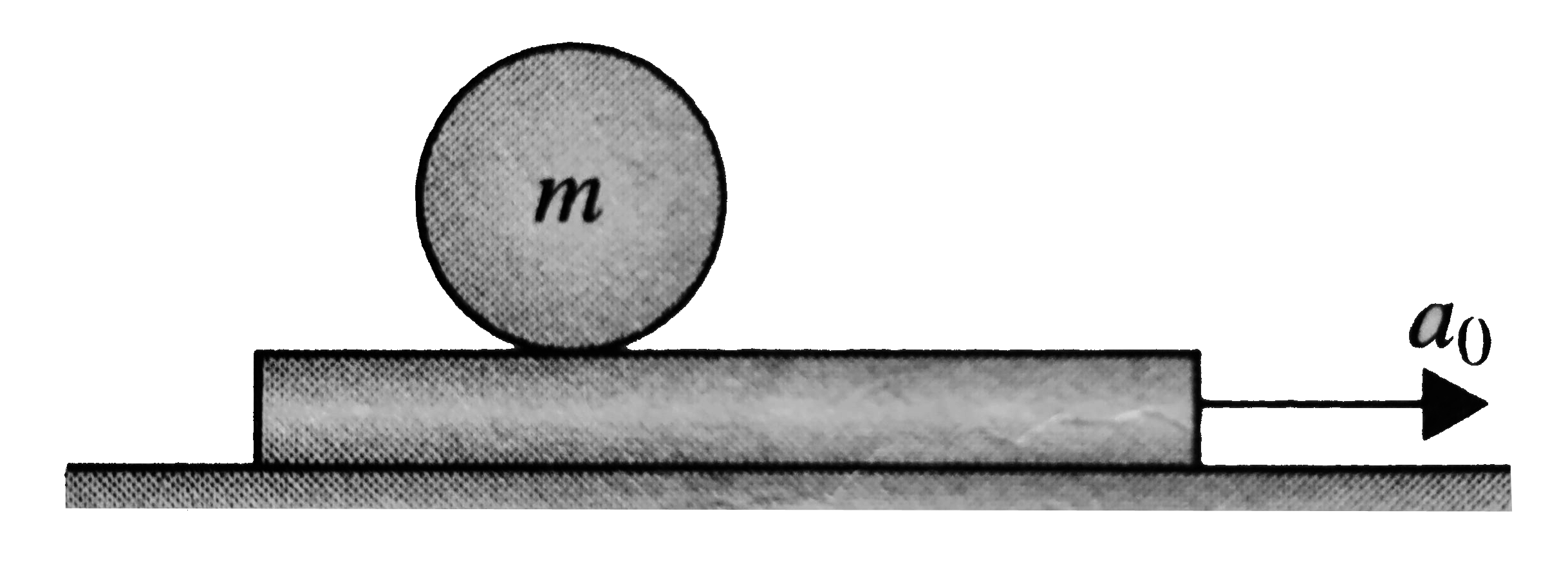
The correct Answer is:1
FBD: let the static friction fs act towards right. It pushes the body with an acceleration a say and rotate the body with an angular acceleration α say. The other forces actin onthe body are N↑ and mg↑
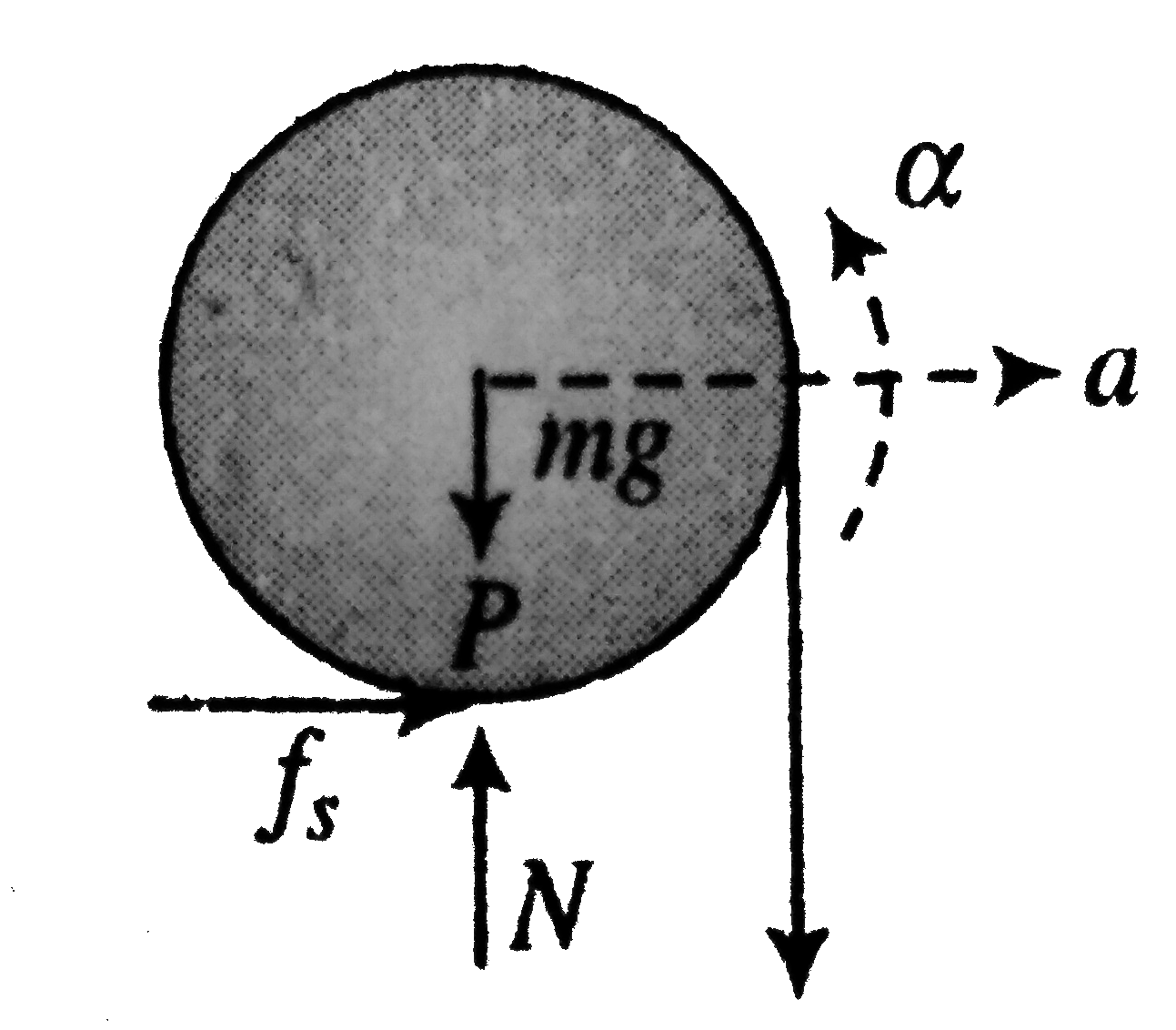
Force equation
fs=ma........i
N=mg..........ii
Torque equation
τC=fsR=mk2α ...........iii
Rolling aP=a0 where aP=a+Rα
a+rα=a0...........iv,
substituting a from eqn i α from eqn iii in eqn iv we have fsm+RfsRmK2=a0
fs=ma01+R2k2=4×1(1+3)=1N
Topper's Solved these Questions
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICSChapter:RIGID BODY DYNAMICS 2Exercise:Fill In The Blanks
Explore 7 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICSChapter:RIGID BODY DYNAMICS 2Exercise:True/False
Explore 4 Videos
- RIGID BODY DYNAMICS 2Book:CENGAGE PHYSICSChapter:RIGID BODY DYNAMICS 2Exercise:Linked Comprehension
Explore 71 Videos
- RIGID BODY DYNAMICS 1Book:CENGAGE PHYSICSChapter:RIGID BODY DYNAMICS 1Exercise:Integer
Explore 11 Videos
- SOUND WAVES AND DOPPLER EFFECTBook:CENGAGE PHYSICSChapter:SOUND WAVES AND DOPPLER EFFECTExercise:Integer
Explore 16 Videos
Similar Questions
(a) A rigid body of radius of gyration k and radius R rolls without slipping down a plane inclined at an angle θ with the horizontal. Calculate its acceleration and the frictional force acting on it.
(b) If the body be in the form of a disc and θ=30∘, what will be the acceleration and the frictional force acting on it.
View Solution
A tangential force F acts at the top of a thin spherical shell of mass m and radius R. Find the acceleration of the shell if it rolls without slipping.
View Solution
Knowledge Check
A body of mass 2 kg is placed in a rough surface. When a force of 60 N is applied on it, it starts moving with an acceleration of 15m/s2. Find the friction force acting on it.
A10NB20NC30ND40NOn the horizontal surface of plank a block of mass 1 kg is placed (μ=0.6) which is stationary w.r.t. plank. Plank is moving with acceleration 5m/s2, then the frictional force on the block will be
A5NB6NC5.88ND8NA rigid body of radius R and radius of gyration k rolls without slipping along a horizontal plane. Then, the ratio of its rotational energy to total energy is
Ak2R2Bk2k2+R2CR2k2DR2k2+R2
A body of mass 2 kg is placed on a horizontal surface having kinetic friction 0.4 and static friction 0.5. If the force applied on the body is 2.5 N, then the frictional force acting on the body will be
View SolutionA body of mass 2kg is placed on a horizontal surface having kinetic friction 0.4 and static friction 0.5 . If the force applied on the body is 2.5N , then the frictional force acting on the body will be
View SolutionThe mass m is placed on a body of mass M. There is no friction. The force F is applied on M on it moves with acceleration a. Then, the force on the top body is
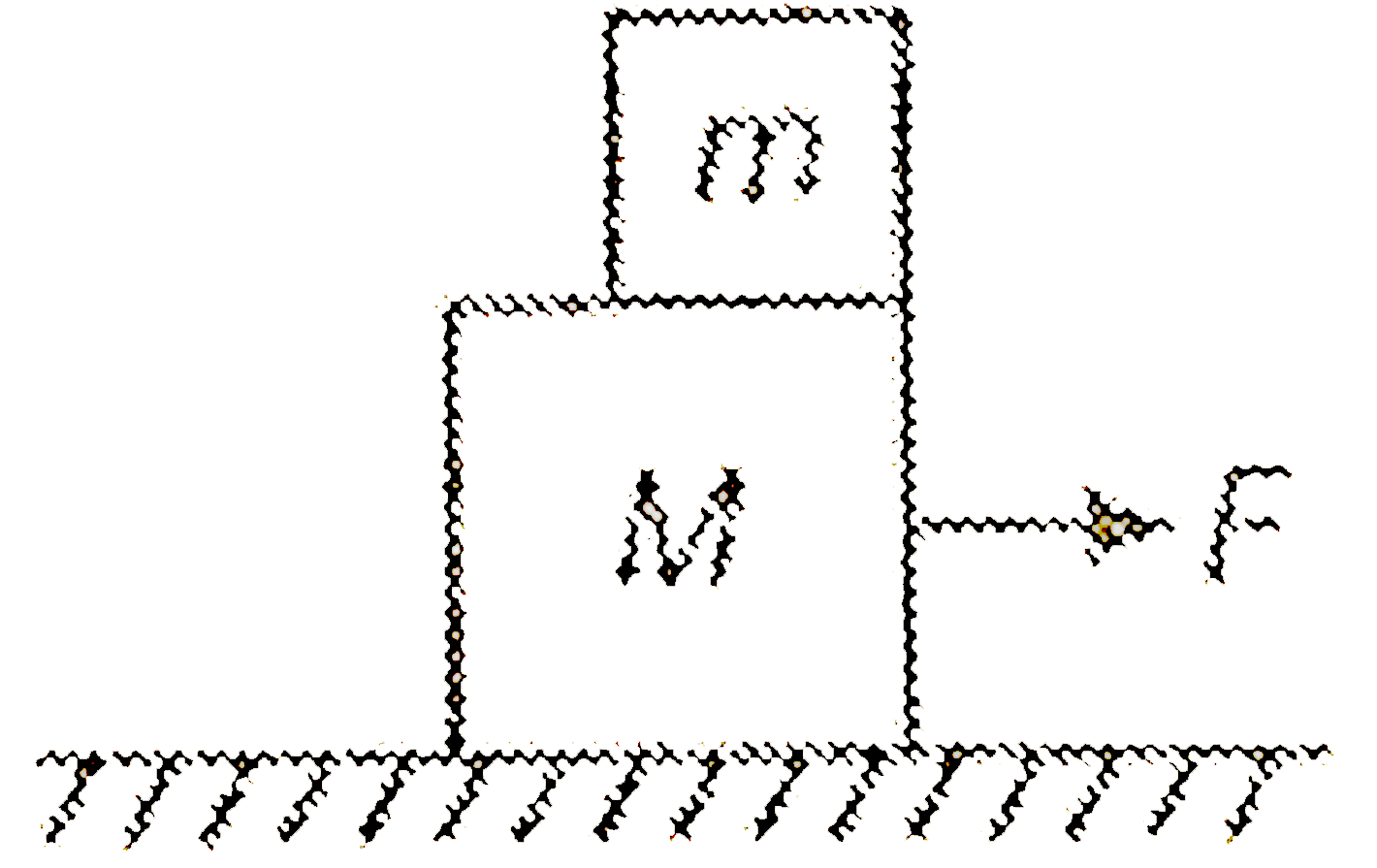
View SolutionA body of mass 20 kg is kept initially at rest. A force of 80 N is applied on the body then the acceleration produced in the body is 3m/s2, force of friction acting on the body is :
View SolutionA block of mass m=2kg is placed in equilibrium on a moving plank accelerating with a=4m/s2 . If coefficient of friction between plank and block μ=0.2 . The friction force acting on the block is:
View Solution
