A sand bag of mass m is hanging from a light spring of stiffness k. Find the elongation of the spring. If we pull the sand bag down by an additional distance x and release it, find its acceleration and maximum velocity of block.
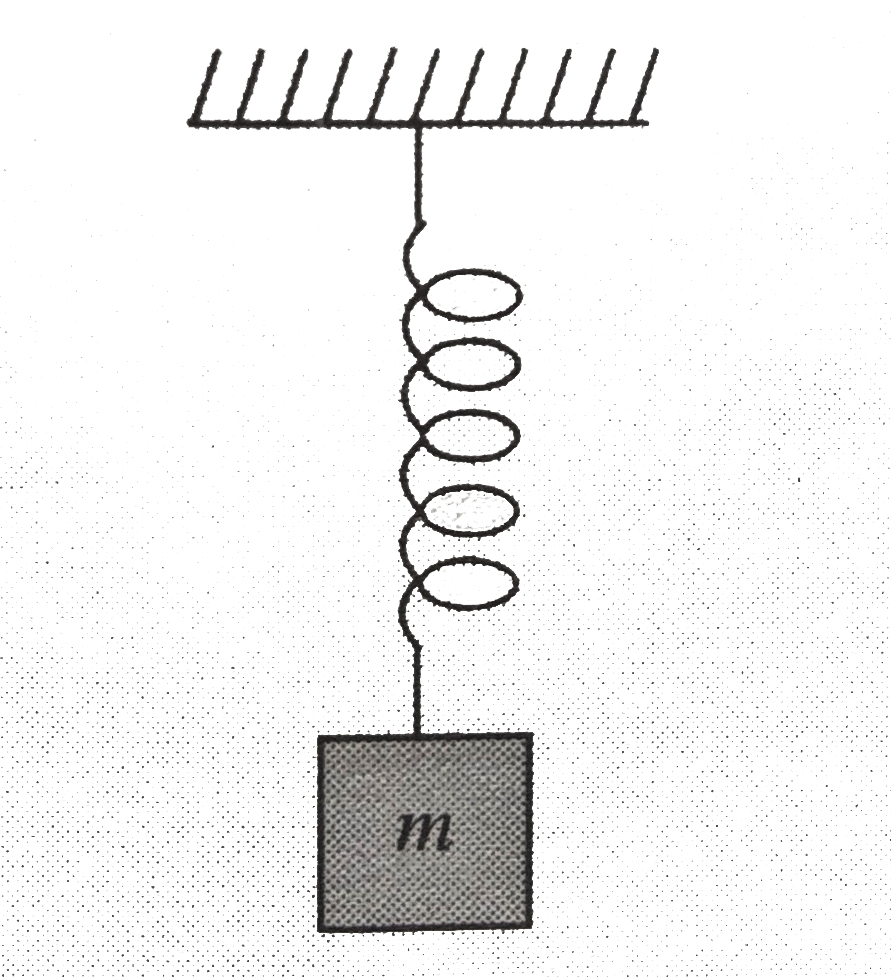
Since and downward force =
If we apply addition force to pull it down by distance x, then FBD
Upward forces are =
Downward force =
If we remove from F then
Maximum acceleration,
Maximum velocity:
.
Topper's Solved these Questions
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Subjective15videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Single Correct76videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 1EXERCISE - Exercise 6.319videos
NEWTON'S LAWS OF MOTION 1
- BOOK - CENGAGE PHYSICSCHAPTER - MISCELLANEOUS VOLUME 2EXERCISE - INTEGER_TYPE10videos
MISCELLANEOUS VOLUME 2
- BOOK - CENGAGE PHYSICSCHAPTER - NEWTON'S LAWS OF MOTION 2EXERCISE - Integer type1videos
NEWTON'S LAWS OF MOTION 2
Similar Questions
Knowledge Check
- Question 1 - Select One
A mass M is suspended from a light spring. An additional mass m added to it displaces the spring further by distance x then its time period is
AT=2π√mgx(M+m)BT=2π√(M+m)xmgCT=2π√(M+m)xMgDT=2π√Mgmg - Question 2 - Select One
Two identicle particles A and B, each of mass m, are interconnected by a spring of stiffness k. If particle B experiences a forec F and the elongation of the spring is x, the acceleration of particle B relative to particle A is equal to
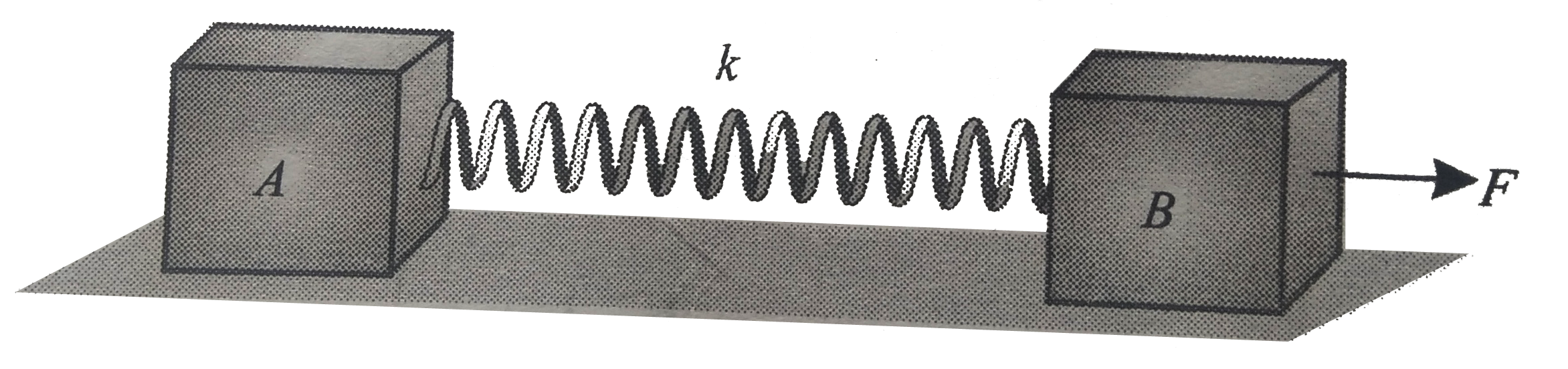 AF2mBF−kxmCF−2kxmDkxm
AF2mBF−kxmCF−2kxmDkxm - Question 3 - Select One
A disc of mass m is connected with an ideal spring of stiffness k. If it is released from rest, it rolls without sliding on an inclination plane. The maximum elongation of the spring is:
 AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk
AmgsinθkB2mgsinθ3kC3mgsinθkD2mgsinθk
Two blocks A and B of mass m and 2m respectively are connected by a light spring of force constant k. They are placed on a smooth horizontal surface. Spring is stretched by a length x and then released. Find the relative velocity of the blocks when the spring comes to its natural length
 View Solution
View SolutionA block of mass m fitted with a light spring of stiffness k and natural length l0 kept on a smooth radial groove made on a disc rotaing with a constant angular axis . If the block is released slowly . Find the maximum elongation of the spring
 View Solution
View SolutionTwo blocks of masses m1 and m2, connected by a weightless spring of stiffness k rest on a smooth horizontal plane as shown in Fig. Block 2 is shifted a small distance x to the left and then released. Find the velocity of centre of mass of the system after block 1 breaks off the wall.
 View Solution
View SolutionA spring mass system is hanging from the ceiling of an elevator in equilbrium Elongation of spring is l. The elevator suddenly starts accelerating downwards with acceleration g/3 find.
(a) the frequency and
(b) the amplitude of the resulting SHM
 View Solution
View SolutionA smooth block of mass m is connected with a spring of stiffness k(=20Nm−1 )and natural length l0=0.25m. If the block is pulled such that the new length of the spring becomes l=0.3m, find the acceleration of the block at the moment when it is released from given positon.
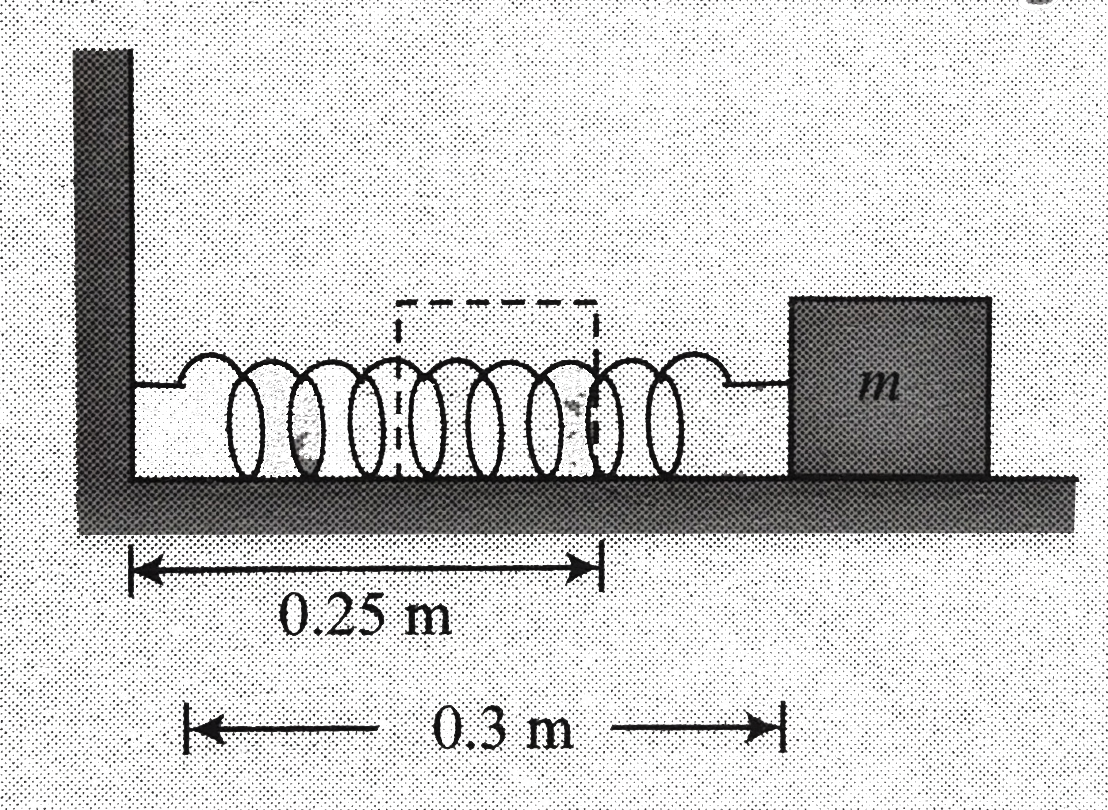 View Solution
View Solution


