A satellite moves in elliptical orbit about a planet. The maximum and minimum velocities of satellites are3×104ms and 1×103ms respectively What is the minimum distance of satellite from planet if maximum distance is 4×104km ?
4×103km
3×103km
43×103km
1×103km
To solve the problem, we will use the principle of conservation of angular momentum. The key points to remember are:
1. In an elliptical orbit, the maximum velocity occurs at the minimum distance (periapsis) and the minimum velocity occurs at the maximum distance (apoapsis).
2. The angular momentum L of the satellite is conserved, which means:
L=m⋅v⋅r
where m is the mass of the satellite, v is its velocity, and r is the distance from the planet.
Given:
- Maximum velocity vmax=3×104m/s
- Minimum velocity vmin=1×103m/s
- Maximum distance rmax=4×104km=4×107m (converting kilometers to meters)
We need to find the minimum distance rmin.
1. Set up the conservation of angular momentum equation:
Since angular momentum is conserved, we can write:
m⋅vmax⋅rmin=m⋅vmin⋅rmax
The mass m of the satellite cancels out:
vmax⋅rmin=vmin⋅rmax
2. Rearrange the equation to solve for rmin:
rmin=vmin⋅rmaxvmax
3. Substitute the known values:
rmin=(1×103m/s)⋅(4×107m)3×104m/s
4. Calculate rmin:
rmin=4×10103×104
rmin=43×106m
rmin≈1.33×106m
5. Convert rmin back to kilometers:
rmin≈1.33×106m=1.33km
Final Answer:
The minimum distance of the satellite from the planet is approximately 1.33 km.
2. The angular momentum L of the satellite is conserved, which means:
L=m⋅v⋅r
where m is the mass of the satellite, v is its velocity, and r is the distance from the planet.
- Maximum velocity vmax=3×104m/s
- Minimum velocity vmin=1×103m/s
- Maximum distance rmax=4×104km=4×107m (converting kilometers to meters)
Since angular momentum is conserved, we can write:
m⋅vmax⋅rmin=m⋅vmin⋅rmax
The mass m of the satellite cancels out:
vmax⋅rmin=vmin⋅rmax
rmin=vmin⋅rmaxvmax
rmin=(1×103m/s)⋅(4×107m)3×104m/s
rmin=4×10103×104
rmin=43×106m
rmin≈1.33×106m
rmin≈1.33×106m=1.33km
The minimum distance of the satellite from the planet is approximately 1.33 km.
Topper's Solved these Questions
Similar Questions
A satellite moves in an elliptical orbit about a planet. The maximum and minimum velocities of the satellite are 3×104 m / s and 1×103ms, respectively. If the minimum. distance of the satellite from the planet is mn×103 km then find (m−n). (The maximum distance of the satellite from the planet is 4×104 km.
View SolutionA satelite revolves around a planet in an elliptical orbit. Its maximum and minimum distances from the planet are 1.5×107 m and 0.5×107 m respectively. If the speed of the satellite at the farthest point be 5×103 m/s, calculate the speed at the nearest point.
View Solution
Knowledge Check
A satellite moving in an elliptical orbit around the earth as shown. The minimum and maximum distance of the satellite from earth are 3 units and 5 units respectively. The distance of satellite from earth when it is at P is .........unit
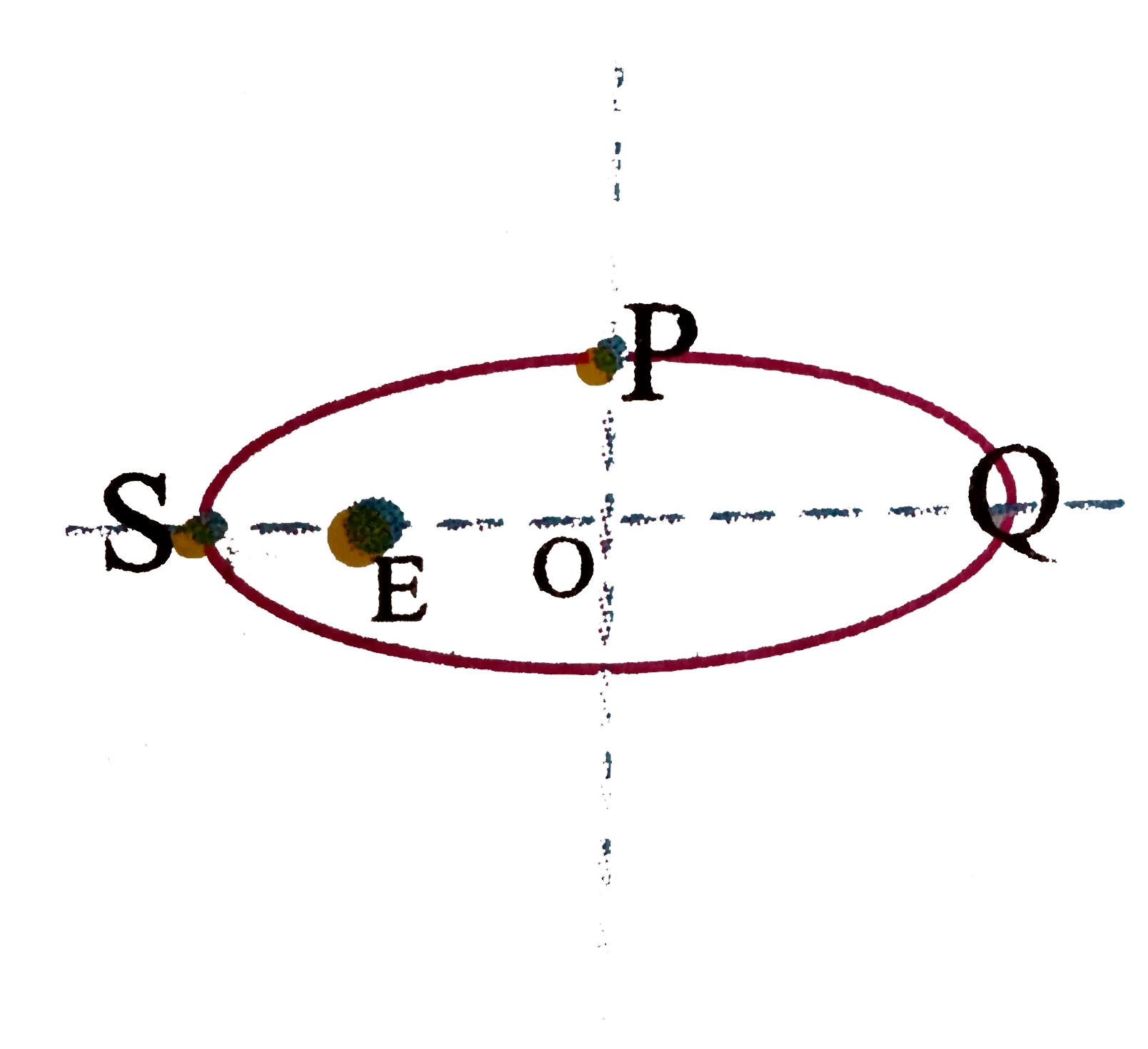 A4B3C3.75D6
A4B3C3.75D6A satellite moves in an elliptical orbit about the earth. The minimum and maximum distance of the satellite from the centre of earth are 7000km and 8750km respectively. For this situation, mark the correct statements (s). [Take Me=6×1024kg]
AThe maximum speed of the satellite during its motion is 5.64km/sBThe minimum speed of the satellite during its motion is 4.51km/sCThe length of major axis of orbit is 15750kmDnone of the aboveThe maximum and minimum distance of a comet from the sun are 14×109 m and 7×107m respectively. If the maximum velocity of the comet is 5×102 km/sec, its minimum velcity will be:-
A2.5km/secB4km/secC6km/secD8km/sec
What will be the escape speed from a planet of mass 6×1016 kg and radius 3.6×104 m ?
View SolutionA planet of mass m revolves in elliptical orbit around the sun of mass M so that its maximum and minimum distance from the sun equal to ra and rp respectively. Find the angular momentum of this planet relative to the sun.
View SolutionA satellite is in an elliptical orbit around a planet P. It is observed that the velocity of the satellite when it is farthest from the planet is 6 times less than that when it is closest to the planet . The ratio of distances between the statellite and the planet at closest and farthest points is :
View SolutionA planet of mass m moves around the Sun of mass Min an elliptical orbit. The maximum and minimum distance of the planet from the Sun are r1 and r2, respectively. Find the relation between the time period of the planet in terms of r1 and r2.
View SolutionA planet of mass m is moving in an elliptical orbit about the sun (mass of sun = M). The maximum and minimum distances of the planet from the sun are r1 and r2 respectively. The period of revolution of the planet wil be proportional to :
View Solution
