A screen is at distance D=80 cm form a diagram having two narrow slits S1 and S2 which are d=2 mm apart.
Slit S1 is covered by a transparent sheet of thickness
t1=2.5μm slit S2 is covered by another sheet of thickness
t2=1.25μm as shown if Fig. 2.52.
Both sheets are made of same material having refractive index μ=1.40
Water is filled in the space between diaphragm and screen. A monochromatic light beam of wavelength λ=5000Å is incident normally on the diaphragm.
Assuming intensity of beam to be uniform, calculate ratio of intensity of C to maximum intensity of interference pattern obtained on the screen (μw=4/3)
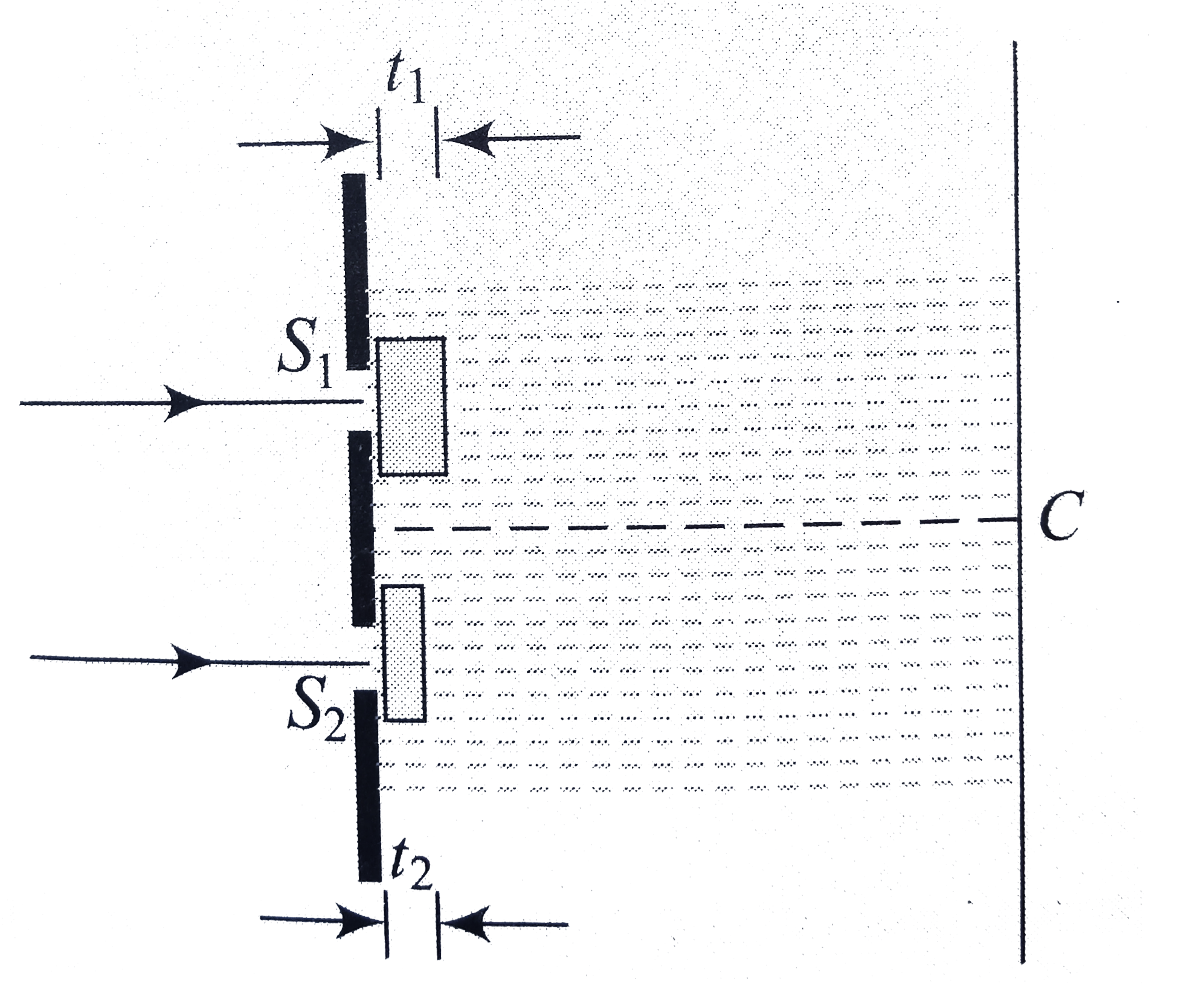
Path difference at C.
Δx=t1(μ−1)−t2(μ−1)
μ(t1−t2)−(t1−t2)
(t1−t2(t1−(t2)
=(2.5−1.25)(1.4×34×101)=1.25×240=2.5400
⇒Δx=116μm
ϕ=2πλΔx=2π×45000×3×10−10×116×10−6=π3
Imax=4I0
Intensity at C, Ic=2I0(1+cos(π)3)=3I0
∴ Required raito =ICImax=34
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Solved Examples
10 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Exercise 2.1
12 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
A screen is at distance D=80 cm form a diagram having two narrow slits S1 and S2 which are d=2 mm apart.
Slit S1 is covered by a transparent sheet of thickness
t1=2.5μm slit S2 is covered by another sheet of thickness
t2=1.25μm as shown if Fig. 2.52.
Both sheets are made of same material having refractive index μ=1.40
Water is filled in the space between diaphragm and screen. A monochromatic light beam of wavelength λ=5000Å is incident normally on the diaphragm.
Assuming intensity of beam to be uniform, calculate ratio of intensity of C to maximum intensity of interference pattern obtained on the screen (μw=4/3)

View SolutionMonochromatic light of wavelength 600 nm is used in a Young's double slit experiment. One of the slits is covered by a transperent sheet of thickness 1.8×10−5m made of a material refrective index 1.6. How many fringes will shift due to the introduction of the sheet ?
View Solution
Knowledge Check
Monichromatic lightof wavelenght 600 nm is used ina Young's double slilt experient. One of the slits is covered by a transparent sheet of thicknes 1.8×10−5 m made of a material of refractive index 1.6. How many fringe will shift due to the introduction of the sheet?
Acos2ϕBcos2ϕ2Ccos2ϕ2Dcos2ϕ4Two slits S1 and S2 illuminated by a white light source give a white central maxima. A transparent sheet of refractive index 1.25 and thickness t1 is placed in front of S1. Another transparent sheet of refractive index 1.50 and thickness t2 is placed in front of S2. If central maxima is not effected, then ratio of the thickness of the two sheets will be :
A1:2B2:1C1:4D4:1Monichromatic lightof wavelenght 600 nm is used ina Young's double slilt experient. One of the slits is covered by a transparent sheet of thicknes 1.8×10−5 m made of a material of refractive index 1.6. How many fringe will shift due to the introduction of the sheet?
A18B10C20D8
Discussion Sheet S1 and S2
View SolutionIn YSDE, both slits are covered by transparent slab. Upper slit is covered by slab of R.I. 1.5 and thickness t and lower is
covered by R.I. 43 and thickness 2t, then central maxima

View SolutionIn the Young's double slit experiment , a mica slip of thickness t and refractive index μ is introduced in the ray from first source S1 . By how much distance fringes pattern will be displaced ? (d = distance between the slits and D is the distance between slits and screen)
View SolutionA plane monochromatic light falls normally on a diaphragm with two narrow slits separated by a distance d = 2.5 mm. A fringe pattern is formed on the screen placed at D = 100 cm behind the diaphragm. If one of the slits is covered by a glass plate of thickness 10μm, then distance by which these fringes will be shifted will be :
View SolutionIn young's double slit experiment, distance between the slit S1andS2 is d and the distance between slit and screen is D . Then longest wavelength that will be missing on the screen in front of S1 is
View Solution
