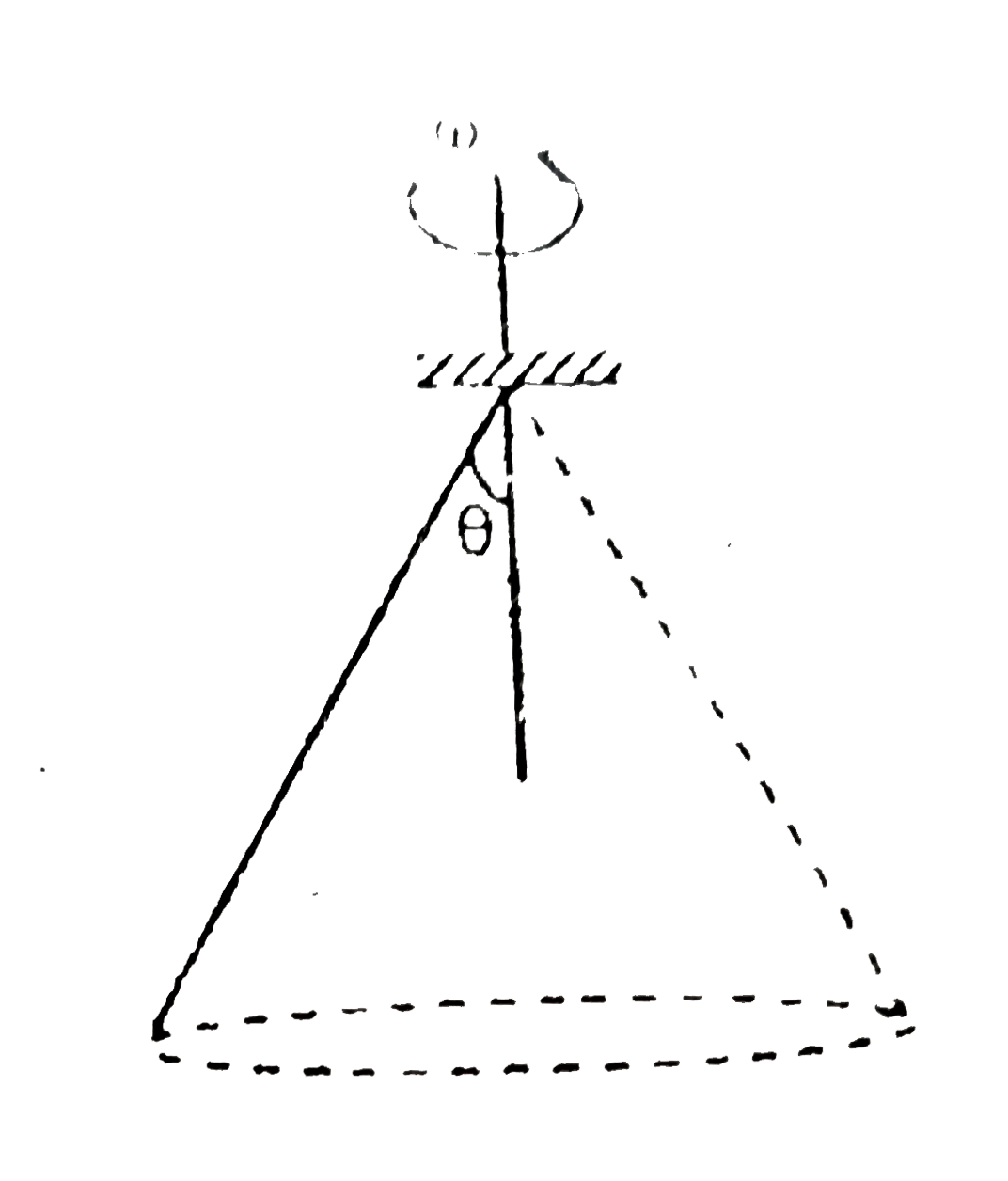
A simple pendulum of length l ils pulled aside to make an angle with the vertical. Find the magnitude of the torque of the weitht w of the bob about the point of suspension. When is the torque zero?
The correct Answer is:A, B
To find the magnitude of the torque of the weight W of the bob about the point of suspension when the pendulum is pulled aside to make an angle θ with the vertical, we can follow these steps:
Step 1: Understand the Setup
We have a simple pendulum consisting of a bob of mass m attached to a string of length L. The bob is displaced to an angle θ from the vertical.
Hint: Visualize the pendulum and identify the forces acting on the bob.
Step 2: Identify the Forces
The only force acting on the bob is its weight W, which acts vertically downward. The weight can be expressed as:
W=mg
where g is the acceleration due to gravity.
Hint: Remember that the weight acts downward regardless of the pendulum's position.
Step 3: Determine the Torque
Torque (τ) about the point of suspension is given by the formula:
τ=r×F
where r is the distance from the pivot point to the point of application of the force, and F is the force.
In this case, the torque due to the weight of the bob is:
τ=W⋅D
where D is the perpendicular distance from the line of action of the weight to the pivot point.
Hint: The torque is maximized when the force is applied at the maximum distance from the pivot.
Step 4: Calculate the Perpendicular Distance D
The perpendicular distance D can be found using trigonometry. When the bob is at an angle θ, the perpendicular distance from the weight to the pivot is:
D=Lsinθ
Hint: Use the sine function to relate the angle to the opposite side of the triangle formed by the pendulum.
Step 5: Substitute D into the Torque Formula
Now substituting D into the torque equation, we get:
τ=W⋅(Lsinθ)
Thus, the magnitude of the torque is:
τ=WLsinθ
Hint: This equation shows how torque depends on both the weight of the bob and the angle θ.
Step 6: Determine When the Torque is Zero
The torque will be zero when:
sinθ=0
This occurs when θ=0 (i.e., when the pendulum is in the vertical position).
Hint: Think about the physical meaning: when the pendulum is vertical, the weight acts directly downward through the pivot, resulting in no torque.
Final Answer
The magnitude of the torque of the weight W of the bob about the point of suspension is:
τ=WLsinθ
The torque is zero when the pendulum is in the vertical position, i.e., θ=0.
We have a simple pendulum consisting of a bob of mass m attached to a string of length L. The bob is displaced to an angle θ from the vertical.
The only force acting on the bob is its weight W, which acts vertically downward. The weight can be expressed as:
W=mg
where g is the acceleration due to gravity.
Torque (τ) about the point of suspension is given by the formula:
τ=r×F
where r is the distance from the pivot point to the point of application of the force, and F is the force.
τ=W⋅D
where D is the perpendicular distance from the line of action of the weight to the pivot point.
The perpendicular distance D can be found using trigonometry. When the bob is at an angle θ, the perpendicular distance from the weight to the pivot is:
D=Lsinθ
Now substituting D into the torque equation, we get:
τ=W⋅(Lsinθ)
Thus, the magnitude of the torque is:
τ=WLsinθ
The torque will be zero when:
sinθ=0
This occurs when θ=0 (i.e., when the pendulum is in the vertical position).
The magnitude of the torque of the weight W of the bob about the point of suspension is:
τ=WLsinθ
The torque is zero when the pendulum is in the vertical position, i.e., θ=0.
Topper's Solved these Questions
- ROTATIONAL MECHANICSBOOK - HC VERMACHAPTER - ROTATIONAL MECHANICSEXERCISE - Objective 2
15 Videos
- REST AND MOTION : KINEMATICSBOOK - HC VERMACHAPTER - REST AND MOTION : KINEMATICSEXERCISE - Exercises
51 Videos
- SIMPLE HARMONIC MOTIONBOOK - HC VERMACHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - Exercises
58 Videos
Similar Questions
A pendulum bob of mass m and length L is released from angle θ with the vertical. Find
(a) the speed of the bob at the bottom of the swing and
(b) tension in the string at that time
View SolutionA particle of mass m is projecte dwilth speed u at an angle θ with the horizontal. Find the torque of the weight of the particle about the point of projection when the particle is at the highest point.
View Solution
Knowledge Check
A conical pendulum of length L makes an angle θ with the vertical. The time period will be
 A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√Lgtanθ
A2π√lcosθgB2π√LgcosθC2π√LtanθγD2π√LgtanθA simple pendulum of length l and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθA simple pendulum of length I and mass (bob) m is suspended vertically. The string makes an angle θ with the vertical. The restoring force acting on the pendulum is
AmgtanθB−mgsinθCmgsinθD−mgcosθ
A nail is located at a certain distance vertically below the point of suspension of a simple pendulum. The pendulum bob is released from a position where the string makes an angle of 60∘ with the vertical. Calculate the distance of nail from the point of suspension such that the bob will just perform revolutions with the nail as centre. Assume the length of the pendulum to be one meter.
View SolutionA pendulum consists of a small mass m at the end of a string of length L. The pendulum is pulled aside making an angle θ with the vertical and released. Taking the suspension point as the axis, at the instant of release
View SolutionA simple pendulum of mass m and length L is held in horizontal position. If it is released from this position, then find the angular momentum of the bob about the point of suspension when it is vertically below the point of suspension.
View SolutionA rod of mass m and length l is rotating about a fixed point in the ceiling with an angular velocity ω as shown in the figure. The rod maintains a constant angle θ with the vertical.
What will be the horizontal component of angular momentum of the rod about the point of suspension in terms of m, ω, l and θ.
View SolutionA simple pendulum of length l and mass m is initially at its lowest position. It is given the minimum horizontal speed necessary after to move in a circular path about the point of suspension. The tension in the string at the lowest positive of the bob is
View Solution
