The surface density (mass/area) of a circular disc of radius a depends on the distance from the centre as $ρ (r) = A + B r .$ Find its moment of inertia about the line perpendicular to the plane of the disc through its centre.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, B, D

The surface density of a circular disc of radius a depends upon the distance from the centre as P(r)=A+Br.
Therefore the mass of the ring of radius r will be
$θ = (a + B r) \times 2 π r \times d r$

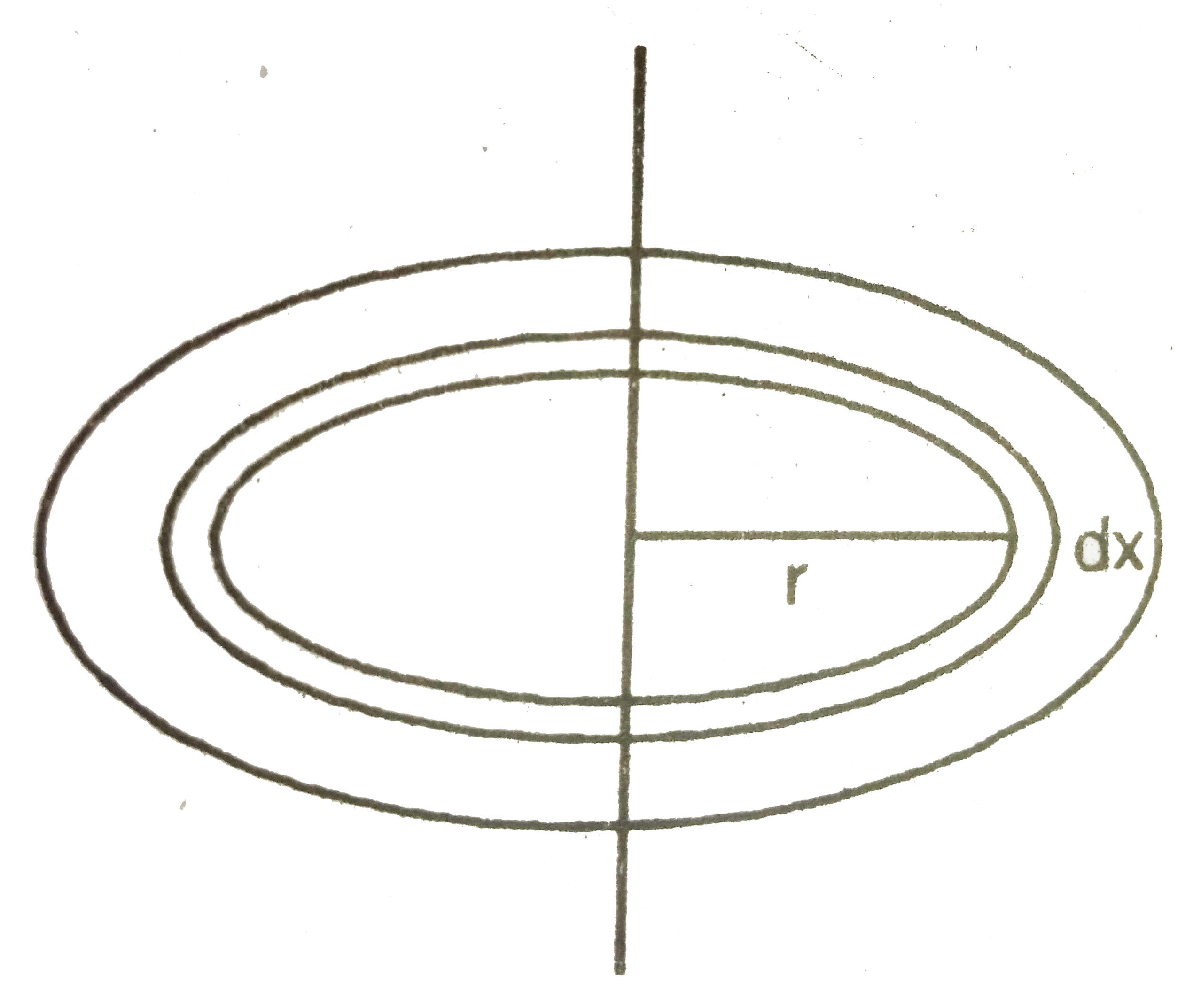
Therefore moment of inertila about the centre
$= \int_{a}^{0} (A + B r) 2 π r . d r \times r^{2}$
$= a \int_{a}^{02} π A r^{3} d r + \int_{a}^{0} 2 π B r^{4} d r$
$= {[2 π A (\frac{r^{4}}{4}) + 2 π B (\frac{r^{5}}{5})]}_{a}^{0}$
$= 2 π (\frac{A a^{4}}{4} + \frac{B a^{5}}{5})$

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The moment of inertia of a uniform semicircular disc of mass $M$ and radius $r$ about a line perpendicular to the plane of the disc through the center is
A $\frac{1}{2} M r^{2}$
B $M t^{2}$
C $\frac{2}{5} M r^{2}$
D $\frac{1}{4} M r^{2}$
Question 2 - Select One
The diagram shows a uniform disc of mass $M$ & radius ' $a$ ', if the moment of inertia of the disc about the axis $X Y$ is $I$ , its moment of inertia about an axis through $O$ and perpendicular to the plane of the disc is

A $\frac{4}{3} M a^{2}$
B $I + \frac{1}{4} M a^{2}$
C2I
D $\frac{I}{2}$
Question 3 - Select One
The M.I. of a uniform semicircular disc of mass M and radius R about a line perpendicular to the plane of the disc and passing through the centre is
A $\frac{1}{2} M R^{2}$
B $\frac{1}{4} M R^{2}$
C $M R^{2}$
D $\frac{3}{4} M R^{2}$

HC VERMA-ROTATIONAL MECHANICS-Exercises

Home
Ask Doubt
Profile