A small body of density ρ' is dropped from rest at a height h into a lake of density ρ, where ρ>ρ’. Which of the following statements is/are correct if all dissipative effects are neglected (neglect viscosity)?
The speed of the body just entering the lake is √2gh
The body in the lake experiences upward acceleration equal to {(ρ/ρ')−1}g
The maximum depth to which the body sinks in the lake is hρ'(ρ−ρ')
The body does not come back to the surface of the lake.
The correct Answer is:A, B, C
To solve the problem step by step, we will analyze the motion of a small body of density ρ′ dropped from a height h into a lake of density ρ (where ρ>ρ′). We will determine the correct statements regarding the motion of the body in the lake, neglecting all dissipative effects.
Step 1: Determine the velocity just before entering the lake
The body is dropped from rest, so its initial velocity u=0. We can use the equation of motion to find the velocity v just before it enters the lake:
v2=u2+2gh
Substituting u=0:
v2=0+2gh⟹v=√2gh
Step 2: Analyze the forces acting on the body in the lake
Once the body enters the lake, two forces act on it:
1. The weight of the body W=ρ′Vg (where V is the volume of the body).
2. The buoyant force Fb=ρVg.
The net force acting on the body when it is submerged is:
Fnet=W−Fb=ρ′Vg−ρVg=Vg(ρ′−ρ)
Since ρ′<ρ, the net force is downward.
Step 3: Determine the upward acceleration of the body
Using Newton's second law, we can express the net force in terms of acceleration a:
Fnet=ma⟹Vg(ρ−ρ′)=ρ′Va
Dividing both sides by V:
g(ρ−ρ′)=ρ′a⟹a=g(ρ−ρ′)ρ′
Step 4: Determine how deep the body will sink
To find the depth h′ to which the body sinks, we can use the kinematic equation:
v2=u2+2ah′
At the maximum depth, the final velocity v=0 (the body comes to rest momentarily), and the initial velocity u is the velocity just before entering the lake √2gh:
0=(2gh)+2(−g(ρ−ρ′)ρ′)h′
Solving for h′:
0=2gh−2g(ρ−ρ′)ρ′h′⟹h′=hρ′ρ−ρ′
Step 5: Conclusion
Based on the analysis, we can conclude the following:
1. The velocity just before entering the lake is v=√2gh.
2. The upward acceleration of the body in the lake is a=g(ρ−ρ′)ρ′.
3. The depth to which the body sinks is h′=hρ′ρ−ρ′.
4. The body will eventually rise back to the surface because it is less dense than the water.
Summary of Correct Statements
- Statement 1: Correct (velocity just before entering the lake).
- Statement 2: Correct (upward acceleration).
- Statement 3: Correct (depth of sinking).
- Statement 4: Incorrect (the body will rise back to the surface).
The body is dropped from rest, so its initial velocity u=0. We can use the equation of motion to find the velocity v just before it enters the lake:
Once the body enters the lake, two forces act on it:
1. The weight of the body W=ρ′Vg (where V is the volume of the body).
2. The buoyant force Fb=ρVg.
Using Newton's second law, we can express the net force in terms of acceleration a:
To find the depth h′ to which the body sinks, we can use the kinematic equation:
Based on the analysis, we can conclude the following:
1. The velocity just before entering the lake is v=√2gh.
2. The upward acceleration of the body in the lake is a=g(ρ−ρ′)ρ′.
3. The depth to which the body sinks is h′=hρ′ρ−ρ′.
4. The body will eventually rise back to the surface because it is less dense than the water.
- Statement 1: Correct (velocity just before entering the lake).
- Statement 2: Correct (upward acceleration).
- Statement 3: Correct (depth of sinking).
- Statement 4: Incorrect (the body will rise back to the surface).
Topper's Solved these Questions
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Multipe Correct
15 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Assertion-Reasoning
8 Videos
- FLUID MECHANICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - FLUID MECHANICSEXERCISE - Subjective
25 Videos
- DIMENSIONS & MEASUREMENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - DIMENSIONS & MEASUREMENTEXERCISE - Integer
2 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
Similar Questions
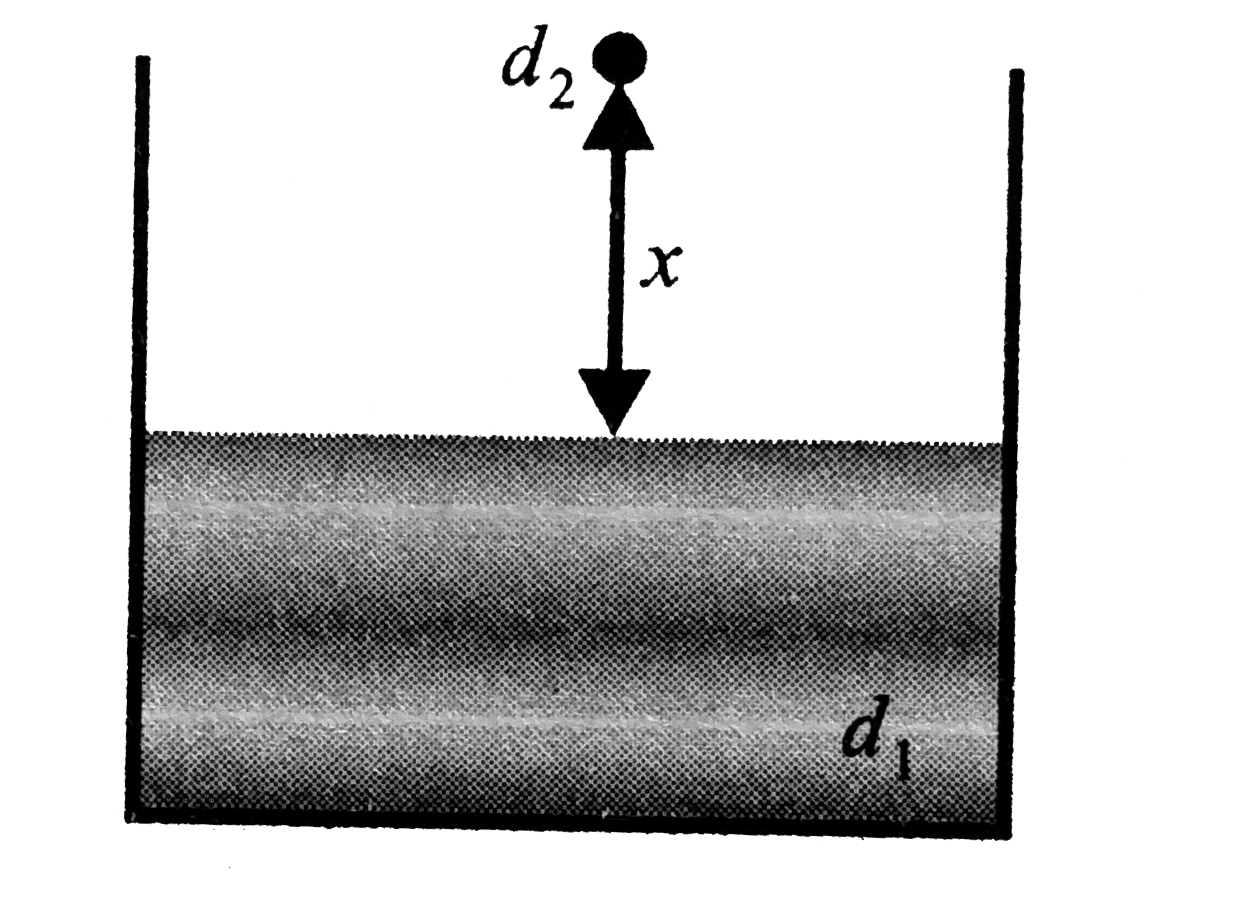
A body of density d2 is dropped from rest at a height x into a beaker having a liquid of density d1(d2>d1). Which of the following is/are true?
View SolutionA body of density ρ is dropped from a height h into a lake of density σ(σ>ρ). The maximum depth the body sinks inside the liquid is (neglect viscous effect of liquid)
View SolutionA body of density ρ is dropped from rest from height h (from the surface of water) into a lake of density of water σ(σ>ρ). Neglecting all disspative effects, the acceleration of body while it is in the take is
View SolutionAn object of density σ(<ρ) is dropped from rest at a height h into a liquid of density ρ kept in a tall vertical cylindrical container. Neglect all dissipative effects and assume that there is no spilling of the liquid.
The time taken by the object to reach the starting point (from which it is dropped) is
View SolutionA ball of density ρ is dropped from a height on the surface of a non-viscous liquid of density 2ρ. Choose the correct options.

View SolutionA liquid drop of density ρ , radius r, and surface tension σ oscillates with time period T . Which of the following expressions for T2 is correct?
View SolutionIf v be the instantaneous velocity of the body dropped from the top of a tower, when it is located at height h, then which of the following remains constant?
View SolutionA ball of density ρ0 falls from rest from a point P onto the surface of a liquid of density ρ in the time T. It enters the liquid, stops, moves up, and returns to P in a total time 3 T. neglect viscosity, surface tension and splashing find the ratio of ρρ0
View SolutionA beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
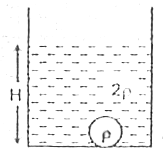
View SolutionA small ball of density ρ is placed from the bottom of a tank in which a non viscous liquid of density 2ρ is filled . Find the time taken by the ball to reach the surface of liquid and maximum height reached above the bottom of container . (Size of the ball and any type of drag force acting on the ball are negligible )
View Solution
