A beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
ρgh
12ρgh
14ρgh
18ρgh
To find the mean pressure at the walls of a beaker filled with a liquid of density ρ up to a height h, we can follow these steps:
Step 1: Understand the Pressure Distribution
The pressure at a depth x in a fluid is given by the formula:
P=ρgx
where:
- P is the pressure at depth x,
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- x is the depth from the surface of the liquid.
Step 2: Consider a Small Element of the Wall
Consider a small horizontal strip of the wall of the beaker at a height x from the bottom with a thickness dx. The perimeter of this strip is 2πr if the beaker has a circular cross-section of radius r.
Step 3: Calculate the Force on the Strip
The force dF exerted on this strip due to the pressure is given by:
dF=P⋅dA
where dA is the area of the strip. The area dA is given by:
dA=2πr⋅dx
Thus, the force on the strip becomes:
dF=(ρgx)⋅(2πr⋅dx)=2πrρgxdx
Step 4: Integrate to Find Total Force
To find the total force exerted on the wall from the surface down to the bottom of the liquid, we integrate dF from x=0 to x=h:
F=∫h02πrρgxdx
Calculating the integral:
F=2πrρg∫h0xdx=2πrρg(x22)h0=2πrρg⋅h22=πrρgh2
Step 5: Calculate the Average Pressure
The average pressure Pavg on the walls of the beaker can be found by dividing the total force by the area of the wall in contact with the liquid. The curved surface area A of the cylinder (beaker) is:
A=2πrh
Thus, the average pressure is:
Pavg=FA=πrρgh22πrh=ρgh2
Final Answer
The mean pressure at the walls of the beaker is:
Pavg=ρgh2
The pressure at a depth x in a fluid is given by the formula:
P=ρgx
where:
- P is the pressure at depth x,
- ρ is the density of the liquid,
- g is the acceleration due to gravity,
- x is the depth from the surface of the liquid.
Consider a small horizontal strip of the wall of the beaker at a height x from the bottom with a thickness dx. The perimeter of this strip is 2πr if the beaker has a circular cross-section of radius r.
The force dF exerted on this strip due to the pressure is given by:
dF=P⋅dA
where dA is the area of the strip. The area dA is given by:
dA=2πr⋅dx
Thus, the force on the strip becomes:
dF=(ρgx)⋅(2πr⋅dx)=2πrρgxdx
To find the total force exerted on the wall from the surface down to the bottom of the liquid, we integrate dF from x=0 to x=h:
F=∫h02πrρgxdx
Calculating the integral:
F=2πrρg∫h0xdx=2πrρg(x22)h0=2πrρg⋅h22=πrρgh2
The average pressure Pavg on the walls of the beaker can be found by dividing the total force by the area of the wall in contact with the liquid. The curved surface area A of the cylinder (beaker) is:
A=2πrh
Thus, the average pressure is:
Pavg=FA=πrρgh22πrh=ρgh2
The mean pressure at the walls of the beaker is:
Pavg=ρgh2
Similar Questions
Consider a regular tank of size (l×b) filled with a liquid of density ρ to a height H as shown in figure. Find the force at the base and on the wall of the tank.

View SolutionAn observer can see through a pin-hole the top end of a thin rod of height h, placed as shown in the figure. The beaker height is 3h and its radius h. When the beaker is filled with a liquid up to a height 2h, he can see the lower end of the rod. Then the refractive index of the liquid is
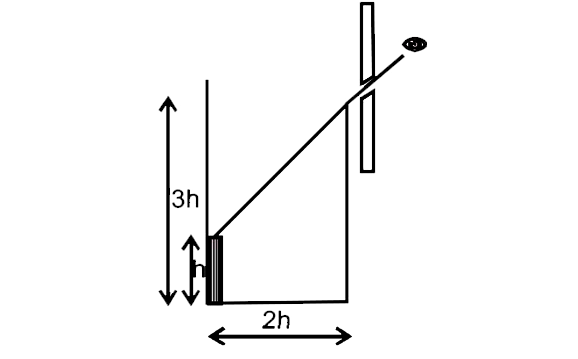
View Solution
Knowledge Check
The pressure inside a liquid of density ρ at a depth h is :
AhρgBhρgChρgDhρ
A ball of mass m and density ρ is immersed in a liquid of density 3ρ at a depth h and released. To what height will the ball jump up above the surface of liqud ? (neglect the reistance of water and air).
View SolutionA beaker containing a liquid of density ρ moves up with an acceleration a. The pressure due to the liquid at a depth h below the free surface of the liquid is.
View SolutionIf liquid of density ρ is filled in an open cylindrical container to height h, then pressure at bottom
View SolutionA small coin is resting on the bottom of a beaker filled with a liquid. A ray of light from the coin travels up to the surface of the liquid and moves along its surface (see figure ).
How fast is the light travelling in the liquid ?
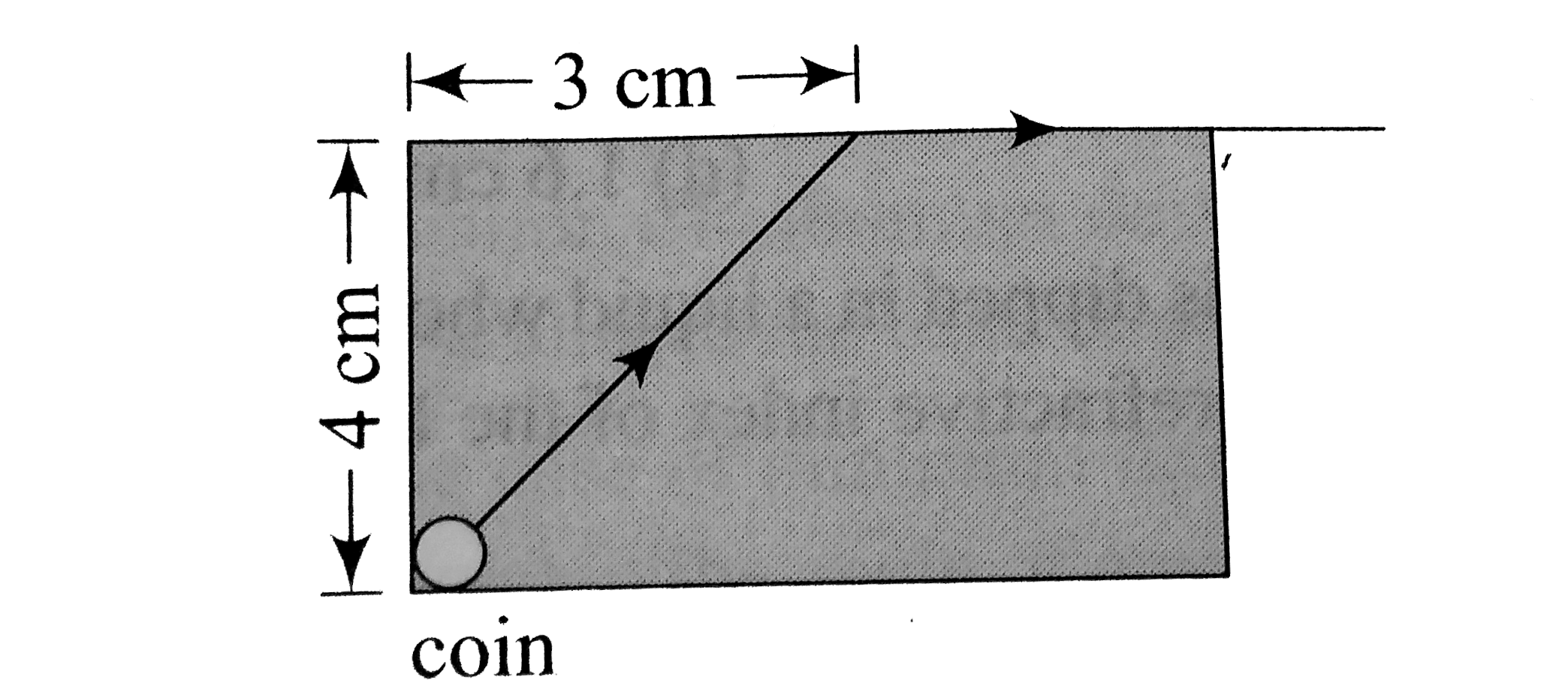
View Solution
Artificial satellites go round the earth in their respective orbits. Astronauts are able to float in side the satellite. Inside space of the satellite could be treated as gravity free space. If a beaker filled with a liquid upto height h having a small hole in the bottom is placed in such a satellite, you will wonder te liquid does not come out of the hole. You have to squeeze or force the liquid to come out. suppose a constant pressure p is applied on the liquid through piston to force it out through the hole. Out side pressure could be neglected in comparison with p Cross-sectional area of beaker is too greater than that of hole (A>>a)
View SolutionA metal coin at the bottom of a beaker filled with a liquid of refractive index 4/3 to height of 6cm. To an observer looking from above the surface of the liquid, coin will appear at a depth of (consider paraxial rays).
View Solution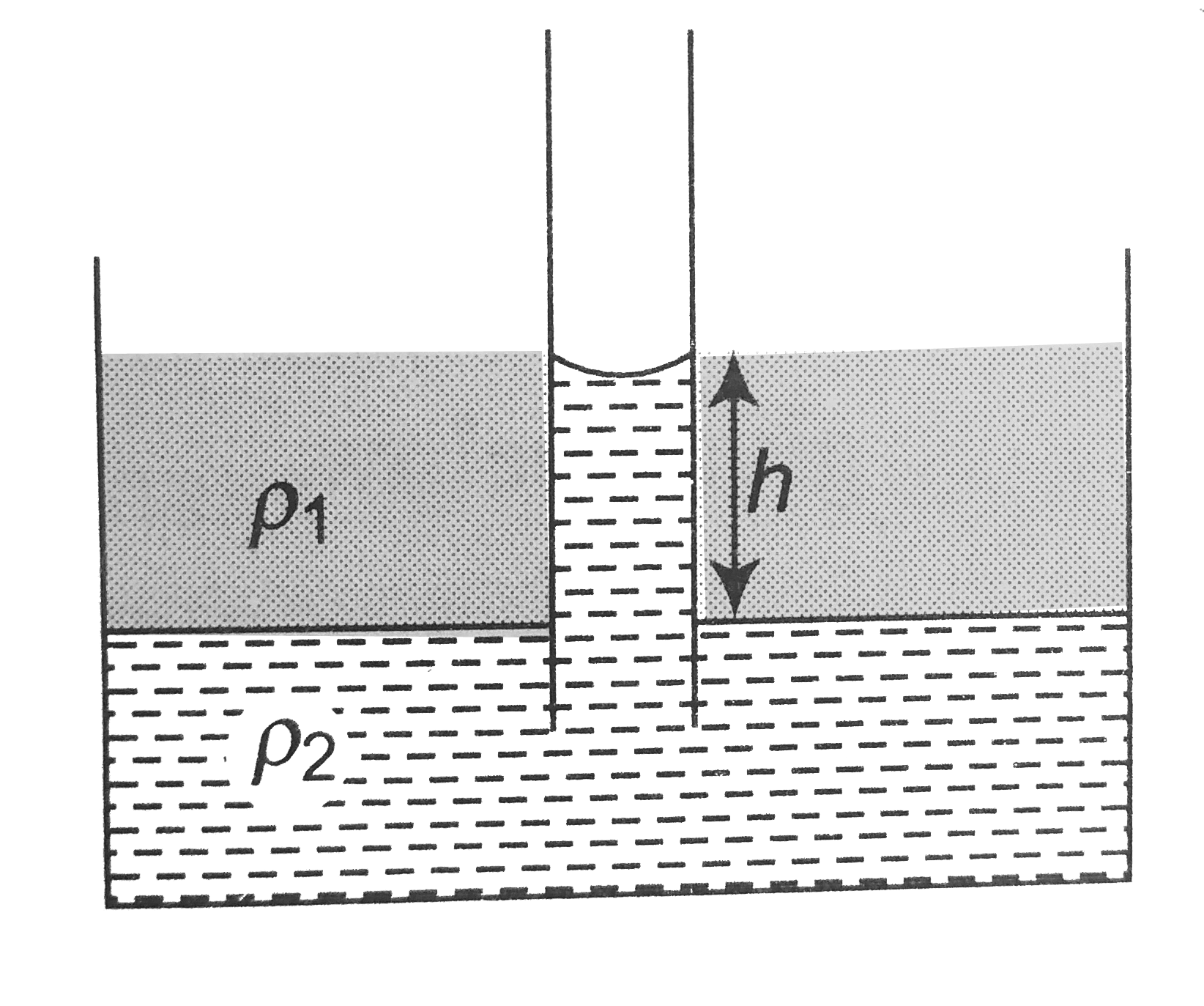
A container is partially filled with a liquid of density ρ2 A capillary tube of radius r is vertically inserted in this liquid. Now another liquid of density ρ1(ρ1<ρ2) is slowly poured in the container to a height h as shown. There is only denser liquid in the capillary tube. The rise of denser liquid in the capillary tube is also h. Assuming zero contact angle, the surface tension of heavier liquid is
View Solution
