A small circular coil with a mass of m consists of N turns of fine wire and carries a current of I. The coil is located in a uniform magnetic field of B with the coil axis orginally parallel to the field direction.

a. What is the period of small angle oscilations of the coil axis around its equilibrium position, assuming no friction and no mechanical force?
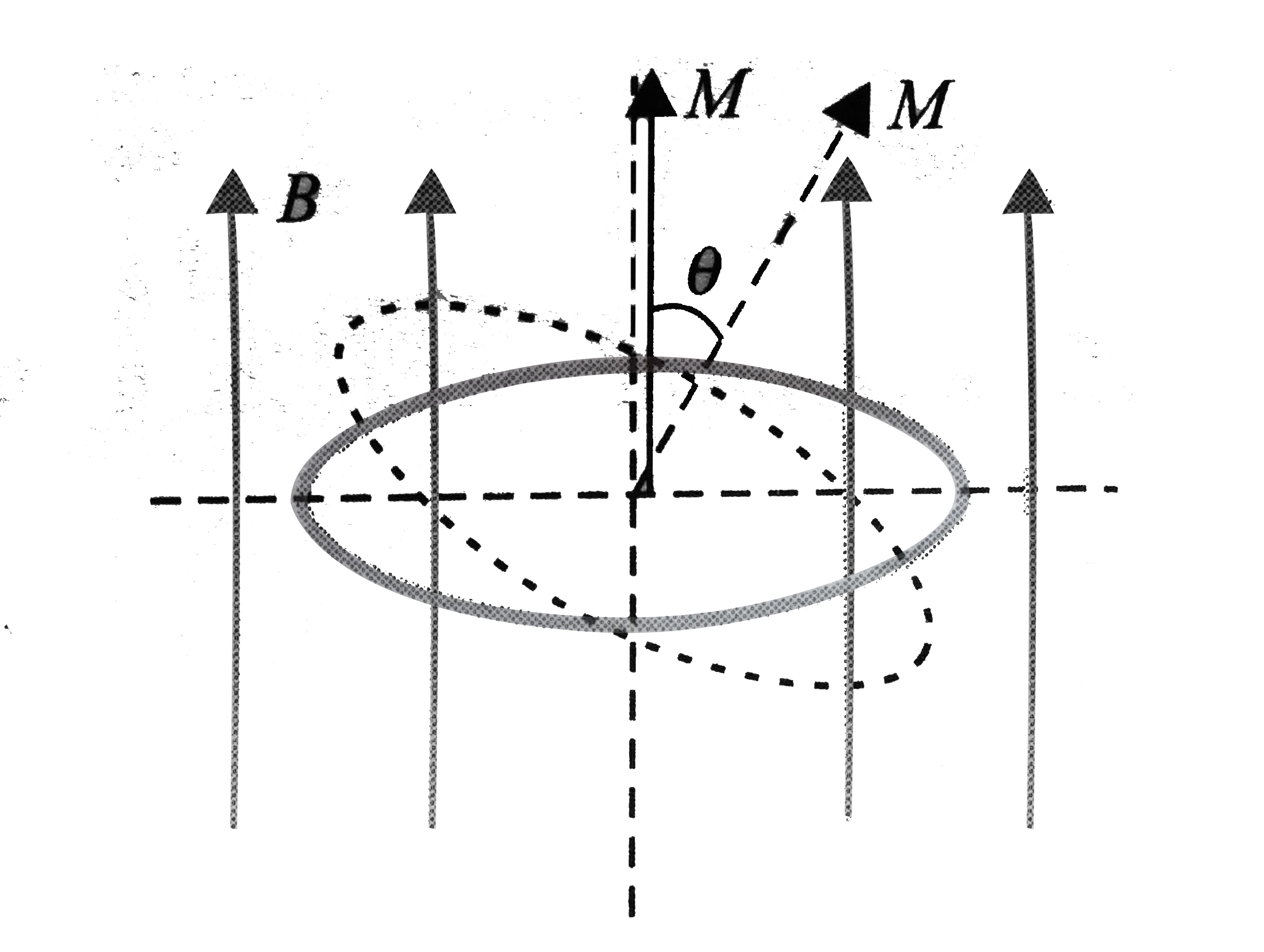
b. If the coil is rotated through an angle of $θ$ from its equilibrium position and then released, what will be its angular speed when it passes through equilibrium position?

Video Solution

Text Solution

Verified by Experts

The correct Answer is:(a) $2 π \sqrt{\frac{m}{2 N I π B}}$ ; (b) $\sqrt{\frac{4 N I π B (1 - cos θ)}{m}}$

(a) Magnetic moment of coil:
$M = N I A = N I π R^{2}$
Let the coil is displaced by small angle $θ$ , the torque on the
coil: $τ = M B sin θ$
$\Rightarrow - I α = M B θ$ (for small angle $sin θ \approx θ$ )
$\Rightarrow α = - (\frac{2 N I π B}{m}) θ$
Comparing with $α = - ω^{2} θ$ we get $ω = \sqrt{\frac{2 N I π B}{m}}$
Time period:
$T = \frac{2 π}{ω} = (2 π) \sqrt{\frac{m}{2 N I π B}}$
(b) Apply conservation of energy: ${(K E + P E)}_{i} = {(K E + P E)}_{f}$
$\Rightarrow θ 0 - M B {cos 10}^{\circ} = \frac{1}{2} I ω^{2} - M B {cos 0}^{\circ}$
$\Rightarrow \frac{1}{2} (\frac{1}{2} m R^{2}) ω^{2} = M B (1 - cos θ)$
$\Rightarrow \frac{1}{4} m R^{2} ω^{2} = N I π R^{2} B (1 - cos θ)$
$\Rightarrow ω = \sqrt{\frac{4 N I π B (1 - cos θ)}{m}}$ .

|

Updated on:21/07/2023

Class 12 PHYSICS MOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

CENGAGE PHYSICS ENGLISH-MAGNETIC FIELD AND MAGNETIC FORCES-Exercises Subjective

Home
Ask Doubt
Profile