A small disc of mass m slides down a smooth hill of height h without initial velocity and gets onto a plank of mass M lying on the horizontal plane at the base of the hill. (figure). Due to friction between the disc and the plank the disc slows down and, beginning with a certain moment, moves in one piece with the plank.
(1) Find the total work performed by the friction forces in this process.
(2) Can it be stated that the result of obtained does not depend on the choice of the reference frame?
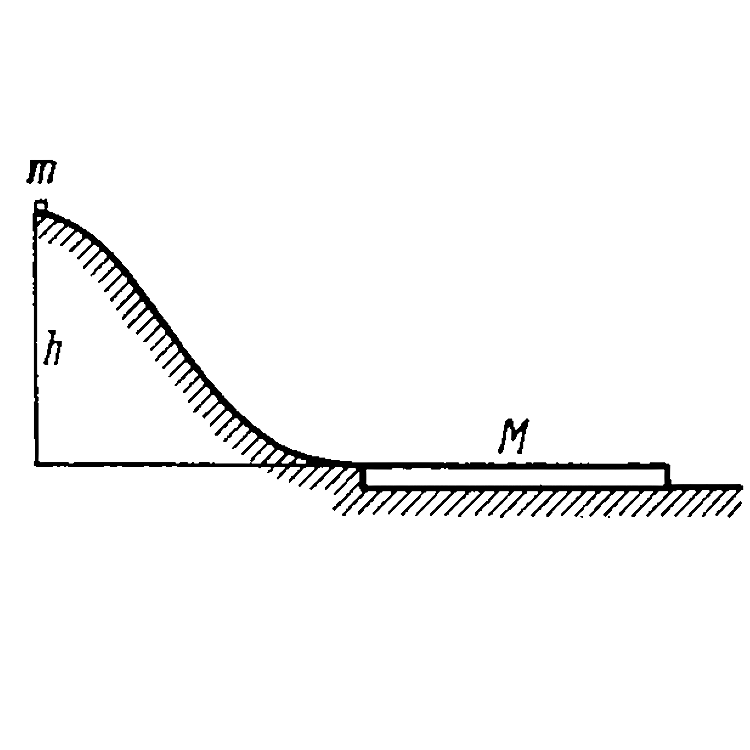
(a) whan the disc slides down and comes onto the plank, then
mgh=12mv2
∴v=√(2gh)
Let v1 be the common velocity of both, the disc and plank when they move together. From law of conservation of linear momentum,
mv=(M+m)v1
∴v1=mv(M+m)
Now, change in KE=(K)f−(K)i=(work done)friction
∴12(M+m)v21−12mv2=(work done)friction
or Wfr=12(M=m)[mvM+m]2−12mv2
=12mv2[mM+m−1]
as12mv2=mgh
Wfr=−mgh[MM+m]
(b) In part (a), we have calculated work done from the ground frame of reference. Now let us take plank as the reference frame.
 .
.
Accleration of plank a0=fm=μmgM
Free body diagram of disc with respect to plank is shown in figure.
Here, ma0=pseudo force.
Retardation of disc w.r.t. plank.
ar=f+ma0m=μmg+μm2gMm=μmg+μmgM
=(M+mM)μg
The disc will stop after travalling a distance Sr relative to plank, where
Sr=v2r2arMgh(M+m)μg,(0=v2r−2arSr)
∴ Work done by friction in this frame of reference
Wfr=−fSr−(μmg)[Mgh(M+m)μg]
=−Mmgh(M+m)
which is same as part (a).
Topper's Solved these Questions
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.1
10 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - Exercise 9.2
9 Videos
- WORK, ENERGY & POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY & POWEREXERCISE - TYPE2
1 Videos
- WAVE MOTIONBOOK - DC PANDEYCHAPTER - WAVE MOTIONEXERCISE - Integer Type Question
11 Videos
- WORK, ENERGY AND POWERBOOK - DC PANDEYCHAPTER - WORK, ENERGY AND POWEREXERCISE - MEDICAL ENTRACES GALLERY
33 Videos
Similar Questions
A block of mass m is pushed with a velocity v0 along the surface of a trolley car of mass M. If the horizontal ground is smooth and the coefficient of kinetic friction between the block of plank is μ. Find the minimum distance of relative sliding between the block and plank.

View SolutionIn the figure shown, a solid sphere of mass 'm' and radius r is released from a height 6r to slide down a smooth surface. A plank of same mass 'm' touches the horizontal portion of the surface at the ground. The co-efficient of friction between the plank and the sphere is μ and that between the plank and the ground is μ/4. Find the work done by the friction force between the plank and the ground till the sphere starts pure rolling on the plank. Neglect the height of the plank.
View Solution
Knowledge Check
A disc of mass m and radius R is placed over a plank of same mass m. There is sufficient friction between disc and plank to prevent slipping. A force F is applied at the centre of the disc.

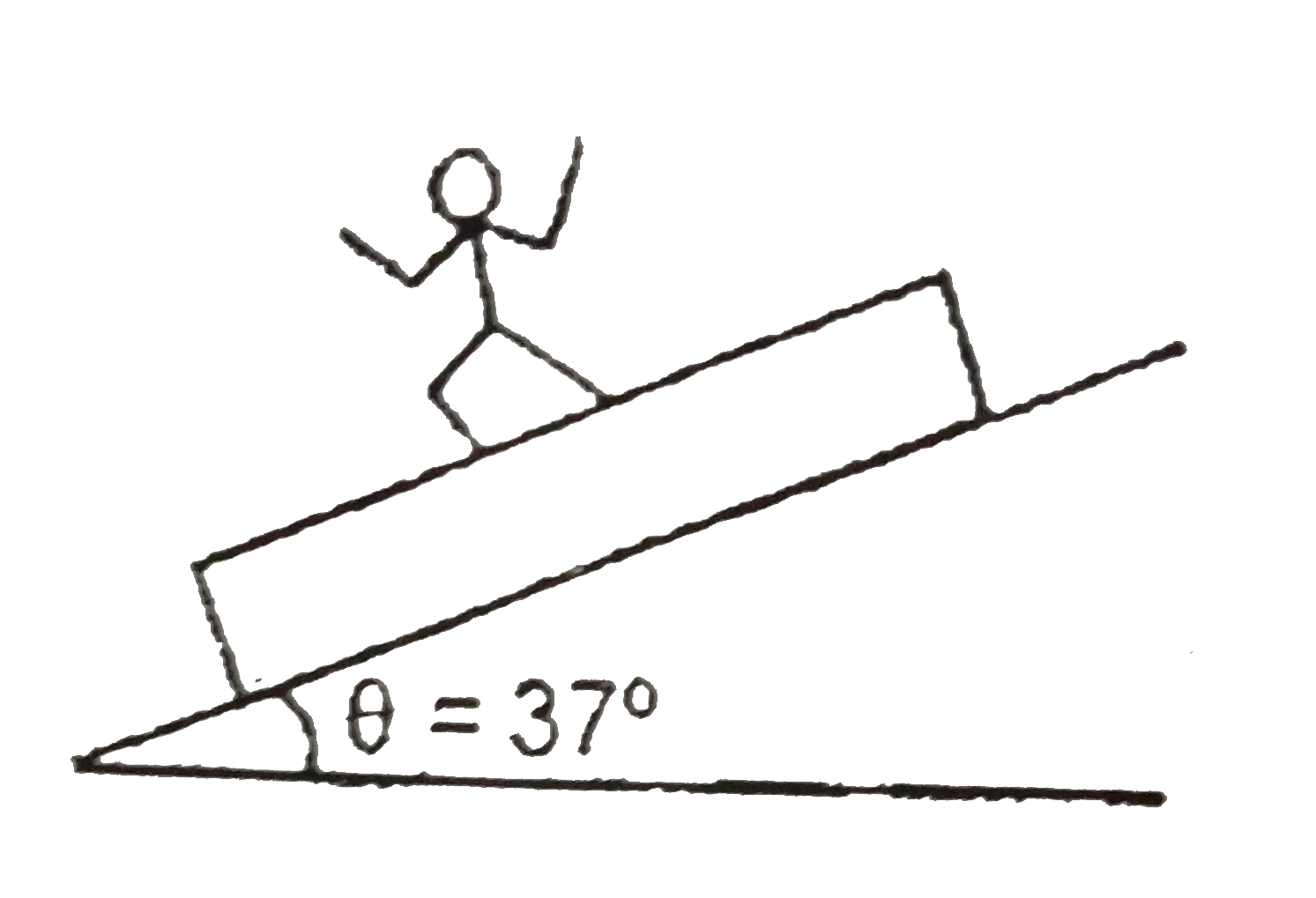
Force of friction between the disc and the plank isAF2BF4CF3D2F3A plank of mass 3m is placed on a rough inclined plane and a man of mass m, the coefficient of friction between the board and inclined plane is μ=0.5, the minimum acceleration of man so that plank does not slide is :
 A8m/s2B4m/s2C6m/s2D3m/s2
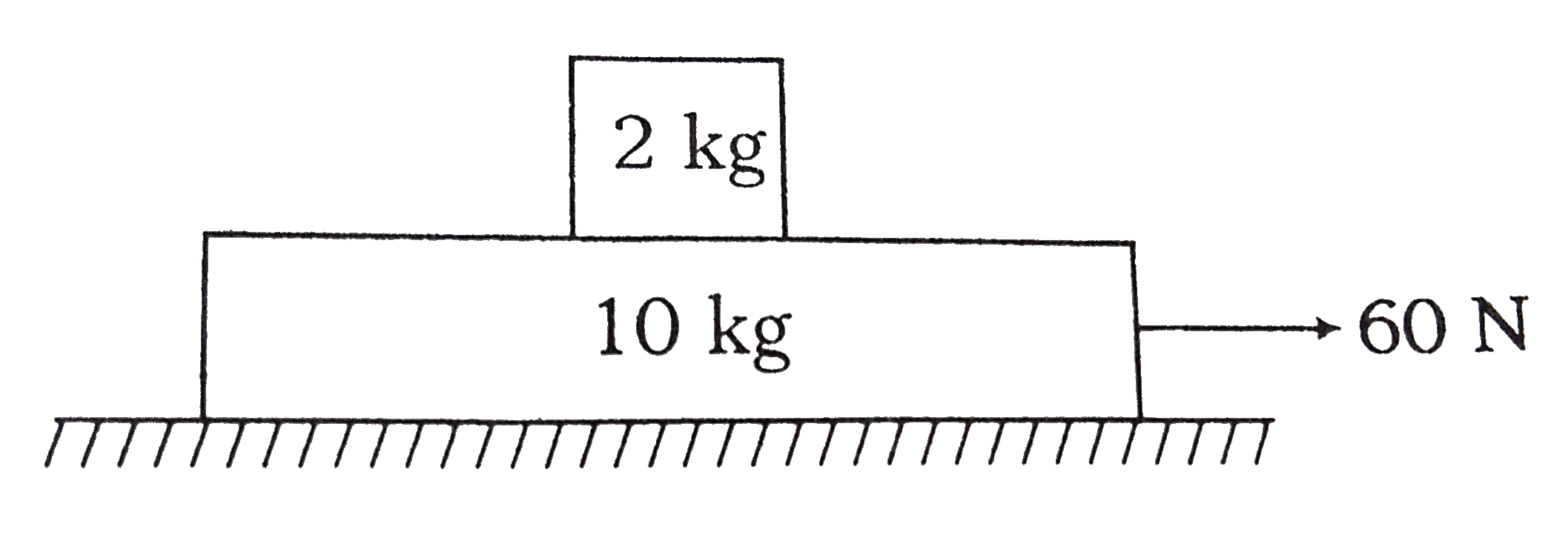
A8m/s2B4m/s2C6m/s2D3m/s2A plank of mass 10 kg and a block of mass 2 kg are placed on a horizontal plane as shown in the figure. There is no friction between plane and plank. The coefficient of friction between block and plank is 0.5 .A force of 60 N is applied on plank horizontally. In first 2 s the work done by friction on the block is
 A−100JB100 JCzeroD200 J
A−100JB100 JCzeroD200 J
A small disc A slides down with initial velocity equal to zero from the top of a smooth hill of height H having a horizontal portion. What must be the height of the horizontal portion h to ensure the maximum distance s covered by the disc? What is it equal to?

View SolutionA disc of mass m slides with the zero initial velocity down an inclined plane of inclination θ to the harizontal, having traveled the distance d along the horizontal plane, the disc stops. Find the work performed by the friction over the whole distance, assuming the friction coefficient μ for both the inclined and horizontal planes.
View SolutionA plank of mass M is placed on a rough horizontal force F is applied on it A man of mass m rens on the plank find the acceleration of the man so that the plank does not move on the surface . The coefficent of friction between the plank and the surface is μ Assume that the man does not slip on the plank

View SolutionA block of mass m is on the smooth horizontal surface of a plank of mass M The plank is on smooth horizontal surface Now, a constant horizontal force F acts on M. Now, for a person standing on the ground:
View SolutionA block of mass m is placed gently onto a long plank of mass M moving with a velocity v0 on a smooth horizontal floor. If friction is present between M and m :
View Solution
