A solid cylinder of mass $m$ is attached to a horizontal spring with force constant $k$ . The cylinder can roll without slipping along the horizontal plane. (See the accompanying figure.) Show that the center of mass of the cylinder executes simple harmonic motion with a period $T = 2 π \sqrt{\frac{3 m}{2 k}}$ , if displaced from mean position.

Video Solution

Text Solution

Verified by Experts

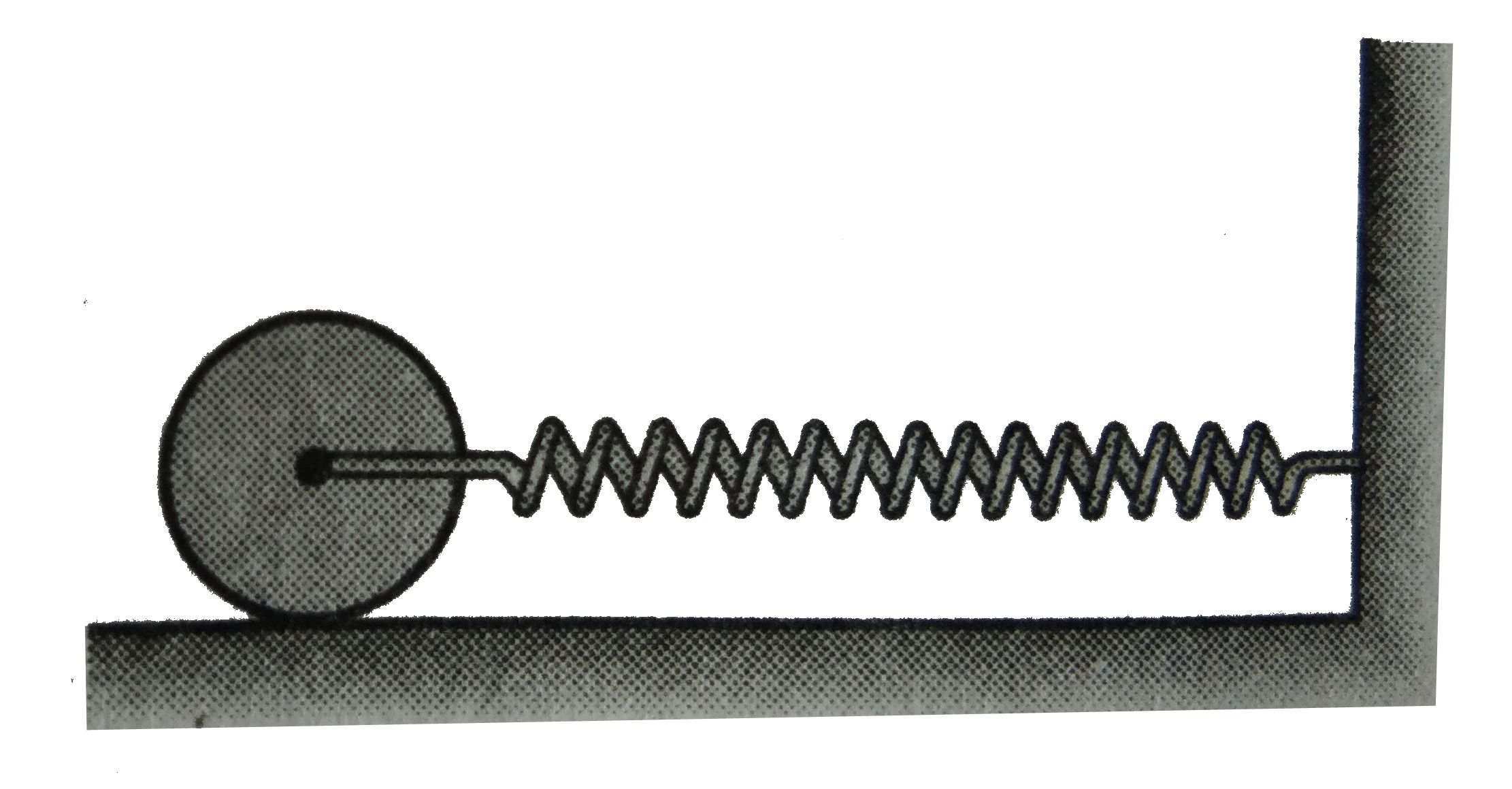
Let at any position, x be the extension in the spring and v be the velocity of centre of mass of the cylinder. Then,
P.E. of spring $U = \frac{1}{2} k x^{2}$
K.E. of translation, $K_{T} = \frac{1}{2} M v^{2}$
K.E. of rotation, $K_{R} = \frac{1}{2} I ω^{2} = \frac{1}{4} M v^{2}$
[ $∵ I = \frac{1}{2} M r^{2}$ and $v = ω r$ ]
Total mechanical energy of the sysem is
$E = U + K_{T} + K_{R} = \frac{1}{2} k x^{2} + \frac{1}{2} M v^{2} + \frac{1}{4} M v^{2}$
$= \frac{1}{2} k x^{2} + \frac{3}{4} M v^{2}$ ...(i)
As per question , $v = 0,$ if $x = 0.25 m$ , then
$E + \frac{1}{2} \times 3 \times (0.25) + 0 = \frac{3}{32} J$
At equilibrium position, $U = 0$ [as $x = 0$ ],
so from (i),
$\frac{3}{32} = 0 + \frac{3}{4} M v^{2}$ or $M v^{2} = \frac{1}{8} J$
At equilibrium position
(a) Translational K.E.
$= \frac{1}{2} M v^{2} = \frac{1}{2} (\frac{1}{8}) = \frac{1}{16} J$
(b) Rotational K.E.,
$= \frac{1}{4} M v^{2} = \frac{1}{4} (\frac{1}{8}) = \frac{1}{32} J$
(c) In SHM, the total energy is conserved,
so $\frac{d E}{d t} = 0$
From (i), we have
$0 = \frac{1}{2} k .2 x \frac{d x}{d t} + \frac{3}{4} M 2 v \frac{d v}{d t}$
or $\frac{3}{4} M \frac{d^{2} x}{{d t}^{2}} = - \frac{1}{2} k x$
$[∵ \frac{d x}{d t} = v$ and $\frac{d v}{d t} = \frac{d^{2} x}{{d t}^{2}}]$
or $\frac{d^{2} x}{{d t}^{2}} = \frac{- 2 k}{3 M} x$ ...(ii)
From (ii), we note that acceleration $(d^{2} x / {d t}^{2}) \propto x$ and is directed towards equilibrium position. Hence, the cylinder will execute SHM. Comparing (ii) with the equation
$\frac{d^{2} x}{{d t}^{2}} = - ω^{2} x,$ we have
$ω^{2} = \frac{2 k}{3 M}$
or $ω = \sqrt{\frac{2 k}{3 M}}$
Time period of SHM is,
$T = \frac{2 π}{ω} = 2 π \sqrt{\frac{3 M}{2 k}}$

Updated on:21/07/2023