A solid hemispherical bowl of radius R, made of glass, is placed on a flat horizontal surface with its curved surface on the ground. A small object is placed under the hemisphere at the center of the curved surface. If an observer sees the object directly above the plane surface, Where will the object appear to be ?
To solve the problem, we need to determine where the observer sees the object placed under the hemispherical bowl. We will use the concept of apparent depth in optics.
1. Understanding the Setup:
- We have a solid hemispherical bowl of radius R made of glass.
- The bowl is placed with its curved surface on the ground, and a small object is placed at the center of the curved surface (which is the bottom of the hemisphere).
2. Position of the Observer:
- The observer is looking from above the flat horizontal surface (the plane surface) and sees the object directly above this surface.
3. Refraction of Light:
- When light travels from one medium to another (in this case, from glass to air), it bends due to refraction. The apparent depth of an object when viewed through a medium can be calculated using the formula:
Apparent Depth=Real Depthμ
where μ is the refractive index of the medium (glass in this case).
4. Real Depth:
- The real depth of the object is equal to the radius R of the hemisphere since the object is at the center of the curved surface.
5. Calculating Apparent Depth:
- Substituting the values into the formula:
Apparent Depth=Rμg
where μg is the refractive index of glass.
6. Conclusion:
- Therefore, the object will appear to be at a depth of Rμg above the flat surface when viewed by the observer.
Final Answer:
The object will appear to be at a depth of Rμg above the plane surface.
---
- We have a solid hemispherical bowl of radius R made of glass.
- The bowl is placed with its curved surface on the ground, and a small object is placed at the center of the curved surface (which is the bottom of the hemisphere).
- The observer is looking from above the flat horizontal surface (the plane surface) and sees the object directly above this surface.
- When light travels from one medium to another (in this case, from glass to air), it bends due to refraction. The apparent depth of an object when viewed through a medium can be calculated using the formula:
Apparent Depth=Real Depthμ
where μ is the refractive index of the medium (glass in this case).
- The real depth of the object is equal to the radius R of the hemisphere since the object is at the center of the curved surface.
- Substituting the values into the formula:
Apparent Depth=Rμg
where μg is the refractive index of glass.
- Therefore, the object will appear to be at a depth of Rμg above the flat surface when viewed by the observer.
The object will appear to be at a depth of Rμg above the plane surface.
Topper's Solved these Questions
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.6
15 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.7
21 Videos
- GEOMETRICAL OPTICSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - GEOMETRICAL OPTICSEXERCISE - Exercise1.4
12 Videos
- ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - ELECTRON,PHONTS,PHOTOELECTRIC EFFECT & X-RAYSEXERCISE - dpp 3.3
15 Videos
- HEATING EFFECT OF CURRENTBOOK - CENGAGE PHYSICS ENGLISHCHAPTER - HEATING EFFECT OF CURRENTEXERCISE - Thermal Power in Resistance Connected in Circuit
27 Videos
Similar Questions
a hemispherical hollow body is placed in a uniform electric field E. the total flux linked with the curved surface is

View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical bowl is made of steel, 0.25 cm thick. The inner radius of the bowl is 5 cm. Find the outer curved surface area of the bowl.
View SolutionA hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
View SolutionA hemispherical body of radius R is placed in a uniform electric field E. What is the flux linked with the curved surface if, the field is (a) parallel to the base, (b) perpendicular to the base.
View Solutiona hemispherical hollow body is placed in a uniform electric field E. the total flux linked with the curved surface is

View SolutionIf two solid hemispheres of same base radius r are joined together along their bases, then the curved surface area of the this new solid is
View SolutionA hemispherical surface of radius R is kept in a uniform electric field E as shown in figure. The flux through the curved surface is
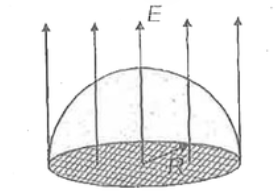
View SolutionA cylinder of same height and radius is placed on the top of a hemisphere. Find the curved surface area of the shape if the length of the shape by 7cm.
View Solution
