A solid metallic sphere of radius r is allowed to fall freely through air. If the frictional resistance due to air is proportional to the cross-sectional area and to the square of the velocity, then the terminal velocity of the sphere is proportional to which of the following ?
r2
r
r3/2
r1/2
The correct Answer is:D
To find the terminal velocity of a solid metallic sphere falling through air, we need to analyze the forces acting on the sphere and how they relate to the terminal velocity. Here's a step-by-step solution:
Step 1: Identify the forces acting on the sphere
When the sphere falls, two main forces act on it:
- The weight of the sphere (downward force)
- The frictional resistance due to air (upward force)
Step 2: Write the expression for the frictional resistance
The frictional resistance (viscous force) Fb due to air is given to be proportional to the cross-sectional area and the square of the velocity. Mathematically, this can be expressed as:
Fb=k⋅A⋅v2
where:
- k is a proportionality constant,
- A is the cross-sectional area of the sphere,
- v is the velocity of the sphere.
Step 3: Calculate the cross-sectional area
The cross-sectional area A of a sphere with radius r is given by:
A=πr2
Step 4: Substitute the area into the frictional force equation
Substituting the expression for the area into the frictional force equation gives:
Fb=k⋅(πr2)⋅v2
Step 5: Set up the equation for terminal velocity
At terminal velocity, the upward frictional force equals the downward weight of the sphere. The weight W of the sphere can be expressed as:
W=m⋅g
where m is the mass of the sphere and g is the acceleration due to gravity. The mass can be expressed in terms of density ρ and volume V:
m=ρ⋅V
The volume V of the sphere is given by:
V=43πr3
Thus, the weight becomes:
W=ρ⋅(43πr3)⋅g
Step 6: Equate the forces at terminal velocity
Setting the frictional force equal to the weight gives:
k⋅(πr2)⋅v2t=ρ⋅(43πr3)⋅g
where vt is the terminal velocity.
Step 7: Simplify the equation
We can cancel π from both sides:
k⋅r2⋅v2t=43ρr3g
Now, divide both sides by r2:
k⋅v2t=43ρrg
Step 8: Solve for terminal velocity
Rearranging the equation to solve for v2t:
v2t=43⋅ρgk⋅r
Taking the square root to find vt:
vt=√43⋅ρgk⋅r
Step 9: Determine the proportionality
From the final expression for terminal velocity, we can see that:
vt∝√r
This indicates that the terminal velocity of the sphere is proportional to the square root of its radius.
Conclusion
The terminal velocity of the sphere is proportional to r1/2.
When the sphere falls, two main forces act on it:
- The weight of the sphere (downward force)
- The frictional resistance due to air (upward force)
The frictional resistance (viscous force) Fb due to air is given to be proportional to the cross-sectional area and the square of the velocity. Mathematically, this can be expressed as:
Fb=k⋅A⋅v2
where:
- k is a proportionality constant,
- A is the cross-sectional area of the sphere,
- v is the velocity of the sphere.
The cross-sectional area A of a sphere with radius r is given by:
A=πr2
Substituting the expression for the area into the frictional force equation gives:
Fb=k⋅(πr2)⋅v2
At terminal velocity, the upward frictional force equals the downward weight of the sphere. The weight W of the sphere can be expressed as:
W=m⋅g
where m is the mass of the sphere and g is the acceleration due to gravity. The mass can be expressed in terms of density ρ and volume V:
m=ρ⋅V
The volume V of the sphere is given by:
V=43πr3
Thus, the weight becomes:
W=ρ⋅(43πr3)⋅g
Setting the frictional force equal to the weight gives:
k⋅(πr2)⋅v2t=ρ⋅(43πr3)⋅g
where vt is the terminal velocity.
We can cancel π from both sides:
k⋅r2⋅v2t=43ρr3g
Now, divide both sides by r2:
k⋅v2t=43ρrg
Rearranging the equation to solve for v2t:
v2t=43⋅ρgk⋅r
Taking the square root to find vt:
vt=√43⋅ρgk⋅r
From the final expression for terminal velocity, we can see that:
vt∝√r
This indicates that the terminal velocity of the sphere is proportional to the square root of its radius.
The terminal velocity of the sphere is proportional to r1/2.
Topper's Solved these Questions
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - JEE Advanced
57 Videos
- PROPERTIES OF MATTERBOOK - DC PANDEY ENGLISHCHAPTER - PROPERTIES OF MATTEREXERCISE - More than one option is correct
21 Videos
- PROJECTILE MOTIONBOOK - DC PANDEY ENGLISHCHAPTER - PROJECTILE MOTIONEXERCISE - Level - 2 Subjective
10 Videos
- RAY OPTICSBOOK - DC PANDEY ENGLISHCHAPTER - RAY OPTICSEXERCISE - Integer type q.
14 Videos
Similar Questions
The viscous force acting on a solid ball of surface area. A moving with terminal velocity v is proportional to
View SolutionA small steel ball of radius r is allowed to fall under gravity through a column of a viscous liquid of coefficient of viscosity η. After some time the velocity of the ball attains a constant value known as terminal velocity υT. The terminal velocity depends on (i) the mass of the ball m (ii) η, (iii) r and (iv) acceleration due to gravity g . Which of the following relations is dimensionally correct?
View Solution
Knowledge Check
Metallic sphere of radius R is charged to potential V. Then charge q is proportional to
AVBRCboth V and RDnone of theseA spherical metal ball of mass m and radius (r) is falling through a viscous medium. The value of its terminal velocity is proportional to
A1/r onlyBm/rC(m/r)1/2Dm only
Two metal spheres of radius r have centers at a distance d apart in air. Find the capacitance of the system.
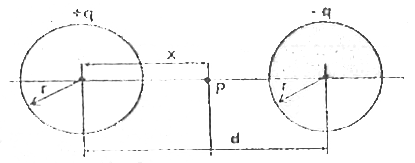
View SolutionA small sphere falls from rest in a viscous liquid. Due to friction, heat is produced. Find the relation between the rate of production of heat and the radius of the sphere at terminal velocity.
View SolutionA stone is allowed to fall freely from a certain Height. Neglecting air resistance, which graph represents the variation of velocity v with time t ?
View SolutionA small metal sphere of radius a is falling with a velocity υ through a vertical column of a viscous liquid. If the coefficient of viscosity of the liquid is η, then the sphere encounters an opposing force of
View SolutionThe terminal velocity of a sphere of radius R, falling in a viscous fluid, is proportional to
View SolutionA small sphere falls from rest in a viscous liquid. Due to friction, heat is produced. Find the relation between the rate of produced heat and the radius of the sphere at terminal velocity.
View Solution
