A sounding body emitting a frequency of 150HZ is dropped from a height. During its fall under gravity it crosses a balloon moving upwards with a constant velocity of 2m/s one second after it started to fall . The difference in the frequency observer by the man in balloon just before and just afer crossing the body will be (velocity of sound =300m/s, g=10m/s2)
12
6
8
4
The correct Answer is:A
To solve the problem step by step, we will calculate the apparent frequency observed by the man in the balloon just before and just after crossing the sounding body.
Step 1: Determine the velocity of the sounding body just before crossing the balloon
The sounding body is dropped from a height and falls under gravity. The velocity of the sounding body just before crossing the balloon after 1 second can be calculated using the formula:
v=g⋅t
Where:
- g=10m/s2 (acceleration due to gravity)
- t=1s
Calculating this gives:
v=10⋅1=10m/s
Step 2: Calculate the apparent frequency just before crossing the balloon
The apparent frequency f′ observed by the observer in the balloon just before crossing the body can be calculated using the Doppler effect formula:
f′=f⋅v+vov−vs
Where:
- f=150Hz (frequency of the source)
- v=300m/s (velocity of sound)
- vo=2m/s (velocity of the observer moving upwards)
- vs=10m/s (velocity of the source moving downwards)
Substituting the values:
f′=150⋅300+2300−10
Calculating this gives:
f′=150⋅302290
Step 3: Calculate the apparent frequency just after crossing the balloon
After crossing, the source is moving downwards at 10 m/s and the observer is moving upwards at 2 m/s. The apparent frequency f′′ can be calculated using:
f′′=f⋅v−vov+vs
Substituting the values:
f′′=150⋅300−2300+10
Calculating this gives:
f′′=150⋅298310
Step 4: Calculate the difference in frequencies
Now, we find the difference in the frequencies observed just before and just after crossing:
Δf=f′−f′′
Substituting the expressions we derived:
Δf=150⋅302290−150⋅298310
Factoring out 150 gives:
Δf=150(302290−298310)
Step 5: Simplify and calculate the final difference
To combine the fractions, we need a common denominator:
Δf=150(302⋅310−298⋅290290⋅310)
Calculating the numerator:
302⋅310=93620
298⋅290=86420
93620−86420=7200
Thus:
Δf=150⋅7200290⋅310
Calculating the denominator:
290⋅310=89900
Finally:
Δf=150⋅720089900
Calculating this gives:
Δf≈12Hz
Final Answer
The difference in frequency observed by the man in the balloon just before and just after crossing the sounding body is approximately 12 Hz.
The sounding body is dropped from a height and falls under gravity. The velocity of the sounding body just before crossing the balloon after 1 second can be calculated using the formula:
- g=10m/s2 (acceleration due to gravity)
- t=1s
The apparent frequency f′ observed by the observer in the balloon just before crossing the body can be calculated using the Doppler effect formula:
- f=150Hz (frequency of the source)
- v=300m/s (velocity of sound)
- vo=2m/s (velocity of the observer moving upwards)
- vs=10m/s (velocity of the source moving downwards)
After crossing, the source is moving downwards at 10 m/s and the observer is moving upwards at 2 m/s. The apparent frequency f′′ can be calculated using:
Now, we find the difference in the frequencies observed just before and just after crossing:
To combine the fractions, we need a common denominator:
298⋅290=86420
93620−86420=7200
The difference in frequency observed by the man in the balloon just before and just after crossing the sounding body is approximately 12 Hz.
Topper's Solved these Questions
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - More Than One Option is Correct23videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - Comprehion Type Questions20videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - WAVE MOTIONEXERCISE - JEE MAINS50videos
WAVE MOTION
- BOOK - DC PANDEY ENGLISHCHAPTER - VECTORSEXERCISE - Medical enrances gallery9videos
VECTORS
- BOOK - DC PANDEY ENGLISHCHAPTER - WORK, ENERGY & POWEREXERCISE - Level 2 Comprehension Based2videos
WORK, ENERGY & POWER
Similar Questions
A balloon is going upwards with a constant velocity 15m/s. When the ballooon is at 50 m height, a stone is dropped outside from the balloon. How long will stone take to reach at the ground? (take g=10m/s2)
View SolutionAn object is projected vertically up from the earth’s surface with velocity √Rg where R is the radius of the earth and ‘g’ is the acceleration due to earth on the surface of earth. The maximum height reached by the object is nR. Find value of n.
View SolutionA body is dropped from a certain height h (h is very large) and second bodyis thrown downward with velocity of 5 m/s simultaneouly. What will be difference in heights of the two bodies after 3 s?
View SolutionA source is moving with a constant speed of 10 m/s on a circular track of 200 m. It emits a sound of frequency 200 Hz. A listener stands at the centre of the circular track. The frequency received by the listener is (velocity of sound = 340 m/s)
View SolutionA man in a balloon throws a stone downwards with a speed of 5m/s with respect to balloon. The balloon is moving upwards with a constant acceleration of 5m/s2. Then velocity of the stone relative to the man after 2 seconds is.
 View Solution
View SolutionA man holding a sound source at the top of a tower emitting frequency of sound as v=1000Hz. Suddenly it slips from his hand and falls down with 'g' acceleration. The frequency of sound heard by the man at t=4sec. (Velocity of sound =320m/s, g=10m/s2)
View SolutionA body falling on the ground from a height of 10m, rebounds to a height 2.5 m Calculate
(i) The percentage loss in KE
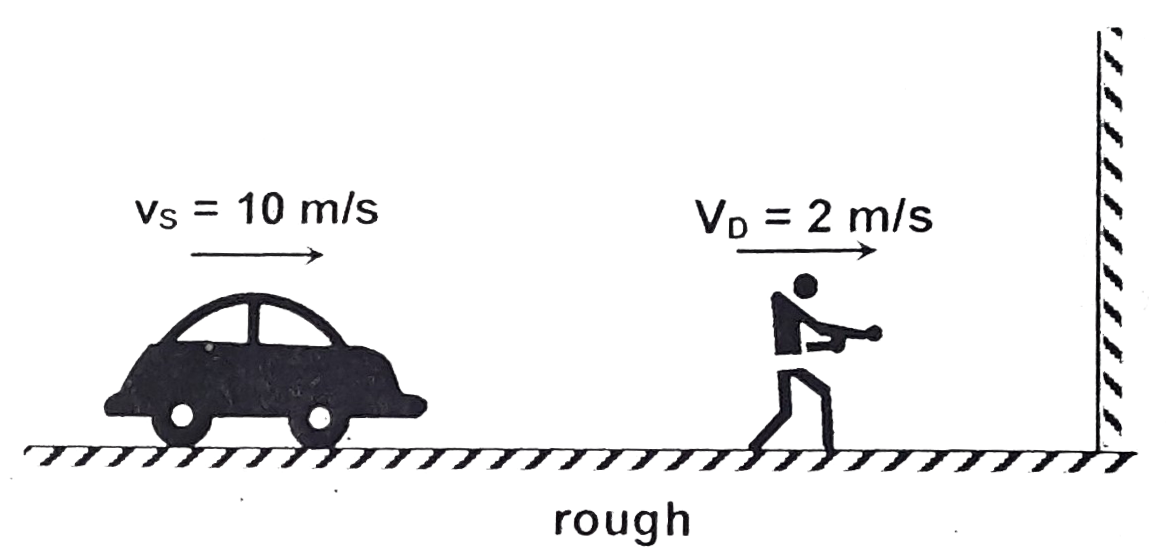
(ii) Ratio of the velocities of the body just before and just after the collision.View SolutionA car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
 View Solution
View SolutionA body is dropped from a balloon meving up wigh a velocity of 4ms−2 when the balloon is at a height of 12.5 m from the ground.
The distance of separation between of separation between the body and the balloon after 5 is.View Solution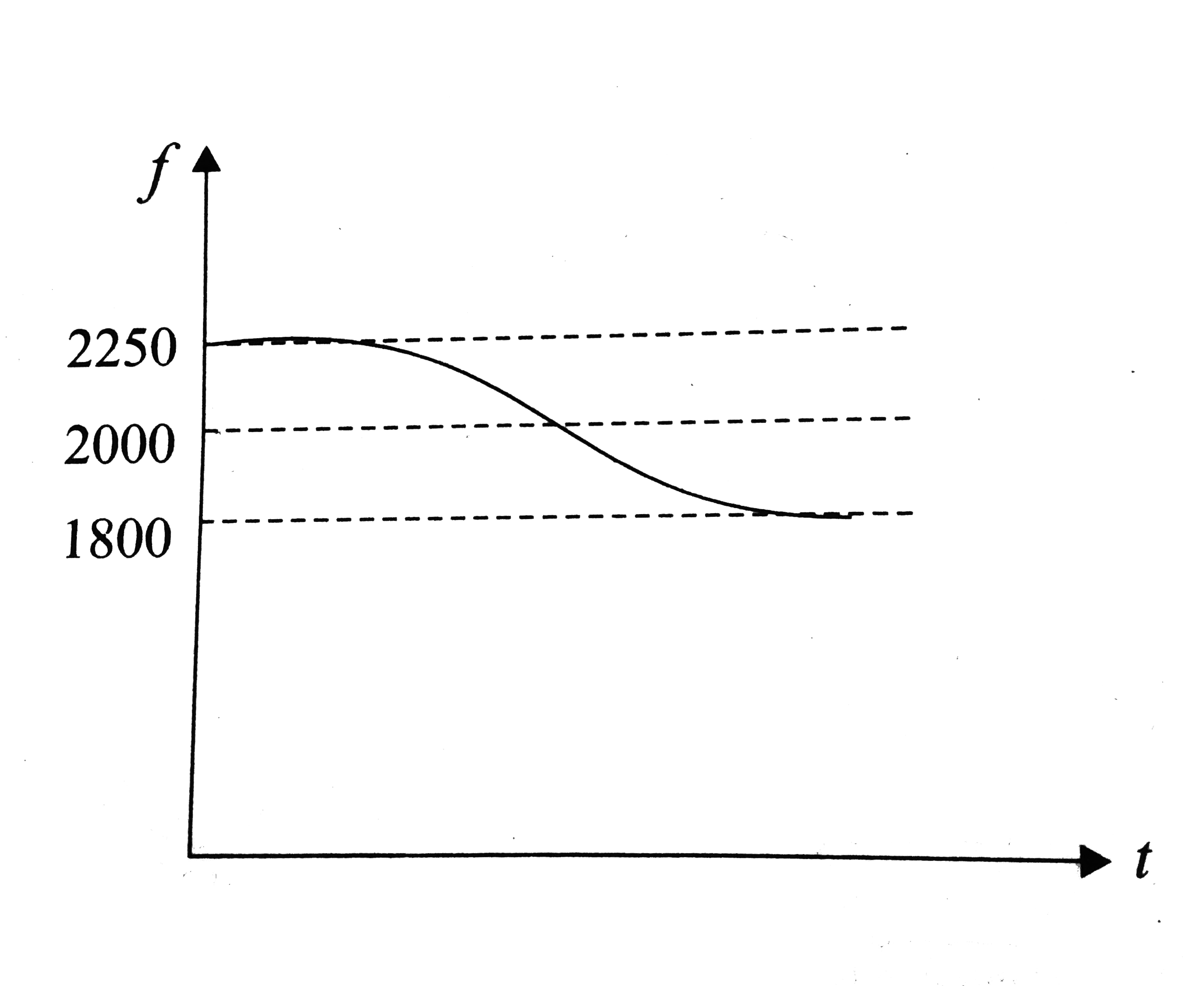
A statinary observer receives a sound of frequency 2000 Hz. The variation of apparent frequency and time is shown. Find the speed of source, if velocity of sound is 300msView Solution

