A source is moving with constant speed υs=20m/s towards a stationary observer due east of the source. Wind is blowing at the speed of 20m/s at 60∘ north of east. The source has frequency 500HZ. Speed of sound =300m/s. The frequency resgistered by the observer is approximately
541HZ
552HZ
534HZ
512HZ
The correct Answer is:C
To solve the problem, we need to calculate the frequency registered by the observer when a sound source is moving towards them while wind is blowing in a specific direction. Here’s a step-by-step solution:
Step 1: Identify the given values
- Speed of the source, vs=20m/s
- Frequency of the source, fs=500Hz
- Speed of sound, v=300m/s
- Speed of wind, vw=20m/s
- Wind direction: 60∘ north of east
Step 2: Resolve the wind speed into components
The wind speed needs to be resolved into its eastward and northward components. Since the wind is blowing at 60∘ north of east:
- Eastward component of wind, vw,east=vwcos(60∘)=20cos(60∘)=20×12=10m/s
- Northward component of wind, vw,north=vwsin(60∘)=20sin(60∘)=20×√32≈17.32m/s
Step 3: Calculate the effective speed of sound towards the observer
The effective speed of sound towards the observer combines the speed of sound and the eastward component of the wind:
v′=v+vw,east=300m/s+10m/s=310m/s
Step 4: Apply the Doppler effect formula
The frequency observed by the observer can be calculated using the Doppler effect formula:
f′=fs⋅v′−0v′−vs
Where:
- f′ is the observed frequency,
- fs is the source frequency,
- v′ is the effective speed of sound towards the observer,
- vs is the speed of the source.
Substituting the values:
f′=500⋅310−0310−20=500⋅310290
Step 5: Calculate the observed frequency
Now, calculate the observed frequency:
f′=500⋅310290≈500⋅1.068965517=534.48Hz
Rounding off, we can say the frequency registered by the observer is approximately:
f′≈534Hz
Conclusion
The frequency registered by the observer is approximately 534 Hz.
- Speed of the source, vs=20m/s
- Frequency of the source, fs=500Hz
- Speed of sound, v=300m/s
- Speed of wind, vw=20m/s
- Wind direction: 60∘ north of east
The wind speed needs to be resolved into its eastward and northward components. Since the wind is blowing at 60∘ north of east:
- Eastward component of wind, vw,east=vwcos(60∘)=20cos(60∘)=20×12=10m/s
- Northward component of wind, vw,north=vwsin(60∘)=20sin(60∘)=20×√32≈17.32m/s
The effective speed of sound towards the observer combines the speed of sound and the eastward component of the wind:
v′=v+vw,east=300m/s+10m/s=310m/s
The frequency observed by the observer can be calculated using the Doppler effect formula:
f′=fs⋅v′−0v′−vs
Where:
- f′ is the observed frequency,
- fs is the source frequency,
- v′ is the effective speed of sound towards the observer,
- vs is the speed of the source.
f′=500⋅310−0310−20=500⋅310290
Now, calculate the observed frequency:
f′=500⋅310290≈500⋅1.068965517=534.48Hz
Rounding off, we can say the frequency registered by the observer is approximately:
f′≈534Hz
The frequency registered by the observer is approximately 534 Hz.
Topper's Solved these Questions
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Single correct option
1 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Level 2 More Than One Correct
5 Videos
- SOUND WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SOUND WAVESEXERCISE - Subjective Questions
1 Videos
- SOLVD PAPERS 2017 NEET, AIIMS & JIPMERBOOK - DC PANDEY ENGLISHCHAPTER - SOLVD PAPERS 2017 NEET, AIIMS & JIPMEREXERCISE - Solved paper 2018(JIPMER)
38 Videos
- SUPERPOSITION OF WAVESBOOK - DC PANDEY ENGLISHCHAPTER - SUPERPOSITION OF WAVESEXERCISE - Level 2 Subjective
8 Videos
Similar Questions
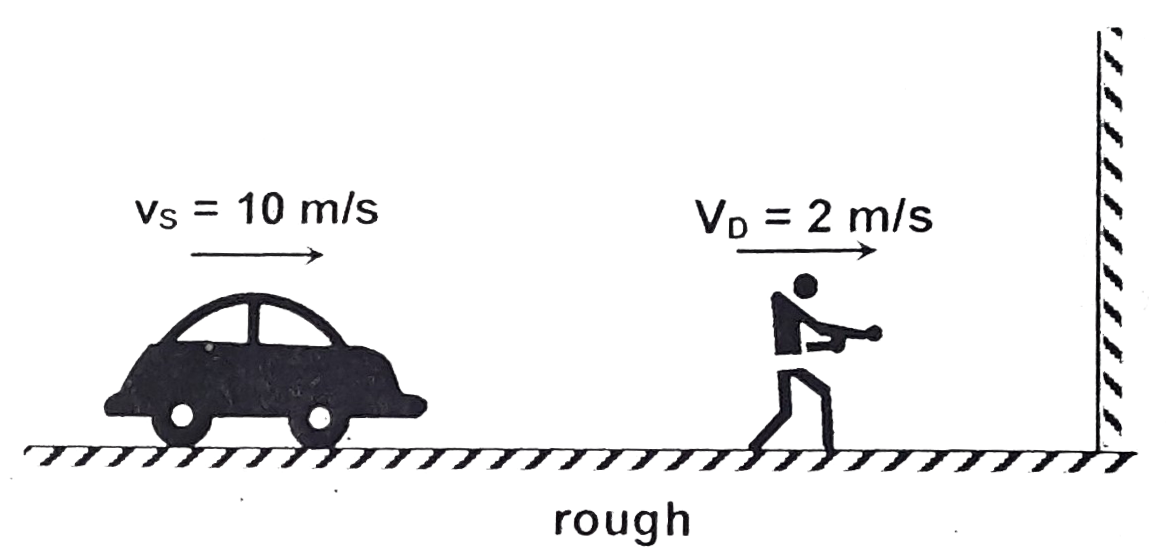
A car is moving with a constant velocity 10m/s towards the stationary wall. The car blows a horn of frequency 320Hz. The velocity of sound in air is 330m/s. The beats frequency received by the observer is
View SolutionAn observer moves on the same line on which two sources of sound of frequency 660Hz are present. The observer observes beat frequency of 10Hz. If speed of sound is 300m/s then speed of the observer is:
View Solution
Knowledge Check
A sound source is moving towards a stationary observer with 1/10 of the speed of sound. The ratio of apparent to real frequency is
A109B(109)2C(1110)2D1110
A source of sound of frequency 500 Hz is moving towards an observer with velocity 30 m/s . The speed of sound is 330 m/s . the frequency heard by the observer will be
View SolutionAt t=0 , observer and source are at same place. Now the source is projected with velocity 60√2m/s at 45∘ . Natural frequency of source is 1000 Hz.find the frequency heard by the observer at t=2s. Take speed of sound = 340 m/s
View SolutionA source of sound is travelling towards a stationary observer. The frequency of sound heard by the observer is 25% more that the actual frequency. If the speed of sound is v, that of the source is
View SolutionA source of sound is travelling with a velocity of 30ms towards a stationary observer. If actual frequency of source is 1000 Hz and the wind is blowing with velocity 20ms in a direction at 60∘C with the direction of motion of source, then the apparent frequency heard by observer is (speed of sound is 340ms)
View Solutiona bus is moving towards and stationary observer with speed 20 m/s blows a horn of frequency 200 Hz. the frequency of sound heard by the observer is approximately ( take speed of sound 320 m/s)
View SolutionA sound source moves with a speed of 80m/s relative to still air toward a stationary listener. The frequency of sound is 200HZ and speed of sound in air is 340m/s. (a) Find the wavelength of the sound between the source and the listener. (b) Find the frequency heard by the listener.
View SolutionA source of sound S is moving with a velocity of 50m/s towards a stationary observer. The observer measures the frequency of the source as 1000 Hz. What will be the apparent frequency of the source as 1000 Hz. What will be the apparent frequency of the source when it is moving away from the observer after crossing him? The velocity of the sound in the medium is 350m/s
View Solution
