A spaceship while flyting in space, splits up into three equal parts, due to an explosion. One fragment keeps moving in the same direction, the other two fly of at 60∘ to the original direction , on either side. If the energy released due to the explosion is twice the kinetic energy of the spaceship, find the kinetic energy of each of the fragment.
Let the mass of the spaceship be 3m and initial speed be u.
Hence, mass of each fragment =m . Let their velocity be v1,v2andv3 after the explosion [Fig.1.46].

Applying the conservation of momentum law along the direction perpendicular to the original direction of motion,
0=mv2sin60∘=mv3sin60∘
or,mv2sin60∘=mv3sin60∘
or,v2=v3=v (say)
Applying the law of conservation of linear momentum along the original direction of motion ,
3μ=mv1+2mvcos60∘
or,3u=v1+v
Kinetic energy of the spaceship before explosion
E=12×3μ2
Energy released during the explosion,
Er=12m(v21+2v2)−12⋅3μ2
According to the problem,
Er=2Eor,12m(v21+2v^2)−12⋅3μ2=2×12⋅3μ2
or,v21+2v2−3u2=6u2
or,v21+2v2=9u2......(2)
Solving (1) and (2) ,v1=uandv=2u
Hence, kinetic energy of the first fragment after explosion
=12mv21=12μ2=12⋅23⋅E=13E
Kinetic energy of each of the other two fragement
=12mv2=12m(2u)2=2μ2=2⋅23E=43E
Topper's Solved these Questions
- WORK AND ENERGYBOOK - CHHAYA PUBLICATIONCHAPTER - WORK AND ENERGYEXERCISE - SECTION RELATED QUESTIONS
24 Videos
- WORK AND ENERGYBOOK - CHHAYA PUBLICATIONCHAPTER - WORK AND ENERGYEXERCISE - HIGHER ORDER THINKING SKILL (HOTS) QUESTIONS
38 Videos
- WBCHSE QUESTION PAPER -2019BOOK - CHHAYA PUBLICATIONCHAPTER - WBCHSE QUESTION PAPER -2019EXERCISE - SECTION - II ( GROUP -D)
10 Videos
Similar Questions
A stationary shell explodes into two fragments, having masses in the ratio of 1:2. The heavier fragment attains a Kinetic energy of 100J. The Kinetic energy released in the explosion is:
View SolutionA shell of mass 2kg moving at a rate of 4m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities, If the explosion imparts 48J of translational kinetic energy to the fragments, find the velocity and direction of each fragment.
View Solution
Knowledge Check
A body kept at rest explodes in four identical fragments and it is found that three of them move along mutually perpendicular directions with same kinetic energy K . Total energy released in the explosion is
A3kB4KC6KD8KAn object of mass 3 kg at rest in space suddenly explodes into three parts of the same mass. The momenta of the two parts are 4ˆi and 2ˆj respectively. Then the energy released in the explosion is
A60 JB40 JC20 JD10 JA particle of mass 4m which is at rest explodes into four equal fragments. All 4 fragments are scattered in the same horizontal plane. Three fragments are found to move with velocity V each as shown in the figure. The total energy released in the process of explosion is
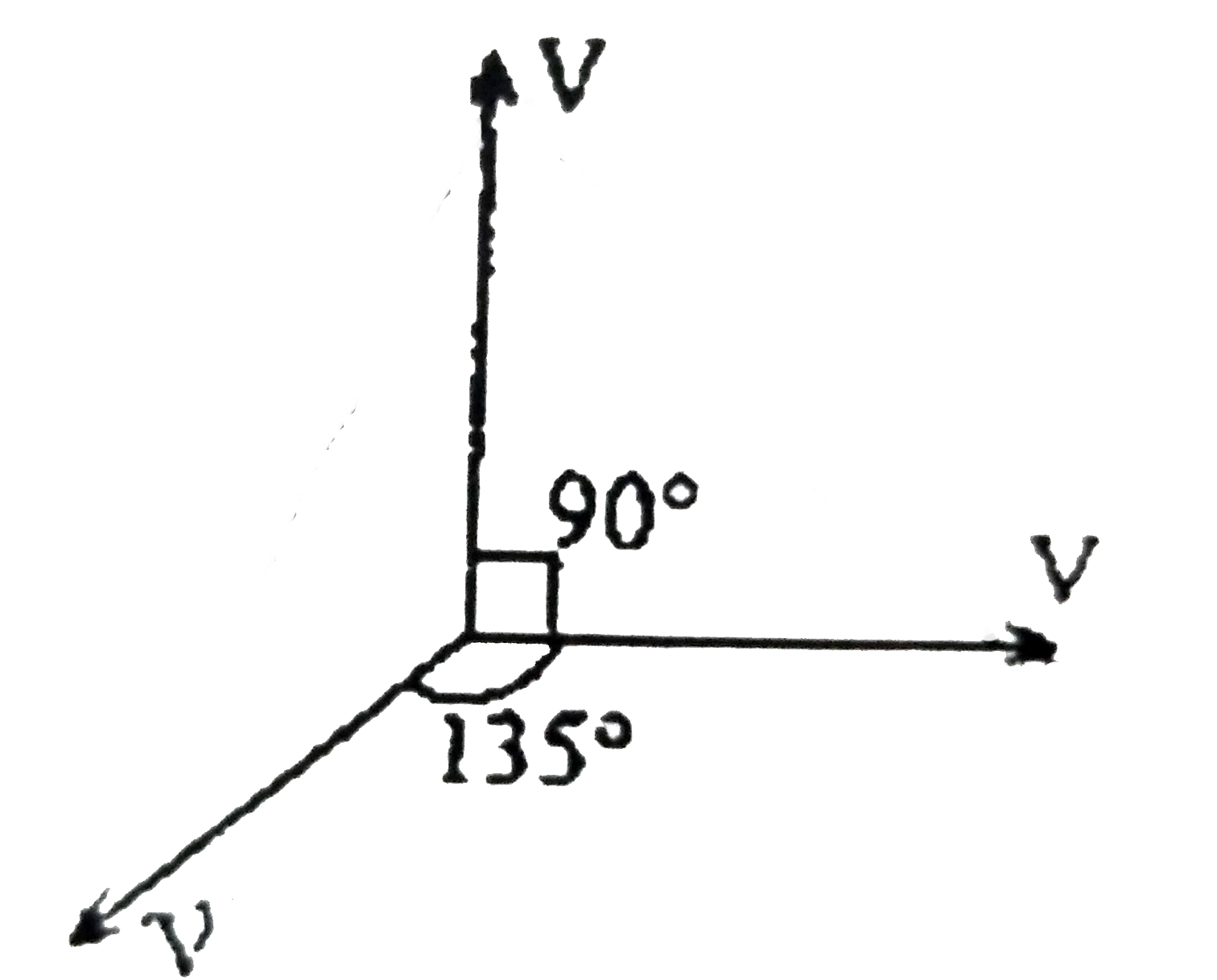 AmV2(3−√2)Bmv2(3−√2)/2C2mV2DmV2(1+√2)/2
AmV2(3−√2)Bmv2(3−√2)/2C2mV2DmV2(1+√2)/2
A shell of mass 2kg moving at a rate of 4m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities, If the explosion imparts 48J of translational kinetic energy to the fragments, find the velocity and direction of each fragment.
View SolutionAn object of mass 3 kg at rest in space suddenly explodes into three parts of the same mass. The momenta of the two parts are 4ˆi and 2ˆj respectively. Then the energy released in the explosion is
View SolutionA projectile at its highest point, breaks into two equal parts due to an internal explosion. One part moves vertically up at 30 m/s with respect to ground. Then the other part will move with speed if (Speed just before explosion is 20 m/s) :
View SolutionA shell of mass 2 kg and moving at a rate of 4m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities. If the explosion imparts 48 J of translational kinetic energy to the fragments divided equall, the velocity (magnitude and direction both) of each fragment:
View SolutionA shell of mass 2 kg and moving at a rate of 4 m/s suddenly explodes into two equal fragments. The fragments go in directions inclined with the original line of motion with equal velocities. If the explosion imparts 48 J of translational kinetic energy to the fragments divided equally, the velocity (magnitude and direction both) of each fragment :-
View Solution
