A sphere is rotating about a diameter
The particles on the surface of the sphere do not have any linear acceleration
The particles on the diameter mentioned above do not have any linear acceleration
Different particles on the surface have different angular speeds
All the particles on the surface have same linear speed
The correct Answer is:B
Step by step video, text & image solution for A sphere is rotating about a diameter by Physics experts to help you in doubts & scoring excellent marks in Class 11 exams.
Updated on:21/07/2023Topper's Solved these Questions
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on torque, couple)
11 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on moment of inertia)
33 Videos
- ROTATIONAL MOTIONBOOK - ERRORLESS CHAPTER - ROTATIONAL MOTIONEXERCISE - Practice Problems (Problems based on centre of mass)
12 Videos
- NEWTONS LAWS OF MOTIONBOOK - ERRORLESS CHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Self Evaluation Test
16 Videos
- SIMPLE HARMONIC MOTIONBOOK - ERRORLESS CHAPTER - SIMPLE HARMONIC MOTIONEXERCISE - simple Harmonic Motion
21 Videos
Similar Questions
A solid sphere is rotating about its diameter.Due to increase in room temperature its volume increases by 0.5% .If no external torque acts the angular speed of the sphere will increase by nearly (1)/(3)% decrease by nearly (1)/(3)% increase by nearly (1)/(2)% decrease by nearly (2)/(3)%
View SolutionSTATEMENT-1 : When a sphere rotates about a diameter, the particles on the surface of sphere have different linear speeds.
STATEMENT-2 : When a ring rotates about its own axis, the particles on the circumference of ring have different linear velocities.
STATEMENT-3 : Linear acceleration of all the particles lying on the surface of a sphere rotating uniformly about its diameter are equal.
View Solution
Knowledge Check
A solid sphere is rotating about a diameter at an angular velocity ω. If it cools so that its radius reduces to I/n of its original value, its angular velocity becomes
AωnBωn2CωnDn2ωConsider a solid sphere of radius r and mass m which has a charge q distributed uniformly over its volume. The sphere is rotated about a diameter with an angular speed ω. The magnetic moment of the sphere is
A13qωr2B15qωr2C17qωr2D19qωr2A metallic solid sphere is rotating about its diameter as axes of rotation. If the temperature is increased by 200∘C, the percentage increase in its moment of inertia is (Coefficient of linear expansion of the metal =10−5/∘C)
A0.1%B0.2%C0.3%D0.4%
A sphere made of iron is rotating about its diameter as axis α=1×10−5.∘C−1. If the temperature rises by 100∘C the percentage increase in its moment of inertia is:
View SolutionA solid sphere is rotating about an axis as shown in figure. An insect follows the dotted path on the cricumference of sphere as shown .
Match the following

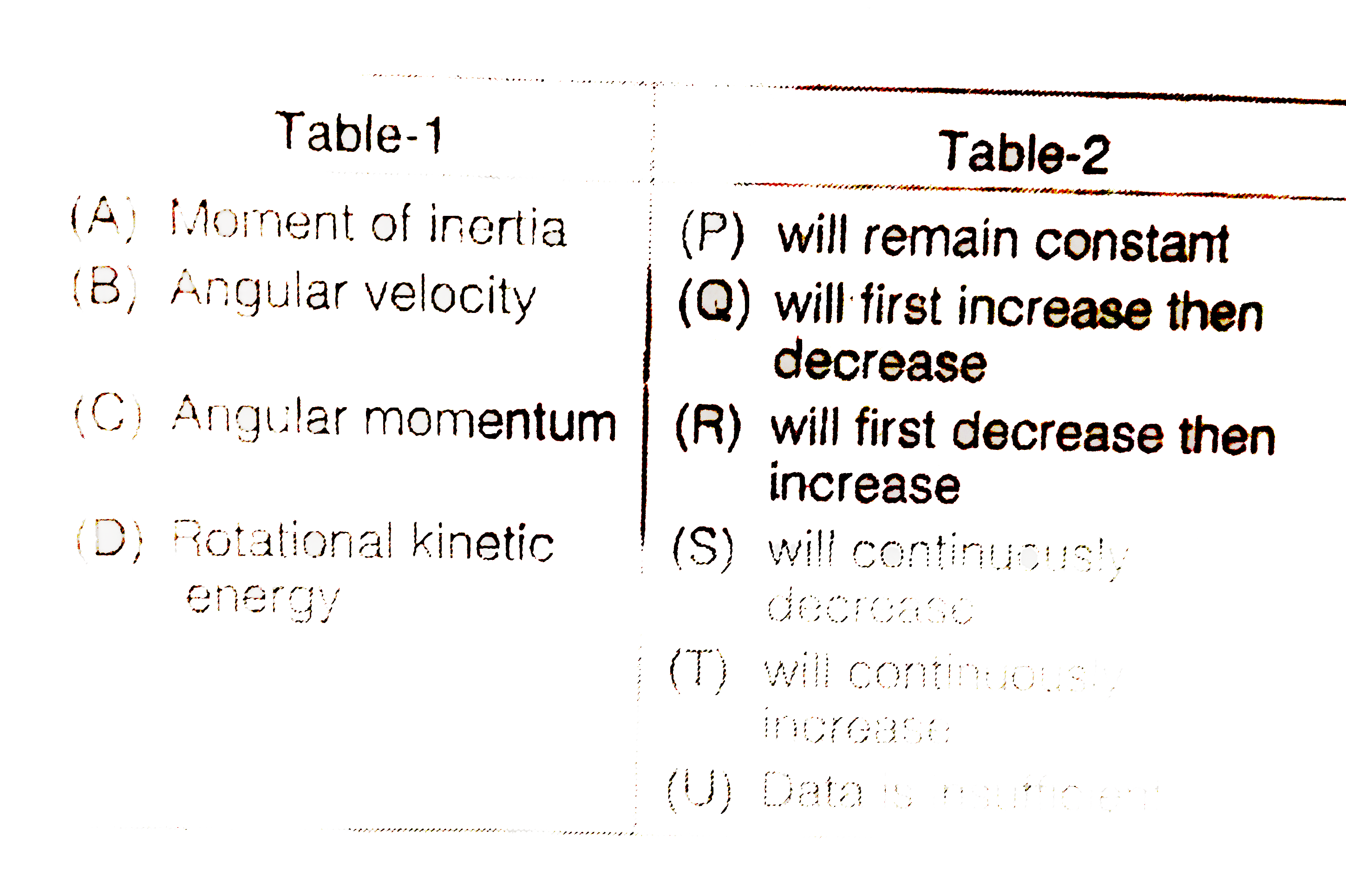
View SolutionCalculate the work that has to be done to stop an iron sphere of radius R rotating about its diameter with an angular velocity ω.
View SolutionTwo uniform spheres of mass M have radii R and 2R. Each sphere is rotating about a fixed axis through a diameter, the rotational kinetic energies of the sphere are identical. What is the ratio of the magnitude of the angular momentum of these spheres gt That is, L2RLR=
View SolutionA solid sphere of mass m and radius R is rotating about its diameter. A solid cylinder of the same mass and same radius is also rotating about its geometrical axis with an angular speed twice that of the sphere. The ratio of their kinetic energies of rotation (Esphere/Ecylinder) will be
View Solution
