A sphere of radius 1 m is kept on a plank kept on horizontal surface. Accelerations of plank and centre of sphere are indicated in the figure.

If sphere is not slipping on the plank then its angular acceleration is
8rad/s2
6rad/s2
4rad/s2
2rad/s2
The correct Answer is:A
Acceleration of centre of sphere with respect to plank is a=5+3=8m/s2.
For pure rolling we can write a=rα⇒α=8/1=8rad/s2. Hence
Topper's Solved these Questions
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS (JEE (Main) & Other State Boards for Engineering Entrance) )
48 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - COMPETITION FILE ( OBJECTIVE TYPE QUESTIONS MULTIPLE CHOICE QUESTIONS (with more than one correct answer) )
35 Videos
- SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONBOOK - MODERN PUBLICATIONCHAPTER - SYSTEMS OF PARTICLES AND ROTATIONAL MOTIONEXERCISE - REVISION EXERCISES (Numerical Problems)
20 Videos
- PHYSICAL WORLD BOOK - MODERN PUBLICATIONCHAPTER - PHYSICAL WORLD EXERCISE - Revision exercises (Long answer questions)
6 Videos
- THERMAL PROPERTIES OF MATTERBOOK - MODERN PUBLICATIONCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
In figure, a sphere of radius 2(~m) rolls on a plank. The accelerations of the sphere and the plank are indicated. Find the valuie of α (in rad(s)2 ).
View SolutionA sphere of mass m and radius R is kept on a rough horizontal floor. Constant force Facts at the top point of sphere along tangent. If there is sufficient friction on the floor to support pure rolling then calculate acceleration of sphere.
View Solution
Knowledge Check
In Fig a sphere of radius 2m rolls on a plank. The accelerations of the sphere and the plank are indicated. The value of α is
 A2rad/s2B4rad/s2C3rad/s2D1rad/s2
A2rad/s2B4rad/s2C3rad/s2D1rad/s2A hollow sphere is completely filled with a liquid having a density ρ. The radius of the sphere is R. Now the sphere is puloled with a constant horizontal acceleration of g on a horizontal surface. Take centre of sphere as origin of coordinate system as shown in the figure.

Consider points A and B as shown in the figure.APA=PBBPA>PBCPB>PADcannot sayA hollow sphere is completely filled with a liquid having a density ρ. The radius of the sphere is R. Now the sphere is puloled with a constant horizontal acceleration of g on a horizontal surface. Take centre of sphere as origin of coordinate system as shown in the figure.

Coordinate of the point having the maximum pressure isAR√2,R√2BR2,R2C−R√2,−R√2D−R2,−R2
A sphere of mass m and radius r is placed on a rough plank of mass M. The system is placed on a smooth horizontal surface. A constant force F is applied on the plank such that the sphere rolls purely on the plank. Find the acceleration of the sphere.

View SolutionA plank of mass m1 with a uniform sphere of mass m2 placed on it rests on a smooth horizontal plane. A constant horizontal force F is applied to the plank. With what acceleration will the plank and the centre of the sphere move provided there is no sliding between the plank and the sphere?
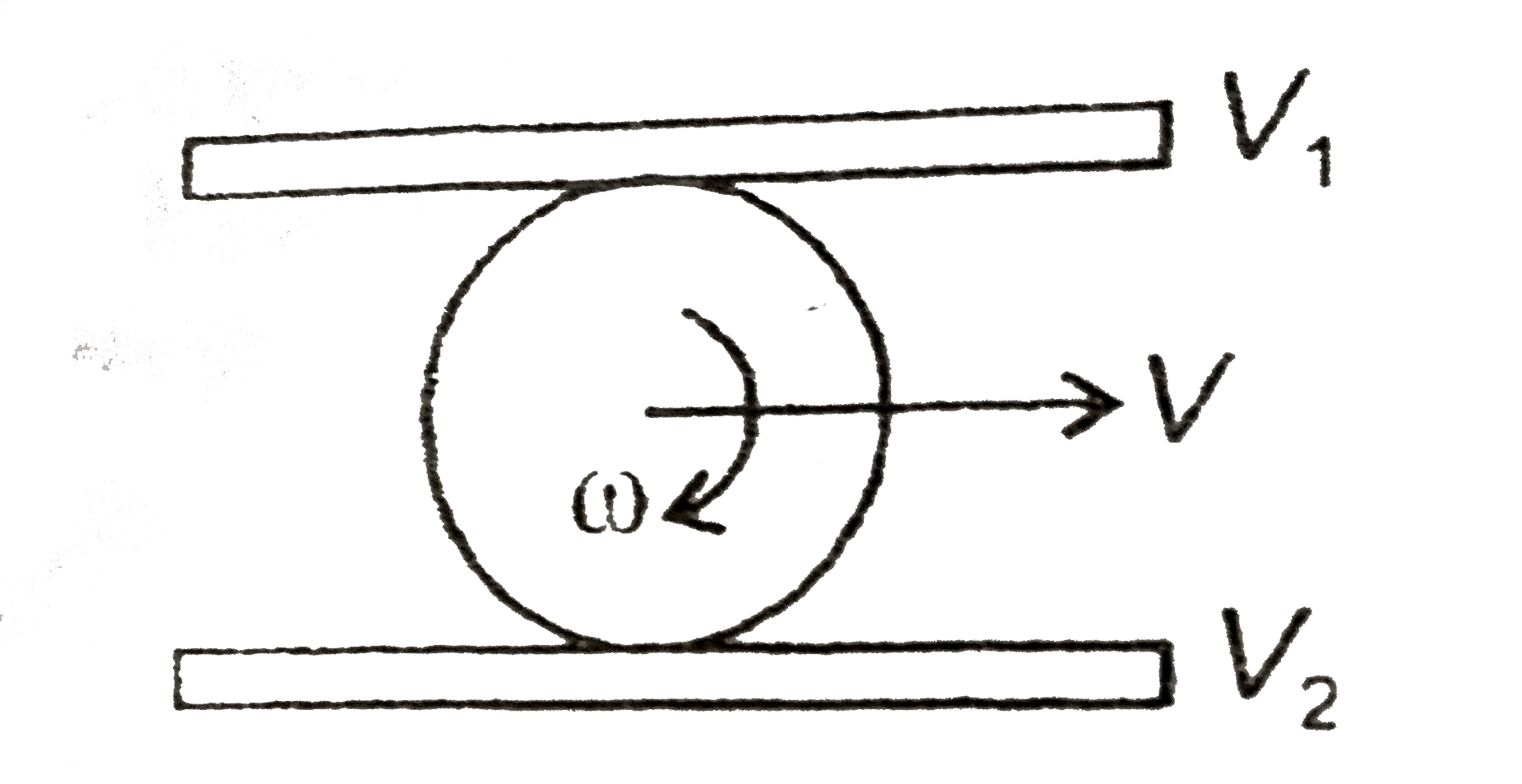
View SolutionA sphere of radius R rolls without slipping between two planks as shown in figure. Find
(a) Speed of centre of sphere
(b) Angular velocity of the sphere
View SolutionA block of mass m is kept on a rough plank which moves with a horizontal acceleration a. If the plank was at rest at t=0, and the block does not slide relative to the plank, find the work done by friction on the (a) block, (b) plane, (c) system, (block+plank) during time t.
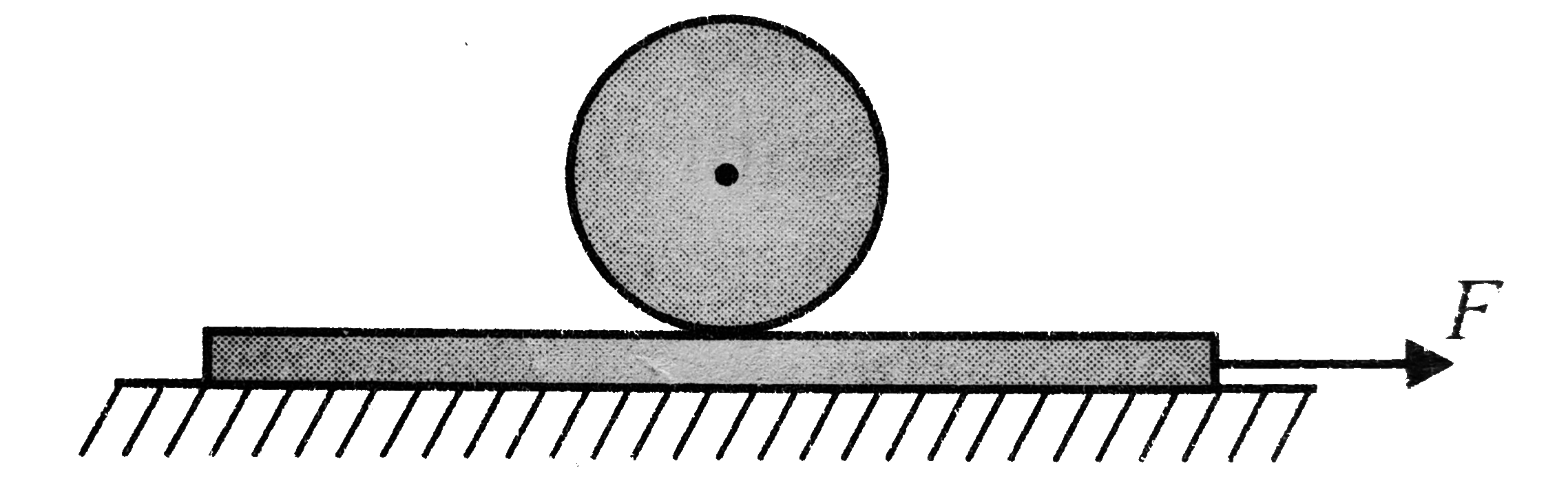
View SolutionA plank with a uniform sphere placed on it rests on a smooth horizontal plane. The plank is pulled to the right by a constant force F. It the sphere does not slip over the plank, then
View Solution
